 $\mu$-invariant of two-variable
$\mu$-invariant of two-variable  $2$-adic
$2$-adic  $\boldsymbol{L}$-functions
$\boldsymbol{L}$-functions
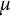 $\mu $-invariants of supersingular elliptic curves
$\mu $-invariants of supersingular elliptic curves
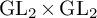 $\mathrm {GL}_2\times \mathrm {GL}_2$
$\mathrm {GL}_2\times \mathrm {GL}_2$
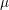 $\unicode[STIX]{x1D707}$-invariants
$\unicode[STIX]{x1D707}$-invariants
 $\unicode[STIX]{x0428}$ in towers for isogenous curves
$\unicode[STIX]{x0428}$ in towers for isogenous curves
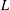 $L$-VALUES
$L$-VALUES
