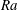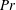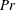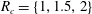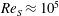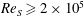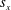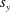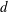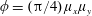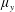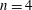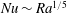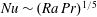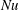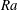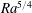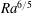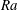Papers
On the flow and coherent structures generated by a circular array of rigid emerged cylinders placed in an open channel with flat and deformed bed
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 1-40
-
- Article
- Export citation
Direct numerical simulation of turbulence over anisotropic porous media
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 41-71
-
- Article
- Export citation
Exploring the dynamics of ‘2P’ wakes with reflective symmetry using point vortices
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 72-100
-
- Article
- Export citation
Added mass: a complex facet of tidal conversion at finite depth
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 101-127
-
- Article
- Export citation
Complete Hamiltonian formalism for inertial waves in rotating fluids
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 128-150
-
- Article
- Export citation
A neural network approach for the blind deconvolution of turbulent flows
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 151-181
-
- Article
- Export citation
Prograde, retrograde, and oscillatory modes in rotating Rayleigh–Bénard convection
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 182-211
-
- Article
- Export citation
Non-local continuum modelling of steady, dense granular heap flows
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 212-227
-
- Article
- Export citation
Boundary element methods for particles and microswimmers in a linear viscoelastic fluid
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 228-251
-
- Article
- Export citation
The instability of a moving interface in a narrow tapering channel of finite length
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 252-270
-
- Article
- Export citation
Supersonic flow fields resulting from axisymmetric internal surface curvature
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 271-288
-
- Article
- Export citation
Consistent equations for open-channel flows in the smooth turbulent regime with shearing effects
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 289-329
-
- Article
- Export citation
Velocity profiles, flow structures and scalings in a wide-gap turbulent Taylor–Couette flow
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 330-357
-
- Article
- Export citation
Spectral energy cascade in thermoacoustic shock waves
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 358-393
-
- Article
- Export citation
On the propagation of gravity currents over and through a submerged array of circular cylinders
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 394-417
-
- Article
- Export citation
Transition to turbulence in pulsating pipe flow
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 418-432
-
- Article
- Export citation
On the macroscopic modelling of dilute emulsions under flow
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 433-473
-
- Article
- Export citation
Gas depletion through single gas bubble diffusive growth and its effect on subsequent bubbles
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 474-490
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Turbulent horizontal convection under spatially periodic forcing: a regime governed by interior inertia
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 491-523
-
- Article
- Export citation
Instability wave–streak interactions in a supersonic boundary layer
-
- Published online by Cambridge University Press:
- 13 October 2017, pp. 524-553
-
- Article
- Export citation