Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-27T14:38:56.244Z Has data issue: false hasContentIssue false
  • English
  • Français

Supersonic flow fields resulting from axisymmetric internal surface curvature

Published online by Cambridge University Press:  13 October 2017

Alessandro A. Filippi  and
Beric W. Skews Open the ORCID record for Beric W. Skews [Opens in a new window]
Alessandro A. Filippi
Affiliation:
Flow Research Unit, School of Mechanical, Industrial and Aeronautical Engineering, University of the Witwatersrand, Johannesburg, 2050, South Africa
Beric W. Skews*
Affiliation:
Flow Research Unit, School of Mechanical, Industrial and Aeronautical Engineering, University of the Witwatersrand, Johannesburg, 2050, South Africa
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

An experimental and numerical study was conducted to examine the effects of internal surface curvature and leading-edge angle on the shock waves and steady flow fields produced by axisymmetric ring wedges. Test models with leading-edge-radius-normalised internal radii of curvature of 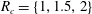$R_{c}=\{1,1.5,2\}$ and leading-edge angles of 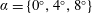$\unicode[STIX]{x1D6FC}=\{0^{\circ },4^{\circ },8^{\circ }\}$ were manufactured and tested. Experimental shadowgraph and schlieren results were obtained for Mach numbers ranging from 2.8 to 3.6 using a blowdown supersonic wind tunnel with accompanying numerical results for additional insight. The higher the internal surface curvature and leading-edge angle, the greater the flow fields were impacted. As a result, steeper compression waves were formed, thus curving the shock wave more noticeably. The internal surface curvature and leading-edge angle were both found to have an effect on the trailing-edge expansion fans. This altered the shape of downstream shock wave structures. The highest curvature models produced steady double reflection patterns due to the imposed internal surface curvature. The effects of conical and curved internal surfaces were explored for the presence of flow-normal curvature and the curving of the attached shock waves.

JFM classification

Compressible Flows: Compressible Flows Compressible Flows: Gas dynamics Compressible Flows: Shock waves
Type
Papers
Information
Journal of Fluid Mechanics , Volume 831 , 25 November 2017 , pp. 271 - 288
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ben-Dor, G. 2008 Shock Wave Reflection Phenomena, 2nd edn. Springer.Google Scholar
Ferri, A. & Nucci, l. M.1951 Preliminary investigation of a new type of supersonic inlet. Tech Rep. National Advisory Committee for Aeronautics, Washington.Google Scholar
Gounko, Y. P. 2017 Patterns of steady axisymmetric supersonic compression flows with a Mach disk. Shock Waves 27 (3), 495–506.CrossRefGoogle Scholar
Mölder, S. 1967 Internal, axisymmetric, conical flow. Am. Inst. Aeronaut. Astronaut. J. 5 (7), 1252–1255.Google Scholar
Mölder, S. 2016 Curved shock theory. Shock Waves 26 (4), 337–353.Google Scholar
Timofeev, E., Mölder, S., Voinovich, P., Takayama, K., Hosseini, S. H. R. & Takayama, K. 2001 Shock wave reflections in axisymmetric flow. In 23rd International Symposium on Shock Waves (ed. Lu, F.). University of Texas at Arlington.Google Scholar