In the following featured article, Boyer, Pouliquen & Guazzelli (J. Fluid Mech., this issue, vol. 686, 2011, pp 5–25) measure the normal stresses in a suspension of non-colloidal rigid spheres. They use the classical rod-climbing experiment, except that for interesting reasons the free surface near to the rotating rod does not rise but dips down. Careful techniques reveal that the normal stresses occur only above a volume concentration of 22 %. Over a period of hours the measurements drift, typical of many observations of suspensions. This is due to particles slowly migrating away from the rotating rod. A model of the migration gives good predictions of the observed changes.

Normal stress differences are measured in dense suspensions of neutrally buoyant non-Brownian spheres dispersed in a Newtonian fluid. Rotating-rod rheometry is used to characterize the suspension normal stresses which are responsible for a rod-dipping phenomenon. These normal stress differences are seen to strongly increase above a volume fraction of approximately 22 %. During the course of the experiments, a new time-dependent behaviour is also observed: the dip is filled with increasing times. This time evolution is found to be related to particle migration from regions of high shear rate to regions of low shear rate. The behaviour is compared with the predictions of a suspension balance model in which the particle migration flux is related to the normal stresses of the suspension.

We measure the second normal-stress difference in suspensions of non-Brownian neutrally buoyant rigid spheres dispersed in a Newtonian fluid. We use a method inspired by Wineman & Pipkin (Acta Mechanica, vol. 2, 1966, pp. 104–115) and Tanner (Trans. Soc. Rheol., vol. 14, 1970, pp. 483–507), which relies on the examination of the shape of the suspension free surface in a tilted trough flow. The second normal-stress difference is found to be negative and linear in shear stress. The ratio of the second normal-stress difference to shear stress increases with increasing volume fraction. A clear behavioural change exhibiting a strong (approximately linear) growth in the magnitude of this ratio with volume fraction is seen above a volume fraction of 0.22. By comparing our results with previous data obtained for the same batch of spheres by Boyer, Pouliquen & Guazzeli (J. Fluid Mech., 2011, doi:10.1017/jfm.2011.272), the ratio of the first normal-stress difference to the shear stress is estimated and its magnitude is found to be very small.

Reactive processes associated with multiphase flows play a significant role in mass transport in unsaturated porous media. For example, the effect of reactions on the solid matrix can affect the formation and stability of fingering instabilities associated with the invasion of a buoyant non-wetting fluid. In this study, we focus on the formation and stability of capillary channels of a buoyant non-wetting fluid (developed because of capillary instabilities) and their impact on the transport and distribution of a reactant in the porous medium. We use a combination of pore-scale numerical calculations based on a multiphase reactive lattice Boltzmann model (LBM) and scaling laws to quantify (i) the effect of dissolution on the preservation of capillary instabilities, (ii) the penetration depth of reaction beyond the dissolution/melting front, and (iii) the temporal and spatial distribution of dissolution/melting under different conditions (concentration of reactant in the non-wetting fluid, injection rate). Our results show that, even for tortuous non-wetting fluid channels, simple scaling laws assuming an axisymmetrical annular flow can explain (i) the exponential decay of reactant along capillary channels, (ii) the dependence of the penetration depth of reactant on a local Péclet number (using the non-wetting fluid velocity in the channel) and more qualitatively (iii) the importance of the melting/reaction efficiency on the stability of non-wetting fluid channels. Our numerical method allows us to study the feedbacks between the immiscible multiphase fluid flow and a dynamically evolving porous matrix (dissolution or melting) which is an essential component of reactive transport in porous media.

We consider weakly nonlinear convection in a fluid layer with a melting top boundary. This leads us to derive a new set of non-autonomous envelope equations as a dynamic generalization to the well-known Ginzburg–Landau equation. However, this new system possesses a number of interesting properties not found in systems close to a traditional dynamic bifurcation, because it involves the interaction of two destabilizing mechanisms. We investigate the system both analytically and numerically; specifically, we find the robust ‘locking in’ of spatially complex patterns, and show this is a general feature of systems of this nature.

The generalizations of the Onsager model for the radial boundary layer and the Carrier–Maslen model for the end-cap axial boundary layer in a high-speed rotating cylinder are formulated for studying the secondary gas flow due to wall heating and due to insertion of mass, momentum and energy into the cylinder. The generalizations have wider applicability than the original Onsager and Carrier–Maslen models, because they are not restricted to the limit 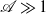, though they are restricted to the limit 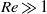and a high-aspect-ratio cylinder whose length/diameter ratio is large. Here, the stratification parameter 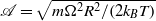. This parameter 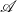is the ratio of the peripheral speed, 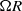, to the most probable molecular speed, 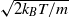, the Reynolds number 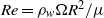, where 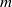is the molecular mass, 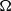and 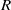are the rotational speed and radius of the cylinder, 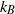is the Boltzmann constant, 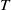is the gas temperature, 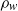is the gas density at wall, and is the gas viscosity. In the case of wall forcing, analytical solutions are obtained for the sixth-order generalized Onsager equations for the master potential, and for the fourth-order generalized Carrier–Maslen equation for the velocity potential. For the case of mass/momentum/energy insertion into the flow, the separation-of-variables procedure is used, and the appropriate homogeneous boundary conditions are specified so that the linear operators in the axial and radial directions are self-adjoint. The discrete eigenvalues and eigenfunctions of the linear operators (sixth-order and second-order in the radial and axial directions for the Onsager equation, and fourth-order and second-order in the axial and radial directions for the Carrier–Maslen equation) are determined. These solutions are compared with direct simulation Monte Carlo (DSMC) simulations. The comparison reveals that the boundary conditions in the simulations and analysis have to be matched with care. The commonly used ‘diffuse reflection’ boundary conditions at solid walls in DSMC simulations result in a non-zero slip velocity as well as a ‘temperature slip’ (gas temperature at the wall is different from wall temperature). These have to be incorporated in the analysis in order to make quantitative predictions. In the case of mass/momentum/energy sources within the flow, it is necessary to ensure that the homogeneous boundary conditions are accurately satisfied in the simulations. When these precautions are taken, there is excellent agreement between analysis and simulations, to within 10 %, even when the stratification parameter is as low as 0.707, the Reynolds number is as low as 100 and the aspect ratio (length/diameter) of the cylinder is as low as 2, and the secondary flow velocity is as high as 0.2 times the maximum base flow velocity. The predictions of the generalized models are also significantly better than those of the original Onsager and Carrier–Maslen models, which are restricted to thin boundary layers in the limit of high stratification parameter.

The gravitationally driven collapse of a reservoir into an initially stationary layer of fluid, termed the tailwater, is studied using the nonlinear shallow water equations. The motion is tackled using the hodograph transformation of the governing equation which allows the solutions for velocity and depth of the shallow flowing layer to be constructed by analytical techniques. The front of the flow emerges as a bore across which the depth of the fluid jumps discontinuously to the tailwater depth. The speed of the front is initially constant, but progressively slows once the finite extent of the reservoir begins to influence the motion. There then emerges a variety of phenomena depending upon the depth of the tailwater relative to the initial depth of the reservoir. Provided that the tailwater is sufficiently deep, a region of quiescent fluid emerges adjacent to the rear wall of the reservoir, followed by a region within which the velocity is negative. Also it is shown that for non-vanishing tailwater depths, continuous solutions for the velocity and height of the flowing layer breakdown after a sufficient period and develop an interior bore, the location and time of inception of which are calculated directly from quasi-analytical solutions.

The analytical result concerning the Markstein number of adiabatic flames was obtained in 1982 with the one-step Arrhenius model in the limit of a large activation energy. This result is not relevant for real flames. The form of the law expressing the flame velocity in terms of the total stretch rate of the flame front through a single Markstein length is not conserved when the location of the front (surface of zero thickness) changes within the flame thickness. It is shown in this paper that two different Markstein numbers characterize usual wrinkled flames sustained by a multiple-step chemical network, for the modification of the flame velocity due to the curvature of the front and for the effect of the flow strain rate. In contrast to , depends on the location of the flame surface within the flame thickness, in such a way that the final result for the flame dynamics is not depending on this choice. The first part of the paper is devoted to present a general method of solution, valid for any multiple-step chemical network. The two Markstein numbers for two-step chain-branching models representing rich hydrogen–air flames and lean hydrocarbon–air flames are then computed analytically in the second part.

At high Reynolds numbers, wake flows become more globally unstable when they are confined within a duct or between two flat plates. At Reynolds numbers around 100, however, global analyses suggest that such flows become more stable when confined, while local analyses suggest that they become more unstable. The aim of this paper is to resolve this apparent contradiction by examining a set of obstacle-free wakes. In this theoretical and numerical study, we combine global and local stability analyses of planar wake flows at to determine the effect of confinement. We find that confinement acts in three ways: it modifies the length of the recirculation zone if one exists, it brings the boundary layers closer to the shear layers, and it can make the flow more locally absolutely unstable. Depending on the flow parameters, these effects work with or against each other to destabilize or stabilize the flow. In wake flows at with free-slip boundaries, flows are most globally unstable when the outer flows are 50 % wider than the half-width of the inner flow because the first and third effects work together. In wake flows at with no-slip boundaries, confinement has little overall effect when the flows are weakly confined because the first two effects work against the third. Confinement has a strong stabilizing effect, however, when the flows are strongly confined because all three effects work together. By combining local and global analyses, we have been able to isolate these three effects and resolve the apparent contradictions in previous work.

Alongshore flow in the direction of propagation of coastal trapped waves can result in upwelling at the shelfbreak. The intensity of this upwelling can be comparable in magnitude to wind-driven coastal upwelling, with its associated ecological features. Recent numerical experiments by Matano & Palma indicate that this upwelling results from convergence of Ekman transport at the shelfbreak. The mechanism for this phenomenon can be understood in terms of steady solutions to the shallow water equations in the presence of Coriolis force and bottom drag. Matano & Palma interpreted their numerical results in terms of the arrested topographic wave, but did not present direct comparisons. Here we present a family of analytical solutions to the equations of the arrested topographic wave that shows striking quantitative agreement with earlier numerical results.

We consider the buoyancy-driven flow in an inclined porous layer which results when fluid of different temperature and composition to that in the reservoir is injected from a horizontal line well. The thermal inertia of the porous matrix leads to a transition in the temperature of the injectate as it spreads from the well and heats up to reservoir temperature. Since the buoyancy and viscosity of the injectate change across this thermal transition, the alongslope characteristic speed of the current also changes. Density and viscosity typically decrease with temperature and, so, for injectate that is positively buoyant at reservoir temperature, the changes in density and viscosity with temperature have complementary effects on the characteristic speed. In contrast, for injectate that is negatively buoyant at reservoir temperature, the changes in viscosity and density with temperature have competing influences on the characteristic speed. The change in characteristic speed, combined with the change in buoyancy across the thermal transition, leads to a series of different flow morphologies with the thermally adjusted injectate either running ahead of or lagging behind the original injectate. By approximating the thermal transition as a discrete jump, we derive the leading-order structure of these currents for the different possible cases. We then build on this to develop a more detailed boundary layer description of the thermal transition based on the theory of thin gravity driven flows in porous media. Under certain injection conditions, we show that the thermal transition is gravitationally unstable and that this may lead to mixing across the thermal transition. We consider the implications of the models for several industrial processes including geothermal heat recovery, aquifer thermal storage and carbon dioxide sequestration.

In a recent article (Nouar, Bottaro & Brancher, J. Fluid. Mech., vol. 592, 2007, pp. 177–194), a linear stability analysis of plane Poiseuille flow of shear-thinning fluids has been performed. The authors concluded that the viscosity stratification delays the transition and that is important to account for the viscosity perturbation. The current paper focuses on the first-principles understanding of the influence of the viscosity stratification and the nonlinear variation of the effective viscosity with the shear rate on the flow stability with respect to a finite-amplitude perturbation. A weakly nonlinear analysis, using the amplitude expansion method, is adopted as a first approach to study nonlinear effects. The bifurcation to two-dimensional travelling waves is studied. For the numerical computations, the shear-thinning behaviour is described by the Carreau model. The rheological parameters are varied in a wide range. The results indicate that (i) the nonlinearity of the viscous terms tends to reduce the viscous dissipation and to accelerate the flow, (ii) the harmonic generated by the nonlinearity is smaller and in opposite phase to that generated by the quadratic nonlinear inertial terms and (iii) with increasing shear-thinning effects, the bifurcation becomes highly subcritical. Consequently, the magnitude of the threshold amplitude of the perturbation, beyond which the flow is nonlinearly unstable, decreases. This result is confirmed by computing higher order-Landau constants.

To quantitatively understand the correlation between the molecular structure of an erythrocyte (red blood cell, RBC) and its mechanical response, and to predict mechanically induced structural remodelling in physiological conditions, we developed a computational model by coupling a multiscale approach of RBC membranes with a boundary element method (BEM) for surrounding Stokes flows. The membrane is depicted at three levels: in the whole cell level, a finite element method (FEM) is employed to model the lipid bilayer and the cytoskeleton as two distinct layers of continuum shells. The mechanical properties of the cytoskeleton are obtained from a molecular-detailed model of the junctional complex. The spectrin, a major protein of the cytoskeleton, is simulated using a molecular-based constitutive model. The BEM model is coupled with the FEM model through a staggered coupling algorithm. Using this technique, we first simulated RBC dynamics in capillary flow and found that the protein density variation and bilayer–skeleton interaction forces are much lower than those in micropipette aspiration, and the maximum interaction force occurs at the trailing edge. Then we investigated mechanical responses of RBCs in shear flow during tumbling, tank-treading and swinging motions. The dependencies of tank-treading frequency on the blood plasma viscosity and the membrane viscosity we found match well with benchmark data. The simulation results show that during tank-treading the protein density variation is insignificant for healthy erythrocytes, but significant for cells with a smaller bilayer–skeleton friction coefficient, which may be the case in hereditary spherocytosis.

A full Lagrangian method (FLM) is used in direct numerical simulations (DNS) of incompressible homogeneous isotropic and statistically stationary turbulent flow to measure the statistical properties of the segregation of small inertial particles advected with Stokes drag by the flow. Qualitative good agreement is observed with previous kinematic simulations (KS) (IJzermans, Meneguz & Reeks, J. Fluid Mech., vol. 653, 2010, pp. 99–136): in particular, the existence of singularities in the particle concentration field and a threshold value for the particle Stokes number above which the net compressibility of the particle concentration changes sign (from compression to dilation). A further KS analysis is carried out by examining the distribution in time of the compression of an elemental volume of particles, which shows that it is close to Gaussian as far as the third and fourth moments but non-Gaussian (within the uncertainties of the measurements) for higher-order moments when the contribution of singularities in the tails of the distribution increasingly dominates the statistics. Measurements of the rate of occurrence of singularities show that it reaches a maximum at , with the distribution of times between singularities following a Poisson process. Following the approach used by Fevrier, Simonin & Squires (J. Fluid Mech., vol. 553, 2005, pp. 1–46), we also measured the random uncorrelated motion (RUM) and mesoscopic components of the compression for and show that the non-Gaussian highly intermittent part of the distribution of the compression is associated with the RUM component and ultimately with the occurrence of singularities. This result is consistent with the formation of caustics (Wilkinson et al. Phys. Fluids, vol. 19, 2007, p. 113303), where the activation of singularities precedes the crossing of trajectories (RUM).

Direct numerical simulations (DNSs) of a zero-pressure-gradient boundary layer flow of a polymeric fluid have been performed. The FENE-P model was used for the polymer stresses and a wide range of Weissenberg numbers () was addressed. In all cases, the streamwise variations of the level of polymer stretching and the level of drag reduction are anticorrelated. Consistent with earlier studies, the inlet condition for the flow consists of Newtonian velocity data with no polymer stretching, so in the upstream region of the boundary layer the polymer molecules stretch strongly in response, leading to an initial spatial maximum in polymer stretching. Beyond this initial region, the level of drag reduction increases with increasing downstream position, while the polymer stretch is decreasing. At sufficiently high Weissenberg numbers, these variations are monotonic with streamwise position (outside the upstream region), but at , both the polymer stretching and level of drag reduction display a decaying oscillation in the downstream position. The streamwise dependence of the velocity statistics is also shown. In addition, simulations in which the polymer stress is turned off beyond a chosen downstream position were performed; in this case the flow continues to exhibit substantial drag reduction well downstream of the cutoff position. These observations are analysed in light of other recent literature and in particular the observations of ‘active’ and ‘hibernating’ turbulence recently found in minimal channel flow by Xi and Graham. All of these observations suggest that an important role for viscoelasticity in the turbulent drag reduction phenomenon, at least near solid surfaces, is to suppress conventional turbulence, while leaving behind a much weaker form of turbulence that can persist for a substantial length of time (or downstream distance) even in the absence of viscoelastic stresses.

There is a large amount of experimental and numerical work dealing with dry granular flows (such as sand, glass beads, etc.) that supports the so-called -rheology. The reliability of the -rheology in the case of complex transient flows is not fully ascertained, however. From this perspective, the granular column collapse experiment provides an interesting benchmark. In this paper we implement the -rheology in a Navier–Stokes solver (Gerris) and compare the resulting solutions with both analytical solutions and two-dimensional contact dynamics discrete simulations. In a first series of simulations, we check the numerical model in the case of a steady infinite two-dimensional granular layer avalanching on an inclined plane. A second layer of Newtonian fluid is then added over the granular layer in order to recover a close approximation of a free-surface condition. Comparisons with analytical and semi-analytical solutions provide conclusive validation of the numerical implementation of the -rheology. In a second part, we simulate the unsteady two-dimensional collapse of granular columns over a wide range of aspect ratios. Systematic comparisons with discrete two-dimensional contact dynamics simulations show good agreement between the two methods for the inner deformations and the time evolution of the shape during most of the flow, while a systematic underestimation of the final run-out is observed. The experimental scalings of spreading of the column as a function of the aspect ratio available from the literature are also recovered. A discussion follows on the performances of other rheologies, and on the sensitivity of the simulations to the parameters of the -rheology.

A fully nonlinear numerical simulation of two-dimensional Faraday waves between two incompressible and immiscible fluids is performed by adopting the phase-field method with the Cahn–Hilliard equation due to Jacqmin (J. Comput. Phys., vol. 155, 1999, pp. 96–127). Its validation is checked against the linear theory. In the nonlinear regime, qualitative comparison is made with an earlier vortex-sheet simulation of two-dimensional Faraday waves by Wright, Yon & Pozrikidis (J. Fluid Mech., vol. 400, 2000, pp. 1–32). The vorticity outside the interface region is studied in this comparison. The period tripling state, which is observed in the quasi-two-dimensional experiment by Jiang, Perlin & Schultz (J. Fluid Mech., vol. 369, 1998, pp. 273–299), is successfully simulated with the present phase-field method.

Fluid–structure interaction (FSI) simulations are carried out to investigate vortex-induced vibrations of a sphere, mounted on elastic supports in all three spatial directions. The reduced velocity () is systematically varied in the range , while the Reynolds number and reduced mass are held fixed at and , respectively. In the lock-in regime, two distinct branches are observed in the response curve, each corresponding to a distinct type of vortex shedding, namely, hairpin and spiral vortices. While shedding of hairpin vortices has been observed in several previous investigations of stationary and vibrating spheres, the shedding of intertwined, longitudinal spiral vortices in the wake of a vibrating sphere is reported herein for the first time. When the wake is in the hairpin shedding mode, the sphere moves along a linear path in the transverse plane, while when spiral vortices are shed, the sphere vibrates along a circular orbit. In the spiral mode branch, the simulations reveal hysteresis in the response amplitude at the beginning of the lock-in regime. Lower-amplitude vibrations are found as the sphere sheds hairpin vortices for increasing up until the beginning of the synchronization regime. On the other hand, higher-amplitude oscillations persist for the spiral mode as is decreased from the point of the start of the synchronization. The hairpin mode is found to be unstable for the value of reduced velocity where the spiral and hairpin solution branches merge together. When this point is approached along the hairpin solution branch, the sphere naturally transitions from shedding hairpin vortices and moving along a linear path to shedding spiral vortices and moving along a circular path in the transverse plane. The spiral mode was not observed in the work of Horowitz & Williamson (J. Fluid Mech., vol. 651, 2010, pp. 251–294), who studied experimentally the vibration modes of a freely rising or falling sphere and only reported zigzag vibrations. Our results suggest that this apparent discrepancy between experiments and simulations should be attributed to the fact that, for the range of governing parameters considered in the simulations, the elastic supports act to suppress streamwise vibrations, thus subjecting the sphere to a nearly axisymmetric elasticity constraint and enabling it to vibrate transversely along a circular path.

We carry out numerical simulations to investigate the vortex dynamics of laminar, impulsively driven flows through inclined nozzles in a piston–cylinder apparatus. Our simulations are motivated by the need to provide a complete description of the intricate vortical structures and governing mechanisms emerging in such flows as documented in the experiments of Webster & Longmire (Phys. Fluids, vol. 10, 1998, pp. 400–416) and Troolin & Longmire (Exp. Fluids, vol. 48, 2010, pp. 409–420). We show that the flow is dominated by the interaction of two main vortical structures: the primary inclined vortex ring at the nozzle exit and the secondary stopping ring that arises due to the entrainment of the flow into the cylinder when the piston stops moving. These two structures are connected together with pairs of vortex tubes, which evolve from the continuous vortex sheet initially connecting the primary vortex ring with the interior cylinder wall. In the exterior of the nozzle the key mechanism responsible for the breakup of the vortical structure is the interaction of the stronger inclined primary ring with the weaker stopping ring near the longest lip of the nozzle. In the interior of the nozzle the dynamics is governed by the axial stretching of the secondary ring and the ultimate impingement of this ring on the cylinder wall. Our simulations also clarify the kinematics of the azimuthal flow along the core of the primary vortex ring documented in the experiments by Lim (Phys. Fluids, vol. 10, 1998, pp. 1666–1671). We show that the azimuthal flow is characterized by a pair of two spiral saddle foci at the long and short lips of the nozzle through which ambient flow enters and exits the primary vortex core.

We investigate the linear stability of miscible, viscosity-layered Poiseuille flow. In the Stokes flow regime, diffusion is observed to have a destabilizing effect very similar to that of inertia in finite-Reynolds-number flows. For two-layer flows, four types of instability can dominate, depending on the interface location. Two interfacial modes exhibit large growth rates, while two additional bulk modes grow more slowly. Three-layer Stokes flows give rise to diffusive modes for each interface. These two diffusive interface modes can be in resonance, thereby enhancing the growth rate. Furthermore, modes without inertia and diffusion are also observed, consistent with a previous long-wave analysis for sharp interfaces. In contrast to that earlier investigation, the present analysis demonstrates that instability can also occur when the more viscous layer is in the centre, at larger wavenumbers.