Let 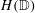 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}H(\mathbb{D})$ denote the space of holomorphic functions on the unit disc
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}H(\mathbb{D})$ denote the space of holomorphic functions on the unit disc  $\mathbb{D}$. Given
$\mathbb{D}$. Given 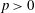 $p>0$ and a weight
$p>0$ and a weight 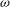 $\omega $, the Hardy growth space
$\omega $, the Hardy growth space 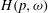 $H(p, \omega )$ consists of those
$H(p, \omega )$ consists of those 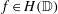 $f\in H(\mathbb{D})$ for which the integral means
$f\in H(\mathbb{D})$ for which the integral means 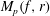 $M_p(f,r)$ are estimated by
$M_p(f,r)$ are estimated by  $C\omega (r)$,
$C\omega (r)$,  $0<r<1$. Assuming that
$0<r<1$. Assuming that 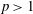 $p>1$ and
$p>1$ and 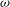 $\omega $ satisfies a doubling condition, we characterise
$\omega $ satisfies a doubling condition, we characterise 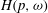 $H(p, \omega )$ in terms of associated Fourier blocks. As an application, extending a result by Bennett et al. [‘Coefficients of Bloch and Lipschitz functions’, Illinois J. Math. 25 (1981), 520–531], we compute the solid hull of
$H(p, \omega )$ in terms of associated Fourier blocks. As an application, extending a result by Bennett et al. [‘Coefficients of Bloch and Lipschitz functions’, Illinois J. Math. 25 (1981), 520–531], we compute the solid hull of 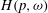 $H(p, \omega )$ for
$H(p, \omega )$ for 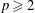 $p\ge 2$.
$p\ge 2$.
 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\gamma $-VECTORS AND THE DERIVATIVES OF THE TANGENT AND SECANT FUNCTIONS
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\gamma $-VECTORS AND THE DERIVATIVES OF THE TANGENT AND SECANT FUNCTIONS
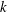 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}k$-REGULAR SEQUENCE
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}k$-REGULAR SEQUENCE
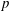 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}p$ IN FINITE
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}p$ IN FINITE 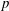 $p$-GROUPS OF COCLASS 2, WHEN
$p$-GROUPS OF COCLASS 2, WHEN 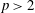 $p>2$
$p>2$
 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}^{*}$ DUNFORD–PETTIS OPERATORS ON BANACH LATTICES
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}^{*}$ DUNFORD–PETTIS OPERATORS ON BANACH LATTICES