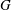Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Abdollahi, Alireza
Ahmadi, Marzieh
and
Ghoraishi, S. Mohsen
2017.
Finite p-groups with the least number of outer p-automorphisms.
Journal of Algebra and Its Applications,
Vol. 16,
Issue. 06,
p.
1750111.
Abdollahi, A.
and
Ghoraishi, S. M.
2017.
On noninner automorphisms of 2-generator finite p-groups.
Communications in Algebra,
Vol. 45,
Issue. 8,
p.
3636.
Ghoraishi, S. Mohsen
2018.
On noninner automorphisms of finite p-groups that fix the Frattini subgroup elementwise.
Journal of Algebra and Its Applications,
Vol. 17,
Issue. 07,
p.
1850137.
Lewis, Mark L.
2018.
Group Theory and Computation.
p.
141.
Garg, Rohit
and
Singh, Mandeep
2021.
Finite p-groups with non-cyclic center have a non-inner automorphism of order p.
Archiv der Mathematik,
Vol. 117,
Issue. 2,
p.
129.
Ghoraishi, S. Mohsen
2021.
Noninner automorphisms of order p for finite p-groups of restricted coclass.
Archiv der Mathematik,
Vol. 117,
Issue. 4,
p.
361.
Abdollahi, Alireza
and
Rahmani, Nafiseh
2022.
Groups of Prime Power Order Isomorphic to Their Automorphism Groups.
Bulletin of the Iranian Mathematical Society,
Vol. 48,
Issue. 5,
p.
2079.
Soleimani, Rasoul
2023.
On semicomplete finite p-groups.
Ricerche di Matematica,
Vol. 72,
Issue. 1,
p.
417.
Singh, Mandeep
and
Sharma, Mahak
2025.
Finite p-groups with cyclic center have non-inner automorphisms of order p.
Archiv der Mathematik,