In this paper, we characterise the quasi-strong endomorphisms of the join of split graphs. We give conditions under which the quasi-strong endomorphisms of the join of split graphs form a monoid.

Given a connected regular graph $G$, let $l(G)$ be its line graph, $s(G)$ its subdivision graph, $r(G)$ the graph obtained from $G$ by adding a new vertex corresponding to each edge of $G$ and joining each new vertex to the end vertices of the corresponding edge and $q(G)$ the graph obtained from $G$ by inserting a new vertex into every edge of $G$ and new edges joining the pairs of new vertices which lie on adjacent edges of $G$. A formula for the normalised Laplacian characteristic polynomial of $l(G)$ (respectively $s(G),r(G)$ and $q(G)$) in terms of the normalised Laplacian characteristic polynomial of $G$ and the number of vertices and edges of $G$ is developed and used to give a sharp lower bound for the degree-Kirchhoff index and a formula for the number of spanning trees of $l(G)$ (respectively $s(G),r(G)$ and $q(G)$).

Let $G$ be a graph of order $n\geq 6$ with minimum degree ${\it\delta}(G)\geq 4$. Arkin and Hassin [‘Graph partitions with minimum degree constraints’, Discrete Math. 190 (1998), 55–65] conjectured that there exists a bipartition $S,T$ of $V(G)$ such that $\lfloor n/2\rfloor -2\leq |S|,|T|\leq \lceil n/2\rceil +2$ and the minimum degrees in the subgraphs induced by $S$ and $T$ are at least two. In this paper, we first show that $G$ has a bipartition such that the minimum degree in each part is at least two, and then prove that the conjecture is true if the complement of $G$ contains no complete bipartite graph $K_{3,r}$, where $r=\lfloor n/2\rfloor -3$.

In this paper, we first prove that for $g\in \{3,4\}$, there are infinitely many 3-geodesic transitive but not 3-arc transitive graphs of girth $g$ with arbitrarily large diameter and valency. Then we classify the family of 3-geodesic transitive but not 3-arc transitive graphs of valency 3 and those of valency 4 and girth 4.

We exhibit the double $q$-shuffle structure for the $q$MZVs introduced by Ohno et al. [‘Cyclic $q$-MZSV sum’, J. Number Theory132 (2012), 144–155].

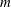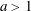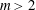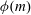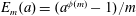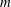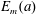Let $a$ and $m$ be relatively prime positive integers with $a>1$ and $m>2$. Let ${\it\phi}(m)$ be Euler’s totient function. The quotient $E_{m}(a)=(a^{{\it\phi}(m)}-1)/m$ is called the Euler quotient of $m$ with base $a$. By Euler’s theorem, $E_{m}(a)$ is an integer. In this paper, we consider the Diophantine equation 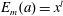$E_{m}(a)=x^{l}$ in integers 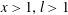$x>1,l>1$. We conjecture that this equation has exactly five solutions 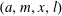$(a,m,x,l)$ except for 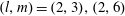$(l,m)=(2,3),(2,6)$, and show that if the equation has solutions, then 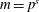$m=p^{s}$ or 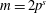$m=2p^{s}$ with 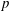$p$ an odd prime and 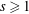$s\geq 1$.

We obtain a characterisation of the monomial ideals $I\subseteq \mathbb{C}[x_{1},\dots ,x_{n}]$ of finite colength that satisfy the condition $e(I)={\mathcal{L}}_{0}^{(1)}(I)\cdots {\mathcal{L}}_{0}^{(n)}(I)$, where ${\mathcal{L}}_{0}^{(1)}(I),\dots ,{\mathcal{L}}_{0}^{(n)}(I)$ is the sequence of mixed Łojasiewicz exponents of $I$ and $e(I)$ is the Samuel multiplicity of $I$. These are the monomial ideals whose integral closure admits a reduction generated by homogeneous polynomials.

We obtain uniqueness theorems for L-functions in the extended Selberg class when the functions share values in a finite set and share values weighted by multiplicities.

We employ a modular method to establish the new result that two types of Eisenstein series to the tredecic base may be parametrised in terms of the eta quotients ${\it\eta}^{13}({\it\tau})/{\it\eta}(13{\it\tau})$ and ${\it\eta}^{2}(13{\it\tau})/{\it\eta}^{2}({\it\tau})$. The method can also be used to give short and simple proofs for the analogous cubic, quintic and septic theories.

A semiprime ring $R$ is called a $\ast$-ring if the factor ring $R/I$ is in the prime radical for every nonzero ideal $I$ of $R$. A long-standing open question posed by Gardner asks whether the prime radical coincides with the upper radical $U(\ast _{k})$ generated by the essential cover of the class of all $\ast$-rings. This question is related to many other open questions in radical theory which makes studying properties of $U(\ast _{k})$ worthwhile. We show that $U(\ast _{k})$ is an N-radical and that it coincides with the prime radical if and only if it is complemented in the lattice $\mathbb{L}_{N}$ of all N-radicals. Along the way, we show how to establish left hereditariness and left strongness of important upper radicals and give a complete description of all the complemented elements in $\mathbb{L}_{N}$.

In this note we shall prove the existence of an uncountable subset of Liouville numbers (which we call the set of ultra-Liouville numbers) for which there exist uncountably many transcendental analytic functions mapping the subset into itself.

We study analytic properties of certain infinite products of cyclotomic polynomials that generalise some products introduced by Mahler. We characterise those that have the unit circle as a natural boundary and use associated Dirichlet series to obtain their asymptotic behaviour near roots of unity.

We study the distribution of the orbits of real numbers under the beta-transformation $T_{{\it\beta}}$ for any ${\it\beta}>1$. More precisely, for any real number ${\it\beta}>1$ and a positive function ${\it\varphi}:\mathbb{N}\rightarrow \mathbb{R}^{+}$, we determine the Lebesgue measure and the Hausdorff dimension of the following set:

$$\begin{eqnarray}E(T_{{\it\beta}},{\it\varphi})=\{(x,y)\in [0,1]\times [0,1]:|T_{{\it\beta}}^{n}x-y|<{\it\varphi}(n)\text{ for infinitely many }n\in \mathbb{N}\}.\end{eqnarray}$$

Let $f$ be a rational function on an algebraic curve over the complex numbers. For a point $p$ and local parameter $x$ we can consider the Taylor series for $f$ in the variable $x$. In this paper we give an upper bound on the frequency with which the terms in the Taylor series have $0$ as their coefficient.

A basis ${\mathcal{B}}=\{u_{i}\}_{i\in I}$ of a commutative or anticommutative algebra $\mathfrak{C},$ over an arbitrary base field $\mathbb{F}$, is called multiplicative if for any $i,j\in I$ we have that $u_{i}u_{j}\in \mathbb{F}u_{k}$ for some $k\in I$. We show that if a commutative or anticommutative algebra $\mathfrak{C}$ admits a multiplicative basis then it decomposes as the direct sum $\mathfrak{C}=\bigoplus _{j}\mathfrak{i}_{j}$ of well-described ideals each one of which admits a multiplicative basis. Also the minimality of $\mathfrak{C}$ is characterised in terms of the multiplicative basis and it is shown that, under a mild condition, the above direct sum is indexed by the family of its minimal ideals admitting a multiplicative basis.

The aim of this paper is to discuss the commutativity of a Banach algebra $A$ via its derivations. In particular, we prove that if $A$ is a unital prime Banach algebra and $A$ has a nonzero continuous linear derivation $d:A\rightarrow A$ such that either $d((xy)^{m})-x^{m}y^{m}$ or $d((xy)^{m})-y^{m}x^{m}$ is in the centre of $A$ for an integer $m=m(x,y)$ and sufficiently many $x,y$, then $A$ is commutative. We give examples to illustrate the scope of the main results and show that the hypotheses are not superfluous.

For any positive integer $n$, let $f(n)$ denote the number of 1-shell totally symmetric plane partitions of $n$. Recently, Hirschhorn and Sellers [‘Arithmetic properties of 1-shell totally symmetric plane partitions’, Bull. Aust. Math. Soc.89 (2014), 473–478] and Yao [‘New infinite families of congruences modulo 4 and 8 for 1-shell totally symmetric plane partitions’, Bull. Aust. Math. Soc.90 (2014), 37–46] proved a number of congruences satisfied by $f(n)$. In particular, Hirschhorn and Sellers proved that $f(10n+5)\equiv 0\ (\text{mod}\ 5)$. In this paper, we establish the generating function of $f(30n+25)$ and prove that $f(250n+125)\equiv 0\ (\text{mod\ 25}).$

The Wielandt subgroup of a group $G$, denoted by ${\it\omega}(G)$, is the intersection of the normalisers of all subnormal subgroups of $G$. The terms of the Wielandt series of $G$ are defined, inductively, by putting ${\it\omega}_{0}(G)=1$ and ${\it\omega}_{i+1}(G)/{\it\omega}_{i}(G)={\it\omega}(G/{\it\omega}_{i}(G))$. In this paper, we investigate the relations between the$p$-length of a $p$-soluble finite group and the Wielandt series of its Sylow $p$-subgroups. Some recent results are improved.

Let $A_{3}(n)$ denote the number of bipartitions of $n$ with 3-cores. Recently, Lin [‘Some results on bipartitions with 3-core’, J. Number Theory139 (2014), 44–52] established some congruences modulo 4, 5, 7 and 8 for $A_{3}(n)$. In this paper, we prove several infinite families of congruences modulo 3 and 9 for $A_{3}(n)$ by employing two identities due to Ramanujan.

Let $G$ be a finite group, let ${\it\pi}(G)$ be the set of prime divisors of $|G|$ and let ${\rm\Gamma}(G)$ be the prime graph of $G$. This graph has vertex set ${\it\pi}(G)$, and two vertices $r$ and $s$ are adjacent if and only if $G$ contains an element of order $rs$. Many properties of these graphs have been studied in recent years, with a particular focus on the prime graphs of finite simple groups. In this note, we determine the pairs $(G,H)$, where $G$ is simple and $H$ is a proper subgroup of $G$ such that ${\rm\Gamma}(G)={\rm\Gamma}(H)$.