Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Xia, Ernest X. W.
2015.
Arithmetic properties of bipartitions with 3-cores.
The Ramanujan Journal,
Vol. 38,
Issue. 3,
p.
529.
Wang, Liuquan
2016.
Explicit formulas for partition pairs and triples with 3-cores.
Journal of Mathematical Analysis and Applications,
Vol. 434,
Issue. 2,
p.
1053.
Chern, Shane
2016.
Formulas for partition k-tuples with t-cores.
Journal of Mathematical Analysis and Applications,
Vol. 437,
Issue. 2,
p.
841.
Saikia, Nipen
and
Boruah, Chayanika
2017.
Some congruences modulo 2 and 5 for bipartition with 5-core.
Arab Journal of Mathematical Sciences,
Vol. 23,
Issue. 2,
p.
124.
Saikia, Nipen
and
Boruah, Chayanika
2018.
New congruences for k-tuples t-core partitions.
The Journal of Analysis,
Vol. 26,
Issue. 1,
p.
27.
Zou, Qing
2018.
Arithmetic properties of bipartitions with ℓ-core.
Journal of Interdisciplinary Mathematics,
Vol. 21,
Issue. 7-8,
p.
1533.
Dasappa, Ranganatha
2021.
Some properties of k-tuple t-core partitions.
The Ramanujan Journal,
Vol. 56,
Issue. 1,
p.
369.
Wen, Xin-Qi
2022.
Arithmetic Properties of 5-Tuple Partitions with 3-Cores.
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 45,
Issue. 4,
p.
1521.
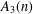 $A_{3}(n)$ denote the number of bipartitions of
$A_{3}(n)$ denote the number of bipartitions of  $n$ with 3-cores. Recently, Lin [‘Some results on bipartitions with 3-core’, J. Number Theory139 (2014), 44–52] established some congruences modulo 4, 5, 7 and 8 for
$n$ with 3-cores. Recently, Lin [‘Some results on bipartitions with 3-core’, J. Number Theory139 (2014), 44–52] established some congruences modulo 4, 5, 7 and 8 for 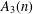 $A_{3}(n)$. In this paper, we prove several infinite families of congruences modulo 3 and 9 for
$A_{3}(n)$. In this paper, we prove several infinite families of congruences modulo 3 and 9 for 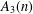 $A_{3}(n)$ by employing two identities due to Ramanujan.
$A_{3}(n)$ by employing two identities due to Ramanujan.