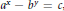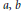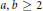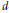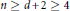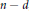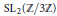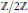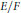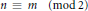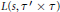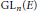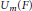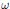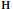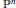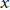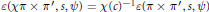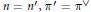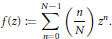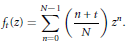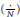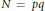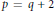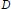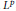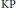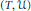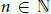Research Article
On Some Exponential Equations of S. S. Pillai
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 897-922
-
- Article
-
- You have access
- Export citation
Monotone Paths on Zonotopes and Oriented Matroids
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1121-1140
-
- Article
-
- You have access
- Export citation
Tensor Product Realizations of Simple Torsion Free Modules
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 225-243
-
- Article
-
- You have access
- Export citation
Descending Rational Points on Elliptic Curves to Smaller Fields
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 449-469
-
- Article
-
- You have access
- Export citation
The Equivariant Grothendieck Groups of the Russell-Koras Threefolds
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 3-32
-
- Article
-
- You have access
- Export citation
Jacquet Modules of Parabolically Induced Representations and Weyl Groups
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 675-695
-
- Article
-
- You have access
- Export citation
On the Tempered Spectrum of Quasi-Split Classical Groups II
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 244-277
-
- Article
-
- You have access
- Export citation
Avoiding Patterns in the Abelian Sense
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 696-714
-
- Article
-
- You have access
- Export citation
Decompositions of the Hilbert Function of a Set of Points in ℙn
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 923-943
-
- Article
-
- You have access
- Export citation
Hyperbolic Polynomials and Convex Analysis
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 470-488
-
- Article
-
- You have access
- Export citation
Sur le comportement, par torsion, des facteurs epsilon de paires
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1141-1173
-
- Article
-
- You have access
- Export citation
Merit Factors of Polynomials Formed by Jacobi Symbols
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 33-50
-
- Article
-
- You have access
- Export citation
Bivariate Polynomials of Least Deviation from Zero
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 489-505
-
- Article
-
- You have access
- Export citation
Differential Structure of Orbit Spaces
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 715-755
-
- Article
-
- You have access
- Export citation
A Generalized Variational Principle
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1174-1193
-
- Article
-
- You have access
- Export citation
A Continuous Field of Projectionless C*-Algebras
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 51-72
-
- Article
-
- You have access
- Export citation
Darboux Transformations for the KP Hierarchy in the Segal-Wilson Setting
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 278-309
-
- Article
-
- You have access
- Export citation
Représentations irréductibles bornées des groupes de Lie exponentiels
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 944-978
-
- Article
-
- You have access
- Export citation
Stratification Theory from the Weighted Point of View
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 73-97
-
- Article
-
- You have access
- Export citation
Explicit Upper Bounds for Residues of Dedekind Zeta Functions and Values of L-Functions at s = 1, and Explicit Lower Bounds for Relative Class Numbers of CM-Fields
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1194-1222
-
- Article
-
- You have access
- Export citation