No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
Soient 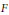 $F$ un corps commutatif localement compact non archimédien et
$F$ un corps commutatif localement compact non archimédien et  $\psi$ un caractère additif non trivial de
$\psi$ un caractère additif non trivial de 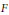 $F$. Soient
$F$. Soient 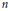 $n$ et
$n$ et 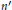 ${n}'$ deux entiers distincts, supérieurs à 1. Soient
${n}'$ deux entiers distincts, supérieurs à 1. Soient  $\pi$ et
$\pi$ et 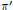 ${\pi }'$ des représentations irréductibles supercuspidales de
${\pi }'$ des représentations irréductibles supercuspidales de  $\text{G}{{\text{L}}_{n}}\left( F \right)$,
$\text{G}{{\text{L}}_{n}}\left( F \right)$, 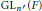 $\text{G}{{\text{L}}_{{{n}'}}}\left( F \right)$ respectivement. Nous prouvons qu’il existe un élément
$\text{G}{{\text{L}}_{{{n}'}}}\left( F \right)$ respectivement. Nous prouvons qu’il existe un élément 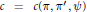 $c=c\left( \pi ,{\pi }',\psi \right)$ de
$c=c\left( \pi ,{\pi }',\psi \right)$ de 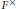 ${{F}^{\times }}$ tel que pour tout quasicaractère modéré
${{F}^{\times }}$ tel que pour tout quasicaractère modéré  $\mathcal{X}$ de
$\mathcal{X}$ de 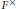 ${{F}^{\times }}$ on ait
${{F}^{\times }}$ on ait 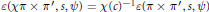 $\mathcal{E}\left( \chi \pi \times {\pi }',s,\psi \right)=\chi {{\left( c \right)}^{-1}}\mathcal{E}\left( \pi \times {\pi }',s,\psi \right)$. Nous examinons aussi certains cas où
$\mathcal{E}\left( \chi \pi \times {\pi }',s,\psi \right)=\chi {{\left( c \right)}^{-1}}\mathcal{E}\left( \pi \times {\pi }',s,\psi \right)$. Nous examinons aussi certains cas où 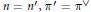 $n={n}',{\pi }'={{\pi }^{\text{v}}}$. Les résultats obtenus forment une étape vers une démonstration de la conjecture de Langlands pour
$n={n}',{\pi }'={{\pi }^{\text{v}}}$. Les résultats obtenus forment une étape vers une démonstration de la conjecture de Langlands pour 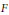 $F$, qui ne fasse pas appel à la géométrie des variétés modulaires, de Shimura ou de Drinfeld.
$F$, qui ne fasse pas appel à la géométrie des variétés modulaires, de Shimura ou de Drinfeld.
Let 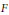 $F$ be a non-Archimedean local field, and
$F$ be a non-Archimedean local field, and  $\psi $ a non-trivial additive character of
$\psi $ a non-trivial additive character of 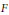 $F$. Let
$F$. Let 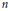 $n$ and
$n$ and 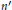 ${n}'$ be distinct positive integers. Let
${n}'$ be distinct positive integers. Let  $\pi $,
$\pi $, 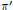 ${\pi }'$ be irreducible supercuspidal representations of
${\pi }'$ be irreducible supercuspidal representations of  $\text{G}{{\text{L}}_{n}}\left( F \right)$,
$\text{G}{{\text{L}}_{n}}\left( F \right)$, 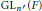 $\text{G}{{\text{L}}_{{{n}'}}}\left( F \right)$ respectively. We prove that there is
$\text{G}{{\text{L}}_{{{n}'}}}\left( F \right)$ respectively. We prove that there is 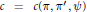 $c=c\left( \pi ,{\pi }',\psi \right)$
$c=c\left( \pi ,{\pi }',\psi \right)$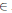 $\in $
$\in $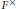 ${{F}^{\times }}$ such that for every tame quasicharacter
${{F}^{\times }}$ such that for every tame quasicharacter  $\mathcal{X}$ of
$\mathcal{X}$ of 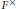 ${{F}^{\times }}$ we have
${{F}^{\times }}$ we have 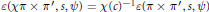 $\mathcal{E}\left( \chi \pi \times {\pi }',s,\psi \right)=\chi {{\left( c \right)}^{-1}}\mathcal{E}\left( \pi \times {\pi }',s,\psi \right)$. We also treat some cases where
$\mathcal{E}\left( \chi \pi \times {\pi }',s,\psi \right)=\chi {{\left( c \right)}^{-1}}\mathcal{E}\left( \pi \times {\pi }',s,\psi \right)$. We also treat some cases where  $n={n}'$ and
$n={n}'$ and  ${\pi }'={{\pi }^{\text{V}}}$. These results are steps towards a proof of the Langlands conjecture for
${\pi }'={{\pi }^{\text{V}}}$. These results are steps towards a proof of the Langlands conjecture for 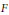 $F$, which would not use the geometry of modular—Shimura or Drinfeld—varieties.
$F$, which would not use the geometry of modular—Shimura or Drinfeld—varieties.