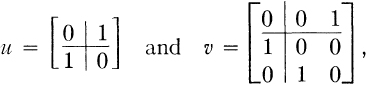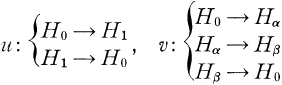Research Article
On Countability of Point-Finite Families of Sets
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 673-679
-
- Article
-
- You have access
- Export citation
Neighbor Relation and Neighbor Homomorphism of Hjelmslev Groups
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 680-699
-
- Article
-
- You have access
- Export citation
Stable Conjugacy: Definitions and Lemmas
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 700-725
-
- Article
-
- You have access
- Export citation
L-Indistinguishability For SL (2)
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 726-785
-
- Article
-
- You have access
- Export citation
A Remark on Disintegrations With Almost All Components Non
 -Additive
-Additive
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 786-788
-
- Article
-
- You have access
- Export citation
Noether Lattices Representable as Quotients of the Lattice of Monomially Generated Ideals of Polynomial Rings
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 789-799
-
- Article
-
- You have access
- Export citation
Inverse Semigroups of Homeomorphisms are Hopfian
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 800-807
-
- Article
-
- You have access
- Export citation
Lifting Isomorphisms of Modules
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 808-811
-
- Article
-
- You have access
- Export citation
Localization, Algebraic Loops and H-Spaces II
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 812-817
-
- Article
-
- You have access
- Export citation
Not Every K1-Embedded Subspace is K0-Embedded
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 818-823
-
- Article
-
- You have access
- Export citation
Baire Category And Laurent Extensions
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 824-830
-
- Article
-
- You have access
- Export citation
Simple Algebras Over Rational Function Fields
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 831-835
-
- Article
-
- You have access
- Export citation
Tensor Products of Holomorphic Discrete Series Representations
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 836-844
-
- Article
-
- You have access
- Export citation
On the Operator Identity ∑ AkXBk ≡ 0
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 845-857
-
- Article
-
- You have access
- Export citation
Sets of Uniqueness for the Group of Integers of a p-Series Field
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 858-866
-
- Article
-
- You have access
- Export citation
A Simple C*-Algebra Generated by Two Finite-Order Unitaries
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 867-880
-
- Article
-
- You have access
- Export citation
Remarks on k-Leviflat Complex Manifolds
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 881-889
-
- Article
-
- You have access
- Export citation
Strict Topology on Spaces of Continuous Vector-Valued Functions
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 890-896
-
- Article
-
- You have access
- Export citation

 . Let
. Let 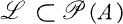 be a family of subsets of
be a family of subsets of 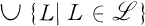 and
and 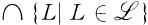 are denoted by
are denoted by  and
and 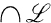 , respectively. We say that the family
, respectively. We say that the family 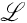 is
is 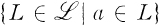 has at most finitely many members (at most one member).
has at most finitely many members (at most one member).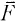 the algebraic closure of
the algebraic closure of 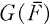 without being conjugate in
without being conjugate in 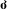 -Additive
-Additive
 ,
,  a sub σ-algebra of
a sub σ-algebra of 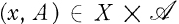 is called a
is called a 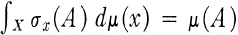
 (
(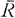 , and quotient field
, and quotient field 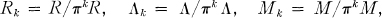
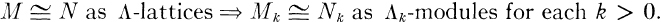
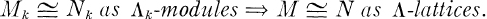
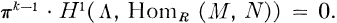
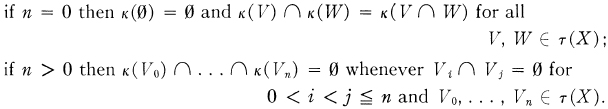
 , the algebra of bounded operators on ᚕ, defined by
, the algebra of bounded operators on ᚕ, defined by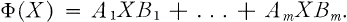

 can be represented as a sequence {
can be represented as a sequence {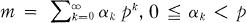 for each
for each 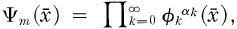
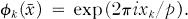
 are the Walsh-Paley functions. A detailed account of these groups and basic properties can be found in [
are the Walsh-Paley functions. A detailed account of these groups and basic properties can be found in [