State estimation is key to both analysing physical mechanisms and enabling real-time control of fluid flows. A common estimation approach is to relate sensor measurements to a reduced state governed by a reduced-order model (ROM). (When desired, the full state can be recovered via the ROM.) Current methods in this category nearly always use a linear model to relate the sensor data to the reduced state, which often leads to restrictions on sensor locations and has inherent limitations in representing the generally nonlinear relationship between the measurements and reduced state. We propose an alternative methodology whereby a neural network architecture is used to learn this nonlinear relationship. A neural network is a natural choice for this estimation problem, as a physical interpretation of the reduced state–sensor measurement relationship is rarely obvious. The proposed estimation framework is agnostic to the ROM employed, and can be incorporated into any choice of ROMs derived on a linear subspace (e.g. proper orthogonal decomposition) or a nonlinear manifold. The proposed approach is demonstrated on a two-dimensional model problem of separated flow around a flat plate, and is found to outperform common linear estimation alternatives.

This work is concerned with the oscillatory rheology of non-colloidal suspensions of highly deformable viscoelastic particles in Newtonian fluids under simple shear loading. To this end, use is made of the homogenization model of Avazmohammadi & Ponte Castañeda (J. Fluid Mech., vol. 763, 2015, pp. 386–432) accounting for the time evolution of the average particle shape and orientation. For small excitation amplitudes, the equations reduce to the small-strain Oldroyd model with expressions for the storage and loss moduli, 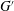$G^{\prime }$ and 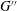$G^{\prime \prime }$, that recover those of Roscoe (J. Fluid Mech., vol. 28 (2), 1967, pp. 273–293) for small particle concentrations. However, at sufficiently large concentrations, 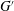$G^{\prime }$ and 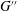$G^{\prime \prime }$ may intersect, such that 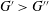$G^{\prime }>G^{\prime \prime }$ in a given frequency range. In the large amplitude oscillatory shear (LAOS) regime, the behaviour of 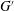$G^{\prime }$ and 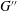$G^{\prime \prime }$ with increasing strain amplitude is consistent with type I or type III behaviour, in the terminology of Hyun et al. (J. Non-Newtonian Fluid Mech., vol. 107 (1–3), 2002, pp. 51–65), depending on the particle properties and concentration. In addition, the rheology is characterized by means of stress waveforms and Lissajous–Bowditch cycles in a Pipkin diagram. By accounting for the microstructure evolution, the model captures a number of nonlinear features commonly observed in the LAOS rheology of complex fluids, including a variety of distorted stress waveforms that manifest themselves in complex intracycle behaviour, as well as secondary loops. Furthermore, as a consequence of directional biases associated with the large deformations of the particles, the model predicts non-vanishing normal stress differences whose characteristic limit cycles are also presented in Pipkin space and reveal the formation of secondary loops for suspensions of Gent particles. However, it should be emphasized that the sources of these nonlinear rheological features for soft particle suspensions are very different from those for rigid particle colloidal systems and, in contrast to the colloidal systems, manifest themselves at dilute volume fractions of the particles.

Turbulence measurements were made in smooth-wall boundary layers subject to changing pressure gradients. Cases were documented over a range of Reynolds numbers and acceleration parameters. In all cases the boundary layer was subject to an initial zero pressure gradient (ZPG) development, followed by a favourable pressure gradient (FPG), a ZPG recovery and an adverse pressure gradient (APG). In the non-ZPG regions, the acceleration parameter, $K$, was held constant. Two component velocity profiles were acquired at multiple streamwise locations to document the response to the changing pressure gradient of the mean velocity, Reynolds stresses and triple products of the fluctuating velocity components. Velocity field measurements were made to document the turbulence structure using two point correlations. In general, turbulence was suppressed by the FPG while structures became larger in streamwise and spanwise extent relative to the boundary layer thickness, particularly near the wall. In the recovery region, the return to canonical ZPG conditions was rapid. Changes in the structure in the APG region were less pronounced. The changes in the turbulence statistics and correlations relative to the ZPG baseline were quantified and presented as functions of streamwise location. When the streamwise location is scaled using the acceleration parameter, the results from all cases (including all statistical moments, and the size and inclination angles of turbulence structures), collapse in each region of the flow, showing a common non-equilibrium response to changes in the pressure gradient. These are new results which apply to the present flows and those with similar types of pressure gradients, but are not necessarily applicable to all flows with arbitrary pressure gradients.

Direct simulations of two-dimensional plane channel flow of a viscoelastic fluid at Reynolds number $Re=3000$ reveal the existence of a family of attractors whose structure closely resembles the linear Tollmien–Schlichting (TS) mode, and in particular exhibits strongly localized stress fluctuations at the critical layer position of the TS mode. At the parameter values chosen, this solution branch is not connected to the nonlinear TS solution branch found for Newtonian flow, and thus represents a solution family that is nonlinearly self-sustained by viscoelasticity. The ratio between stress and velocity fluctuations is in quantitative agreement for the attractor and the linear TS mode, and increases strongly with Weissenberg number, $\mathit{Wi}$. For the latter, there is a transition in the scaling of this ratio as $\mathit{Wi}$ increases, and the $\mathit{Wi}$ at which the nonlinear solution family comes into existence is just above this transition. Finally, evidence indicates that this branch is connected through an unstable solution branch to two-dimensional elastoinertial turbulence (EIT). These results suggest that, in the parameter range considered here, the bypass transition leading to EIT is mediated by nonlinear amplification and self-sustenance of perturbations that excite the TS mode.

The inverse Leidenfrost regime occurs when a heated object in relative motion with a liquid is surrounded by a stable vapour layer, drastically reducing the hydrodynamic drag at large Reynolds numbers due to a delayed separation of the flow. To elucidate the physical mechanisms that control separation, here we report a numerical study of the boundary layer equations describing the liquid–vapour flow around a solid sphere whose surface temperature is above the Leidenfrost point. Our analysis reveals that the dynamics of the thin layer of vaporised liquid controls the downstream evolution of the flow, which cannot be properly described substituting the vapour layer by an effective slip length. In particular, the dominant mechanism responsible for the separation of the flow is the onset of vapour recirculation caused by the adverse pressure gradient in the rearward half of the sphere, leading to an explosive growth of the vapour-layer thickness due to the accumulation of vapour mass. Buoyancy forces are shown to have an important effect on the onset of recirculation, and thus on the separation angle. Our results compare favourably with previous experiments.

Large-scale circulations around a city are co-modulated by the urban heat island and by regional wind patterns. Depending on these variables, the circulations fall into different regimes ranging from advection-dominated (plume regime) to convection-driven (bubble regime). Using dimensional analysis and large-eddy simulations, this study investigates how these different circulations scale with urban and rural heat fluxes, as well as upstream wind speed. Two dimensionless parameters are shown to control the dynamics of the flow: (1) the ratio of rural to urban thermal convective velocities that contrasts their respective buoyancy fluxes and (2) the ratio of bulk inflow velocity to the convection velocity in the rural area. Finally, the vertical flow velocities transecting the rural to urban transitions are used to develop a criterion for categorizing different large-scale circulations into plume, bubble or transitional regimes. The findings have implications for city ventilation since bubble regimes are expected to trap pollutants, as well as for scaling analysis in canonical mixed-convection flows.

Reliable estimates of the fluxes of momentum, heat and moisture at the air–sea interface are essential for accurate long-term climate projections, as well as the prediction of short-term weather events such as tropical cyclones. In recent years, it has been suggested that these estimates need to incorporate an accurate description of the transport of sea spray within the atmospheric boundary layer and the drop-induced fluxes of momentum, heat and moisture, so that the resulting effects on atmospheric flow can be evaluated. In this paper we propose a model based on a theoretical and mathematical framework inspired from kinetic gas theory. This approach reconciles the Lagrangian nature of spray transport with the Eulerian description of the atmosphere. In turn, this enables a relatively straightforward inclusion of the spray fluxes and the resulting spray effects on the atmospheric flow. A comprehensive dimensional analysis has led us to identify the spray effects that are most likely to influence the speed, temperature and moisture of the airflow. We also provide an example application to illustrate the capabilities of the model in specific environmental conditions. Finally, suggestions for future work are offered.

With decreasing Reynolds number, $Re$, turbulence in channel flow becomes spatio-temporally intermittent and self-organises into solitary stripes oblique to the mean flow direction. We report here the existence of localised nonlinear travelling wave solutions of the Navier–Stokes equations possessing this obliqueness property. Such solutions are identified numerically using edge tracking coupled with arclength continuation. All solutions emerge in saddle-node bifurcations at values of $Re$ lower than the non-localised solutions. Relative periodic orbit solutions bifurcating from branches of travelling waves have also been computed. A complete parametric study is performed, including their stability, the investigation of their large-scale flow, and the robustness to changes of the numerical domain.

Gaseous $\text{SO}_{2}$ entrainment from a contaminated outer air stream into a pair of side-by-side homogeneous and heterogeneous micro-sized water drops is numerically examined for varied gap ratio $0.1\leqslant G/R\leqslant 6.0$ (ratio of interfacial gap to radius), Reynolds number $20\leqslant Re\leqslant 150$, Weber number $We\leqslant 1.1$, and liquid-phase Péclet number $58.33\leqslant Pe_{l}\leqslant 1055.56$. For $20<Re\leqslant 150$ and $0.1\leqslant G/R\leqslant 6.0$, the separation–attachment induced momentum exchange and imposed non-uniform interfacial shear stress lead to breakup of the primary Hill’s vortex ring and create a significant secondary vortex ring in each drop, which together construct a dominant advective $\text{SO}_{2}$ transport mechanism therein. Beneath a three-dimensional (3-D) topological separation line, the study identifies an active advective mass entrainment process that is led by the ‘inflow’ natured local dynamics of this primary–secondary vortex ring pair. Mechanistically, the secondary and primary vortex rings regulate species transfer into a drop by maintaining the spontaneous inflow-type counter-rotating motion along the 3-D separation line, whereby the $\text{SO}_{2}$ is entrained; and near the attachment points/nodes, two vortices distinctly repulse $\text{SO}_{2}$ entry by virtue of their ‘outflow’ natured local dynamics. The blockage effect and nozzle effect on flow approaching and passing the narrow neck that formed in the presence of a second drop lead to the asymmetric growth of both primary and secondary vortex rings via the locally weakened and enhanced near-interfacial air flow and imposed variable shear stress, which induce the occurrence of an asymmetric mass transfer phenomenon plus biased saturation. The $\text{SO}_{2}$, once entrained, rotates mostly along a spiral orbit of a primary vortex ring, owing to its higher strength. For increased $Re$, the $\text{SO}_{2}$ transport process is reinforced following increased strength of the inflow paired secondary–primary vortex dynamics that enhanced the net entrainment rate and also advanced its transport to the vortex core via augmented convective flow plus radial diffusion. A narrow gap facilitated faster near-gap saturation, while the quantitative $\text{SO}_{2}$ transport rate is decreased by virtue of the produced tapered primary–secondary vortex pairs, associated inner flow bifurcation, and changed topology of the separated wake, which appear similar to what develops for a larger single drop. The gap induced inner vortical structures are characterized by a weaker secondary vortex and a tapered primary vortex near the neck. For heterogeneous drop pairs, the influence of varying 3-D surface flow topology on the two interfaces and the impact of solid fraction $0.1\leqslant S\leqslant 0.8$ ($S=R_{p}/R$, with $R_{p}$ being the radius of the solid core) on the created advective mechanism by the primary–secondary vortex ring pair and resultant $\text{SO}_{2}$ transport are exclusively elucidated.

The perceived velocity gradient tensor (PVGT), constructed from four fluid tracers forming a tetrahedron, provides a natural way to study the structure of velocity fluctuations and its dependence on spatial scales. It generalizes and shares qualitatively many properties with the true velocity gradient tensor. Here, we establish the evolution equation for the PVGT, and, for homogeneous and isotropic incompressible turbulent flows, we analyse the dynamics of the PVGT in particular using its second- and third-order invariants. We show that, for PVGT based on regular tetrads with lateral size $R_{0}$, the second-order invariants can be expressed solely in terms of the usual second-order velocity structure functions, while the third-order invariants involve the usual third-order longitudinal velocity structure function and a less well known three-point velocity correlation function. For homogeneous and isotropic turbulence, exact relations between the second moments of strain and vorticity, as well as enstrophy production and the third moments of the strain, are derived. These generalized relations are valid for all ranges of $R_{0}$, and reduce to classical results for the velocity gradient tensor when $R_{0}$ is in the dissipative range. With the help of these relations, we quantify the importance of the various terms, such as vortex stretching, as a function of the scale $R_{0}$. Our analysis, which is supported by the results of direct numerical simulations of turbulent flows in the Reynolds-number range $100\leqslant R_{\unicode[STIX]{x1D706}}\leqslant 610$, allows us to demonstrate that strain prevails over vorticity when $R_{0}$ is in the inertial range.

The motive behind the present paper is to investigate a method for representing the effect of surface roughness in eddy resolving simulations of turbulent flow, without including the geometric form of the roughness as a boundary. It is found that introducing a drag force, quadratic in a reference velocity, and confined to a zone next to the wall, is remarkably possible to capture the dominant effects of roughness. The drag representation is not new; indeed, it is motivated by Reynolds averaged models. The present assessment provides a new perspective on the fluid dynamical action of distributed roughness: its dominant effect is not to create eddies in the wake of asperities, or to provide a geometric obstruction. The drag model, with no geometrical features, suppresses streaks that occur over smooth walls, and generates large, outer region eddies, in a quite similar way to resolved roughness. In a sense, this is an expanded perspective on Townsend’s hypothesis. As in that hypothesis, Reynolds stresses scale on friction velocity; but, expanding on the original hypothesis, spectra over the forcing layer agree closely with those over resolved roughness, when the force is calibrated to produce the same friction Reynolds number as the resolved roughness.

The instantaneous velocity field of turbulent flows is traditionally described in a statistical sense as a sum of an ensemble or temporal mean velocity at a specific location and a fluctuating term. While the Reynolds decomposition is well-established for fully turbulent flows, its applicability for flows that show intermittent behaviour is questionable. As intermittency is an integral part of turbulent boundary layer and turbulent jet and wake flows, an analysis of the effect of the intermittency on the statistical quantities is of fundamental interest. In this work, the effect of intermittency on single and multipoint statistics is studied for an experimentally obtained turbulent boundary layer data set at high Reynolds numbers by means of three different decomposition approaches. The analysis clearly shows that the Reynolds decomposition is only valid for single point statistics in the inner fully turbulent region of wall-bounded flows. In the wake region of a turbulent boundary layer, the Reynolds decomposition overestimates the turbulent kinetic energy due to the intermittency of the turbulent/non-turbulent interface. It is shown herein that these artefacts can be reduced or even completely avoided by applying either the boundary layer height or zonal based decomposition approach. In this study, the limitations of the different methods are analysed in detail and the approaches are evaluated comparatively by investigating statistical quantities such as turbulence intensity, anisotropy of fluctuations and characteristic length scales of coherent motions. The results make it possible to better understand and interpret the characteristics of the turbulent statistics of intermittent flows.

Here we extend the results in Gordillo et al. (J. Fluid Mech., vol. 866, 2019, pp. 298–315), where the spreading of drops impacting perpendicularly a solid wall was analysed, to predict the time-varying flow field and the thickness of the liquid film created when a spherical drop of a low viscosity fluid, like water or ethanol, spreads over a smooth dry surface at arbitrary values of the angle formed between the drop impact direction and the substrate. Our theoretical results accurately predict the time evolving asymmetric shape of the border of the thin liquid film extending over the substrate during the initial instants of the drop spreading process. In addition, the particularization of the ordinary differential equations governing the unsteady flow when the rim velocity vanishes provides an algebraic equation for the asymmetric final shapes of the liquid stains remaining after the impact, valid for low values of the inclination angle. For larger values of the inclination angle, the final shape of the drop can be approximated by an ellipse whose major and minor semiaxes can also be calculated by making use of the present theory. The predicted final shapes agree with the observed remaining stains, excluding the fact that a liquid rivulet develops from the bottom part of the drop. The limitations of the present theory to describe the emergence of the rivulet are also discussed.

The tidal waves scattered by a small island and a small cape of elliptical shape are derived by the method of matched asymptotics. The results complement the irrotational flow approximation of the near field by Proudman (Proc. Lond. Math. Soc., vol. 14, 1915, pp. 89–102). The potential for harnessing tidal power is assessed for the limiting case of a coast-connected thin dam.

We study the nonlinear mode competition of various spiral instabilities in magnetised Taylor–Couette flow. The resulting finite-amplitude mixed-mode solution branches are tracked using the annular-parallelogram periodic domain approach developed by Deguchi & Altmeyer (Phys. Rev. E, vol. 87, 2013, 043017). Mode competition phenomena are studied in both the anticyclonic and cyclonic Rayleigh-stable regimes. In the anticyclonic sub-rotation regime, with the inner cylinder rotating faster than the outer, Hollerbach et al. (Phys. Rev. Lett., vol. 104, 2010, 044502) found competing axisymmetric and non-axisymmetric magneto-rotational linearly unstable modes within the parameter range where experimental investigation is feasible. Here we confirm the existence of mode competition and compute the nonlinear mixed-mode solutions that result from it. In the cyclonic super-rotating regime, with the inner cylinder rotating slower than the outer, Deguchi (Phys. Rev. E, vol. 95, 2017, 021102) recently found a non-axisymmetric purely hydrodynamic linear instability that coexists with the non-axisymmetric magneto-rotational instability discovered a little earlier by Rüdiger et al. (Phys. Fluids, vol. 28, 2016, 014105). We show that nonlinear interactions of these instabilities give rise to rich pattern-formation phenomena leading to drastic angular momentum transport enhancement/reduction.

An experimental study is performed to determine the mechanisms by which a forward-facing step impacts the growth and breakdown to turbulence of the stationary cross-flow instability. Particle image velocimetry measurements are obtained in the boundary layer of a $30^{\circ }$ swept flat plate with a pressure body. Step heights range from 53 % to 71 % of the boundary-layer thickness. The critical step height is approximately 60 % of the boundary-layer thickness for the current study, although it is also shown that the critical step height depends on the initial amplitude of the stationary cross-flow vortices. For the critical cases, the stationary cross-flow amplitude grows sharply downstream of the step, decays for a short region and then grows again. The initial growth region is linear, and can be explained primarily through the impact of the step on the mean flow. Namely, the step causes abrupt changes to the mean flow, resulting in large values of wall-normal shear, as well as highly inflectional profiles, due to either cross-flow reversal, separation or both. These inflectional profiles are highly unstable for the stationary cross-flow. Additionally, the reversed flow regions are significantly modulated by the stationary cross-flow vortices. The second region of growth occurs due to the stationary-cross-flow-induced modulation of the shear layer, which leads to multiple smaller wavelength streamwise vortices. High-frequency fluctuations indicate that the unsteady transition mechanism for the critical cases relates to the shedding of vortices downstream of reattachment of the modulated separated regions.

The motion of a slightly rarefied gas in a long straight two-dimensional channel caused by a discontinuous surface temperature is investigated on the basis of kinetic theory with a special interest in the fluid-dynamic description. More precisely, the channel is longitudinally divided into two parts and each part is kept at a uniform temperature different from each other, so that the surface temperature of the whole channel has a jump discontinuity at the junction. Under the assumption that the amount of jump in the surface temperature is small, the steady behaviour of the gas induced in the channel is studied on the basis of the linearized Boltzmann equation and the diffuse reflection boundary condition in the case where the Knudsen number, defined by the ratio of the molecular mean free path and the width of the channel, is small. Using a matched asymptotic expansion method combined with Sone’s asymptotics, a Stokes system describing the overall macroscopic behaviour of the gas inside the channel is derived, with a new feature of the ‘slip boundary condition’ for the flow velocity due to the jump discontinuity in the surface temperature of the channel. This condition takes the form of a diverging singularity with source and sink located at the point of discontinuity, with a multiplicative factor determined through the analysis of a spatially two-dimensional Knudsen-layer (or a Knudsen-zone) problem. Some numerical demonstrations based on the Bhatnagar–Gross–Krook equation are also presented.

Researchers have hypothesized that the post-stall lift benefit of bird’s alular feathers, or alula, stems from the maintenance of an attached leading-edge vortex (LEV) over their thin-profiled, outer hand wing. Here, we investigate the connection between the alula and LEV attachment via flow measurements in a wind tunnel. We show that a model alula, whose wetted area is 1 % that of the wing, stabilizes a recirculatory aft-tilted LEV on a steadily translating unswept wing at post-stall angles of attack. The attached vortex is the result of the alula’s ability to smoothly merge otherwise separate leading- and side-edge vortical flows. We identify two key processes that facilitate this merging: (i) the steering of spanwise vorticity generated at the wing’s leading edge back to the wing plane and (ii) an aft-located wall jet of high-magnitude root-to-tip spanwise flow (${>}80\,\%$ that of the free-stream velocity). The former feature induces LEV roll-up while the latter tilts LEV vorticity aft and evacuates this flow toward the wing tip via an outboard vorticity flux. We identify the alula’s streamwise position (relative to the leading edge of the thin wing) as important for vortex steering and the alula’s cant angle as important for high-magnitude spanwise flow generation. These findings advance our understanding of the likely ways birds leverage LEVs to augment slow flight.

Numerical work on shock-wave/boundary-layer interactions (SBLI) to date has largely focused on span-periodic quasi-two-dimensional (quasi-2-D) configurations that neglect the influence lateral confinement has on the core flow. The present study is concerned with the effect of flow confinement on Mach 2 laminar SBLI in rectangular ducts. An oblique shock generated by a $2^{\circ }$ wedge forms a conical swept SBLI with sidewall boundary layers before reflecting from the bottom wall of the domain. Multiple large regions of flow-reversal are observed on the sidewalls, bottom wall and at the corner intersection. The main interaction is found to be strongly three-dimensional and highly dependent on the geometry of the duct. Comparison to quasi-2-D span-periodic simulations showed sidewalls strengthen the interaction by 31 % for the baseline configuration with an aspect ratio of one. The length of the shock generator and subsequent trailing edge expansion fan position was shown to be a critical parameter in determining the central separation length. By shortening the length of the shock generator, modification of the interaction and suppression of the central interaction is demonstrated. Parametric studies of shock strength and duct aspect ratio were performed to find limiting behaviours. For the largest aspect ratio of four, three-dimensionality was visible across 30 % of the span width away from the wall. The topology of the three-dimensional separation is shown to be similar to ‘owl-like’ separations of the first kind. Reflection of the initial conical swept SBLI is found to be the most significant factor determining the flow structures downstream of the main interaction.

Flow induced by an oscillating circular cylinder close to a plane boundary in quiescent fluid is simulated numerically by solving the two-dimensional Navier–Stokes equations. The aim of this study is to investigate the effects of the gap ratio between the cylinder and plane boundary ($G$), the oscillation direction of the cylinder ($\unicode[STIX]{x1D6FD}$) and the Keulegan–Carpenter ($KC$) number on the flow at a low Reynolds number of 150. Simulations are conducted for $G=0.1$, 0.5, 1, 1.5, 2 and 4, and $KC$ numbers between 2 and 12. Streaklines generated by releasing massless particles near the cylinder surface and contours of vorticity are used to observe the behaviour of the flow around the cylinder. The vortex shedding process from the cylinder is found to be very similar to that of a cylinder without a plane boundary except for $G=0.1$ and $\unicode[STIX]{x1D6FD}=0^{\circ }$, where vortices cannot be generated below the cylinder. Two streakline streets exist for all the flow regimes if there was not a plane boundary. A streakline street from the cylinder can be affected by the plane boundary in three ways: (1) it is suppressed by the plane boundary and stops propagating; (2) it rolls up after it meets the boundary and forms a recirculation zone; and (3) it splits into two streakline streets and forms two recirculation zones after it attacks the plane boundary. A refined classification method for flow induced by an oscillating cylinder close to a plane boundary is proposed by including a variant number, which represents the behaviour of the streaklines, into the regime names, and all the identified flow regimes are mapped on the $KC$–$G$ plane. The drag and inertia coefficients of the Morison equation are obtained using the least-squares method. A very small gap of $G=0.1$ significantly increases both the drag and inertia coefficients especially when $\unicode[STIX]{x1D6FD}=0^{\circ }$. If $G=1$ and above, the plane boundary changes the drag coefficient by less than 10 % compared with that of a cylinder without a plane boundary, and the effect of the plane boundary on the inertia coefficient is weak only when the $KC$ number is sufficiently small and vortex shedding does not exist.