Published online by Cambridge University Press: 09 June 2020
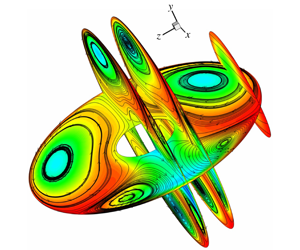
Gaseous  $\text{SO}_{2}$ entrainment from a contaminated outer air stream into a pair of side-by-side homogeneous and heterogeneous micro-sized water drops is numerically examined for varied gap ratio
$\text{SO}_{2}$ entrainment from a contaminated outer air stream into a pair of side-by-side homogeneous and heterogeneous micro-sized water drops is numerically examined for varied gap ratio  $0.1\leqslant G/R\leqslant 6.0$ (ratio of interfacial gap to radius), Reynolds number
$0.1\leqslant G/R\leqslant 6.0$ (ratio of interfacial gap to radius), Reynolds number  $20\leqslant Re\leqslant 150$, Weber number
$20\leqslant Re\leqslant 150$, Weber number  $We\leqslant 1.1$, and liquid-phase Péclet number
$We\leqslant 1.1$, and liquid-phase Péclet number  $58.33\leqslant Pe_{l}\leqslant 1055.56$. For
$58.33\leqslant Pe_{l}\leqslant 1055.56$. For  $20<Re\leqslant 150$ and
$20<Re\leqslant 150$ and  $0.1\leqslant G/R\leqslant 6.0$, the separation–attachment induced momentum exchange and imposed non-uniform interfacial shear stress lead to breakup of the primary Hill’s vortex ring and create a significant secondary vortex ring in each drop, which together construct a dominant advective
$0.1\leqslant G/R\leqslant 6.0$, the separation–attachment induced momentum exchange and imposed non-uniform interfacial shear stress lead to breakup of the primary Hill’s vortex ring and create a significant secondary vortex ring in each drop, which together construct a dominant advective  $\text{SO}_{2}$ transport mechanism therein. Beneath a three-dimensional (3-D) topological separation line, the study identifies an active advective mass entrainment process that is led by the ‘inflow’ natured local dynamics of this primary–secondary vortex ring pair. Mechanistically, the secondary and primary vortex rings regulate species transfer into a drop by maintaining the spontaneous inflow-type counter-rotating motion along the 3-D separation line, whereby the
$\text{SO}_{2}$ transport mechanism therein. Beneath a three-dimensional (3-D) topological separation line, the study identifies an active advective mass entrainment process that is led by the ‘inflow’ natured local dynamics of this primary–secondary vortex ring pair. Mechanistically, the secondary and primary vortex rings regulate species transfer into a drop by maintaining the spontaneous inflow-type counter-rotating motion along the 3-D separation line, whereby the  $\text{SO}_{2}$ is entrained; and near the attachment points/nodes, two vortices distinctly repulse
$\text{SO}_{2}$ is entrained; and near the attachment points/nodes, two vortices distinctly repulse  $\text{SO}_{2}$ entry by virtue of their ‘outflow’ natured local dynamics. The blockage effect and nozzle effect on flow approaching and passing the narrow neck that formed in the presence of a second drop lead to the asymmetric growth of both primary and secondary vortex rings via the locally weakened and enhanced near-interfacial air flow and imposed variable shear stress, which induce the occurrence of an asymmetric mass transfer phenomenon plus biased saturation. The
$\text{SO}_{2}$ entry by virtue of their ‘outflow’ natured local dynamics. The blockage effect and nozzle effect on flow approaching and passing the narrow neck that formed in the presence of a second drop lead to the asymmetric growth of both primary and secondary vortex rings via the locally weakened and enhanced near-interfacial air flow and imposed variable shear stress, which induce the occurrence of an asymmetric mass transfer phenomenon plus biased saturation. The  $\text{SO}_{2}$, once entrained, rotates mostly along a spiral orbit of a primary vortex ring, owing to its higher strength. For increased
$\text{SO}_{2}$, once entrained, rotates mostly along a spiral orbit of a primary vortex ring, owing to its higher strength. For increased  $Re$, the
$Re$, the  $\text{SO}_{2}$ transport process is reinforced following increased strength of the inflow paired secondary–primary vortex dynamics that enhanced the net entrainment rate and also advanced its transport to the vortex core via augmented convective flow plus radial diffusion. A narrow gap facilitated faster near-gap saturation, while the quantitative
$\text{SO}_{2}$ transport process is reinforced following increased strength of the inflow paired secondary–primary vortex dynamics that enhanced the net entrainment rate and also advanced its transport to the vortex core via augmented convective flow plus radial diffusion. A narrow gap facilitated faster near-gap saturation, while the quantitative  $\text{SO}_{2}$ transport rate is decreased by virtue of the produced tapered primary–secondary vortex pairs, associated inner flow bifurcation, and changed topology of the separated wake, which appear similar to what develops for a larger single drop. The gap induced inner vortical structures are characterized by a weaker secondary vortex and a tapered primary vortex near the neck. For heterogeneous drop pairs, the influence of varying 3-D surface flow topology on the two interfaces and the impact of solid fraction
$\text{SO}_{2}$ transport rate is decreased by virtue of the produced tapered primary–secondary vortex pairs, associated inner flow bifurcation, and changed topology of the separated wake, which appear similar to what develops for a larger single drop. The gap induced inner vortical structures are characterized by a weaker secondary vortex and a tapered primary vortex near the neck. For heterogeneous drop pairs, the influence of varying 3-D surface flow topology on the two interfaces and the impact of solid fraction  $0.1\leqslant S\leqslant 0.8$ (
$0.1\leqslant S\leqslant 0.8$ ( $S=R_{p}/R$, with
$S=R_{p}/R$, with  $R_{p}$ being the radius of the solid core) on the created advective mechanism by the primary–secondary vortex ring pair and resultant
$R_{p}$ being the radius of the solid core) on the created advective mechanism by the primary–secondary vortex ring pair and resultant  $\text{SO}_{2}$ transport are exclusively elucidated.
$\text{SO}_{2}$ transport are exclusively elucidated.