Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-28T21:40:27.136Z Has data issue: false hasContentIssue false
  • English
  • Français

Flow induced by an oscillating circular cylinder close to a plane boundary in quiescent fluid

Published online by Cambridge University Press:  15 June 2020

Ming Zhao Open the ORCID record for Ming Zhao [Opens in a new window]
Ming Zhao*
Affiliation:
School of Engineering, Western Sydney University, Penrith, NSW 2751, Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

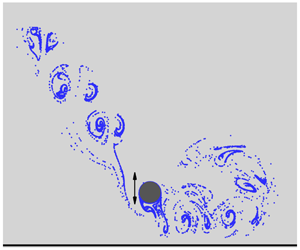
Flow induced by an oscillating circular cylinder close to a plane boundary in quiescent fluid is simulated numerically by solving the two-dimensional Navier–Stokes equations. The aim of this study is to investigate the effects of the gap ratio between the cylinder and plane boundary ($G$), the oscillation direction of the cylinder ($\unicode[STIX]{x1D6FD}$) and the Keulegan–Carpenter ($KC$) number on the flow at a low Reynolds number of 150. Simulations are conducted for $G=0.1$, 0.5, 1, 1.5, 2 and 4, and $KC$ numbers between 2 and 12. Streaklines generated by releasing massless particles near the cylinder surface and contours of vorticity are used to observe the behaviour of the flow around the cylinder. The vortex shedding process from the cylinder is found to be very similar to that of a cylinder without a plane boundary except for $G=0.1$ and $\unicode[STIX]{x1D6FD}=0^{\circ }$, where vortices cannot be generated below the cylinder. Two streakline streets exist for all the flow regimes if there was not a plane boundary. A streakline street from the cylinder can be affected by the plane boundary in three ways: (1) it is suppressed by the plane boundary and stops propagating; (2) it rolls up after it meets the boundary and forms a recirculation zone; and (3) it splits into two streakline streets and forms two recirculation zones after it attacks the plane boundary. A refined classification method for flow induced by an oscillating cylinder close to a plane boundary is proposed by including a variant number, which represents the behaviour of the streaklines, into the regime names, and all the identified flow regimes are mapped on the $KC$–$G$ plane. The drag and inertia coefficients of the Morison equation are obtained using the least-squares method. A very small gap of $G=0.1$ significantly increases both the drag and inertia coefficients especially when $\unicode[STIX]{x1D6FD}=0^{\circ }$. If $G=1$ and above, the plane boundary changes the drag coefficient by less than 10 % compared with that of a cylinder without a plane boundary, and the effect of the plane boundary on the inertia coefficient is weak only when the $KC$ number is sufficiently small and vortex shedding does not exist.

JFM classification

Vortex Flows: Vortex dynamics Vortex Flows: Vortex shedding
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 897 , 25 August 2020 , A19
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

An, H., Cheng, L. & Zhao, M. 2009 Steady streaming around a circular cylinder in an oscillatory flow. Ocean Engng 36, 1089–1097.CrossRefGoogle Scholar
An, H., Cheng, L. & Zhao, M. 2010 Steady streaming around a circular cylinder near a plane boundary due to oscillatory flow. J. Hydraul. Engng ASCE 137, 23–33.CrossRefGoogle Scholar
An, H., Cheng, L. & Zhao, M. 2011 Direct numerical simulation of oscillatory flow around a circular cylinder at low Keulegan–Carpenter number. J. Fluid Mech. 666, 77–103.CrossRefGoogle Scholar
Anagnostopoulos, P. & Minear, R. 2004 Blockage effect of oscillatory flow past a fixed cylinder. Appl. Ocean Res. 26, 147–153.CrossRefGoogle Scholar
Bearman, P. W., Downie, M. J., Graham, J. M. R. & Obasaju, E. D. 1985 Forces on cylinders in viscous oscillatory flow at low Keulegan–Carpenter numbers. J. Fluid Mech. 154, 337–356.CrossRefGoogle Scholar
Brooks, A. N. & Hughes, T. J. R. 1982 Streamline upwind/Petrov–Galerkin formulations for convection dominated flows with particular emphasis on the incompressible Navier–Stokes equations. Comput. Meth. Appl. Mech. Engng 32, 199–259.CrossRefGoogle Scholar
Carstensen, S., Sumer, B. M. & Fredsøe, J. 2010 Coherent structures in wave boundary layers. Part 1. Oscillatory motion. J. Fluid Mech. 646, 169–206.CrossRefGoogle Scholar
Dütsch, H., Durst, F., Becker, S. & Lienhart, H. 1998 Low-Reynolds-number flow around an oscillating circular cylinder at low Keulegan–Carpenter numbers. J. Fluid Mech. 360, 249–271.CrossRefGoogle Scholar
Elston, J. R., Blackburn, H. M. & Sheridan, J. 2006 The primary and secondary instabilities of flow generated by an oscillating circular cylinder. J. Fluid Mech. 550, 359–389.CrossRefGoogle Scholar
Justesen, P. 1991 A numerical study of oscillating flow around a circular cylinder. J. Fluid Mech. 222, 157–196.CrossRefGoogle Scholar
Lin, X. W., Bearman, P. W. & Graham, J. M. R. 1996 A numerical study of oscillatory flow about a circular cylinder for low values of beta parameter. J. Fluids Struct. 10, 501–526.CrossRefGoogle Scholar
Munir, A., Zhao, M., Wu, H., Ning, D. & Lu, L. 2018 Numerical investigation of the effect of plane boundary on two-degree-of-freedom of vortex-induced vibration of a circular cylinder in oscillatory flow. Ocean Engng 148, 17–32.CrossRefGoogle Scholar
Nehari, D., Armenio, V. & Ballio, F. 2004 Three-dimensional analysis of the unidirectional oscillatory flow around a circular cylinder at low Keulegan–Carpenter and 𝛽 numbers. J. Fluid Mech. 520, 157–186.CrossRefGoogle Scholar
Rahmanian, M., Cheng, L., Zhao, M. & Zhou, T. 2014 Vortex induced vibration and vortex shedding characteristics of two side-by-side circular cylinders of different diameters in close proximity in steady flow. J. Fluids Struct. 48, 260–279.CrossRefGoogle Scholar
Sarpkaya, T. 2002 Experiments on the stability of sinusoidal flow over a circular cylinder. J. Fluid Mech. 457, 157–180.CrossRefGoogle Scholar
Scandura, P., Armenio, V. & Foti, E. 2009 Numerical investigation of the oscillatory flow around a circular cylinder close to a wall at moderate Keulegan–Carpenter and low Reynolds numbers. J. Fluid Mech. 627, 259–290.CrossRefGoogle Scholar
Sumer, B. M. & Fredsøe, J. 2001 Wave scour around a large vertical circular cylinder. J. Waterways Port Coast. Ocean Engng 127, 125–134.CrossRefGoogle Scholar
Tatsuno, M. & Bearman, P. W. 1990 A visual study of the flow around an oscillating circular cylinder at low Keulegan–Carpenter numbers and low Stokes numbers. J. Fluid Mech. 211, 157–182.CrossRefGoogle Scholar
Tong, F., Cheng, L., Zhao, M. & An, H. 2015 Oscillatory flow regimes around four cylinders in a square arrangement under small KC Re conditions. J. Fluid Mech. 769, 298–336.CrossRefGoogle Scholar
Uzunoglu, B., Tan, M. & Price, W. G. 2001 Low-Reynolds-number flow around an oscillating circular cylinder using a cell viscousboundary element method. Intl J. Numer. Meth. Engng 50, 2317–2338.CrossRefGoogle Scholar
Williamson, C. H. K. 1985 Sinusoidal flow relative to circular cylinders. J. Fluid Mech. 155, 141–174.CrossRefGoogle Scholar
Wybrow, M. F. & Riley, N. 1996 Oscillatory flow over a cylinder resting on a plane boundary. Eur. J. Appl. Maths 7, 545–558.CrossRefGoogle Scholar
Wybrow, M. F., Yan, B. & Riley, N. 1996 Oscillatory flow over a circular cylinder close to a plane boundary. Fluid Dyn. Res. 18, 269–288.CrossRefGoogle Scholar
Xiong, C., Cheng, L., Tong, F. & An, H. 2018 Oscillatory flow regimes for a circular cylinder near a plane boundary. J. Fluid Mech. 844, 127–161.CrossRefGoogle Scholar
Zhao, M. & Cheng, L. 2014 Two-dimensional numerical study of vortex shedding regimes of oscillatory flow past two circular cylinders in side-by-side and tandem arrangements at low Reynolds numbers. J. Fluid Mech. 751, 1–37.CrossRefGoogle Scholar
Zhao, M., Cheng, L., Teng, B. & Dong, G. 2007 Hydrodynamic forces on dual cylinders of different diameters in steady currents. J. Fluids Struct. 23, 59–83.CrossRefGoogle Scholar
Zhao, M., Cheng, L. & Zhou, T. 2013 Numerical simulation of vortex-induced vibration of a square cylinder at a low Reynolds number. Phys. Fluids 25, 023603.Google Scholar
Zhao, M. & Yan, G. 2013 Numerical simulation of vortex-induced vibration of two circular cylinders of different diameters at low Reynolds number. Phys. Fluids 25, 083601.Google Scholar