Rotating, stratified flows are important in a wide variety of both geophysical and engineering applications. Whilst ‘steady state’ flows of this type are generally very simple (in effect, rigid body rotation), the effect of abruptly altering (even a little) the rotation rate can induce significant temporal flow disruptions, made all the more complicated when the fluid is bounded inside a closed finite container, a problem studied both experimentally and theoretically by Foster & Munro (J. Fluid Mech., this issue, vol. 712, 2012, pp. 7–40).

Here we present experimental and theoretical results for how a stratified fluid, initially rotating as a solid body with constant angular velocity, $\Omega $, within a closed cylinder of square cross-section, is spun up when subject to a small, impulsive increase, $ \mrm{\Delta} \Omega $, in the cylinder’s rotation rate. The fluid’s adjustment to the new state of solid rotation can be characterized by: (a) an inviscid, horizontal starting flow which conserves the vorticity of the initial condition; (b) the eruption of Ekman layer fluid from the perimeter region of the cylinder’s base and lid; (c) horizontal-velocity Rayleigh layers that grow into the interior from the container’s sidewalls; and (d) the formation and decay of columnar vortices in the vertical corner regions. Asymptotic results describe the inviscid starting flow, and the subsequent interior spin-up that occurs due to the combined effects of Ekman suction through the base and lid Ekman layers, and the growth of the sidewall Rayleigh layers. Attention is focused on the flow development over the spin-up time scale ${T}_{s} = {E}^{\ensuremath{-} 1/ 2} {\Omega }^{\ensuremath{-} 1} $, where $E$ is the Ekman number. (The spin-up process over the much longer diffusive time scale, ${T}_{d} = {E}^{\ensuremath{-} 1} {\Omega }^{\ensuremath{-} 1} $, is not considered here.) Experiments were performed using particle imaging velocimetry (PIV) to measure horizontal velocity components at fixed heights within the flow interior and at regular stages during the spin-up period. The velocity data obtained are shown to be in excellent agreement with the asymptotic theory.

We investigate dilute suspensions of Taylor-microscale-sized particles in homogeneous isotropic turbulence. In particular, we focus on the effect of particle shape on particle–fluid interaction. We conduct laboratory experiments using a novel experimental technique to simultaneously measure the kinematics of fluid and particle phases. This uses transparent particles having the same refractive index as water, whose motion we track via embedded optical tracers. We compare the turbulent statistics of a single-phase flow to the turbulent statistics of the fluid phase in a particle–laden suspension. Two suspensions are compared, one in which the particles are spheres and the other in which they are prolate ellipsoids with aspect ratio 2. We find that spherical particles at volume fraction ${\phi }_{v} = 0. 14\hspace{0.167em} \% $ reduce the turbulent kinetic energy (TKE) by 15 % relative to the single-phase flow. At the same volume fraction (and slightly smaller total surface area), ellipsoidal particles have a much smaller effect: they reduce the TKE by 3 % relative to the single-phase flow. Spectral analysis shows the details of TKE reduction and redistribution across spatial scales: spherical particles remove energy from large scales and reinsert it at small scales, while ellipsoids remove relatively less TKE from large scales and reinsert relatively more at small scales. Shape effects are far less evident in the statistics of particle rotation, which are very similar for ellipsoids and spheres. Comparing these with fluid enstrophy statistics, we find that particle rotation is dominated by velocity gradients on scales much larger than the particle characteristic length scales.

In this study we examine the impact of the strength of the large-scale motions on the amplitude and frequency of the small scales in high-Reynolds-number turbulent boundary layers. Time series of hot-wire data are decomposed into large- and small-scale components, and the impact of the large scale on the amplitude and frequency of the small scales is considered. The amplitude modulation effect is examined by conditionally averaging the small-scale intensity (${ u}_{S}^{2} $) for various values of the large-scale fluctuation (${u}_{L} $). It is shown that ${ u}_{S}^{2} $ increases with increasing value of ${u}_{L} $ in the near-wall region, whereas, farther away from the wall, ${ u}_{S}^{2} $ decreases with increasing ${u}_{L} $. The rate of increase in small-scale intensity with the strength of the large-scale signal is neither symmetric (about ${u}_{L} = 0$) nor linear. The extent of the frequency modulation is examined by counting the number of occurrences of local maxima or minima in the small-scale signal. It is shown that the frequency modulation effect is confined to the near-wall region and its extent diminishes rapidly beyond ${y}^{+ } = 100$. A phase lag between the large- and small-scale fluctuations, in terms of amplitude modulation, has also been identified, which is in agreement with previous studies. The phase lag between large- and small-scale fluctuations for frequency modulation is comparable to that of amplitude modulation in the near-wall region. The combined effect of both amplitude and frequency modulation is also examined by computing conditional spectra of the small-scale signal conditioned on the large scales. In the near-wall region, the results indicate that the peak value of pre-multiplied spectra increases with increasing value of ${u}_{L} $, indicating amplitude modulation, while the frequency at which this peak occurs also increases with increasing value of ${u}_{L} $, revealing frequency modulation. The overall trends observed from the conditional spectra are consistent with the results obtained through statistical analyses. Finally, a physical mechanism that can capture most of the above observations is also presented.

This paper focuses on turbulence structure in a fully developed rough-wall channel flow and its role in subgrid-scale (SGS) energy transfer. Our previous work has shown that eddies of scale comparable to the roughness elements are generated near the wall, and are lifted up rapidly by large-scale coherent structures to flood the flow field well above the roughness sublayer. Utilizing high-resolution and time-resolved particle-image-velocimetry datasets obtained in an optically index-matched facility, we decompose the turbulence into large (${\gt }\lambda $), intermediate ($3\text{{\ndash}} 6k$), roughness ($1\text{{\ndash}} 3k$) and small (${\lt }k$) scales, where $k$ and $\lambda (\lambda / k= 6. 8)$ are roughness height and wavelength, respectively. With decreasing distance from the wall, there is a marked increase in the ‘non-local’ SGS energy flux directly from large to small scales and in the fraction of turbulence dissipated by roughness-scale eddies. Conditional averaging is used to show that a small fraction of the flow volume (e.g. 5 %), which contains the most intense SGS energy transfer events, is responsible for a substantial fraction (50 %) of the energy flux from resolved to subgrid scales. In streamwise wall-normal ($x\text{{\ndash}} y$) planes, the averaged flow structure conditioned on high SGS energy flux exhibits a large inclined shear layer containing negative vorticity, bounded by an ejection below and a sweep above. Near the wall the sweep is dominant, while in the outer layer the ejection is stronger. The peaks of SGS flux and kinetic energy within the inclined layer are spatially displaced from the region of high resolved turbulent kinetic energy. Accordingly, some of the highest correlations occur between spatially displaced resolved velocity gradients and SGS stresses. In wall-parallel $x\text{{\ndash}} z$ planes, the conditional flow field exhibits two pairs of counter-rotating vortices that induce a contracting flow at the peak of SGS flux. Instantaneous realizations in the roughness sublayer show the presence of the counter-rotating vortex pairs at the intersection of two vortex trains, each containing multiple $\lambda $-spaced vortices of the same sign. In the outer layer, the SGS flux peaks within isolated vortex trains that retain the roughness signature, and the distinct pattern of two counter-rotating vortex pairs disappears. To explain the planar signatures, we propose a flow consisting of U-shaped quasi-streamwise vortices that develop as spanwise vorticity is stretched in regions of high streamwise velocity between roughness elements. Flow induced by adjacent legs of the U-shaped structures causes powerful ejections, which lift these vortices away from the wall. As a sweep is transported downstream, its interaction with the roughness generates a series of such events, leading to the formation of inclined vortex trains.

The Enskog kinetic theory is used as a starting point to model a suspension of solid particles in a viscous gas. Unlike previous efforts for similar suspensions, the gas-phase contribution to the instantaneous particle acceleration appearing in the Enskog equation is modelled using a Langevin equation, which can be applied to a wide parameter space (e.g. high Reynolds number). Attention here is limited to low Reynolds number flow, however, in order to assess the influence of the gas phase on the constitutive relations, which was assumed to be negligible in a previous analytical treatment. The Chapman–Enskog method is used to derive the constitutive relations needed for the conservation of mass, momentum and granular energy. The results indicate that the Langevin model for instantaneous gas–solid force matches the form of the previous analytical treatment, indicating the promise of this method for regions of the parameter space outside of those attainable by analytical methods (e.g. higher Reynolds number). The results also indicate that the effect of the gas phase on the constitutive relations for the solid-phase shear viscosity and Dufour coefficient is non-negligible, particularly in relatively dilute systems. Moreover, unlike their granular (no gas phase) counterparts, the shear viscosity in gas–solid systems is found to be zero in the dilute limit and the Dufour coefficient is found to be non-zero in the elastic limit.

The effects of rough surfaces on turbulent channel flow are modelled by an extra force term in the Navier–Stokes equations. This force term contains two parameters, related to the density and the height of the roughness elements, and a shape function, which regulates the influence of the force term with respect to the distance from the channel wall. This permits a more flexible specification of a rough surface than a single parameter such as the equivalent sand grain roughness. The effects of the roughness force term on turbulent channel flow have been investigated for a large number of parameter combinations and several shape functions by direct numerical simulations. It is possible to cover the full spectrum of rough flows ranging from hydraulically smooth through transitionally rough to fully rough cases. By using different parameter combinations and shape functions, it is possible to match the effects of different types of rough surfaces. Mean flow and standard turbulence statistics have been used to compare the results to recent experimental and numerical studies and a good qualitative agreement has been found. Outer scaling is preserved for the streamwise velocity for both the mean profile as well as its mean square fluctuations in all but extremely rough cases. The structure of the turbulent flow shows a trend towards more isotropic turbulent states within the roughness layer. In extremely rough cases, spanwise structures emerge near the wall and the turbulent state resembles a mixing layer. A direct comparison with the study of Ashrafian, Andersson & Manhart (Intl J. Heat Fluid Flow, vol. 25, 2004, pp. 373–383) shows a good quantitative agreement of the mean flow and Reynolds stresses everywhere except in the immediate vicinity of the rough wall. The proposed roughness force term may be of benefit as a wall model for direct and large-eddy numerical simulations in cases where the exact details of the flow over a rough wall can be neglected.

A combined experimental, numerical and theoretical investigation of the geometric scaling of the onset of a purely elastic flow instability in serpentine channels is presented. Good qualitative agreement is obtained between experiments, using dilute solutions of flexible polymers in microfluidic devices, and three-dimensional numerical simulations using the upper-convected Maxwell model. The results are confirmed by a simple theoretical analysis, based on the dimensionless criterion proposed by Pakdel & McKinley (Phys. Rev. Lett., vol. 77, 1996, pp. 2459–2462) for onset of a purely elastic flow instability. Three-dimensional simulations show that the instability is primarily driven by the curvature of the streamlines induced by the flow geometry and not due to the weak secondary flow in the azimuthal direction. In addition, the simulations also reveal that the instability is time-dependent and that the flow oscillates with a well-defined period and amplitude close to the onset of the supercritical instability.

We study spontaneous pattern formation and its asymptotic behaviour in binary fluid flow driven by a temperature gradient. When the conductive state is unstable and the size of the domain is large enough, finitely many spatially localized time-periodic travelling pulses (PTPs), each containing a certain number of convection cells, are generated spontaneously in the conductive state and are finally arranged at non-uniform intervals while moving in the same direction. We found that the role of PTP solutions and their strong interactions (collision) are important in characterizing the asymptotic state. Detailed investigations of pulse–pulse interactions showed the differences in asymptotic behaviour between that in a finite but large domain and in an infinite domain.

We use a variational formulation incorporating the full Navier–Stokes equations to identify initial perturbations with finite kinetic energy ${E}_{0} $ which generate the largest gain in perturbation kinetic energy at some time 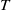$T$ later for plane Couette flow. Using the flow geometry originally used by Butler & Farrell (Phys. Fluids A, vol. 4, 1992, pp. 1637–1650) to identify the linear transient optimal perturbations for 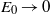${E}_{0} \ensuremath{\rightarrow} 0$ and incorporating 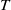$T$ as part of the optimization procedure, we show how the addition of nonlinearity smoothly changes the result as 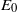${E}_{0} $ increases from zero until a small but finite 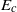${E}_{c} $ is reached. At this point, the variational algorithm is able to identify an initial condition of completely different form which triggers turbulence – called the minimal seed for turbulence. If instead 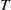$T$ is fixed at some asymptotically large value, as suggested by Pringle, Willis & Kerswell (J. Fluid Mech., vol. 703, 2012, pp. 415–443), a fundamentally different ‘final’ optimal perturbation emerges from our algorithm above some threshold initial energy 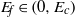${E}_{f} \in (0, {E}_{c} )$ which shows signs of localization. This nonlinear optimal perturbation clearly approaches the structure of the minimal seed as 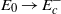${E}_{0} \ensuremath{\rightarrow} { E}_{c}^{\ensuremath{-} } $, although for 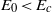${E}_{0} \lt {E}_{c} $, its maximum gain over all time intervals is always less than the equivalent maximum gain for the ‘quasi-linear optimal perturbation’, i.e. the finite-amplitude manifestation of the underlying linear optimal perturbation. We also consider a wider flow geometry recently studied by Monokrousos et al. (Phys. Rev. Lett., vol. 106, 2011, 134502) and present evidence that the critical energy for transition ${E}_{c} $ they found by using total dissipation over a time interval as the optimizing functional is recovered using energy gain at a fixed target time as the optimizing functional, with the same associated minimal seed emerging. This emphasizes that the precise form of the functional does not appear to be important for identifying 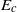${E}_{c} $ provided it takes heightened values for turbulent flows, as postulated by Pringle, Willis & Kerswell (J. Fluid Mech., vol. 703, 2012, pp. 415–443). All our results highlight the irrelevance of the linear energy gain optimal perturbation for predicting or describing the lowest-energy flow structure which triggers turbulence.

We consider the elastocapillary interaction of a liquid drop placed between two elastic beams, which are both clamped at one end to a rigid substrate. This is a simple model system relevant to the problem of surface-tension-induced collapse of flexible micro-channels that has been observed in the manufacture of microelectromechanical systems (MEMS). We determine the conditions under which the beams remain separated, touch at a point, or stick along a portion of their length. Surprisingly, we show that in many circumstances multiple equilibrium states are possible. We develop a lubrication-type model for the flow of liquid out of equilibrium and thereby investigate the stability of the multiple equilibria. We demonstrate that for given material properties two stable equilibria may exist, and show via numerical solutions of the dynamic model that it is the initial state of the system that determines which stable equilibrium is ultimately reached.

The dynamics of a perturbed incompressible, inviscid, axisymmetric, near-critical swirling flow in a long, finite-length, straight, circular pipe is studied through a weakly nonlinear analysis. The flow is subjected to non-periodic inlet and outlet conditions. The long-wave approach involves a rescaling of the axial distance and time. It results in a separation of the perturbation’s structure into a critical standing wave in the radial direction and an evolving wave in the axial direction, that is described by a nonlinear model problem. The approach is first validated by establishing the bifurcation of non-columnar states from the critical swirl and the linear stability modes of these states. Examples of the flow dynamics at various near-critical swirl levels in response to different initial perturbations demonstrate the important role of the nonlinear steepening terms in perturbation dynamics. The computed dynamics shows quantitative agreement with results from numerical simulations that are based on the axisymmetric Euler equations for various swirl levels and as long as perturbations are small, thereby verifying the accuracy of each computation and capturing the essence of flow dynamics. Results demonstrate the various stages of the flow dynamics, specifically during the transition to vortex breakdown states. They reveal the evolution of faster-than-exponential and shape-changing modes as perturbations grow into the vortex breakdown process. These explosive modes provide the sudden and abrupt nature of the vortex breakdown phenomenon. Further analysis of the model problem shows the important role of the nonlinear evolution of perturbations and its relevance to the transfer of the perturbation’s kinetic energy between the boundaries and flow bulk, the evolution of perturbations in practical concentrated vortex flows, and the design of control methods of vortex flows. A robust feedback control method to stabilize a solid-body rotation flow in a pipe at a wide range of swirl levels above critical is developed. The applicability of this method to stabilizing medium and small core-size vortices is also discussed.

Linear instabilities, both momentum-balanced and unbalanced, in several different $ \overline{u} (y)$ shear profiles are investigated in the rotating shallow water equations. The unbalanced instabilities are strongly ageostrophic and involve inertia–gravity wave motions, occurring only for finite Rossby ($\mathit{Ro}$) and Froude ($\mathit{Fr}$) numbers. They serve as a possible route for the breakdown of balance in a rotating shallow water system, which leads the energy to cascade towards small scales. Unlike previous work, this paper focuses on general shear flows with non-uniform potential vorticity, and without side or equatorial boundaries or vanishing layer depth (frontal outcropping). As well as classical shear instability among balanced shear wave modes (i.e. B–B type), two types of ageostrophic instability (B–G and G–G) are found. The B–G instability has attributes of both a balanced shear wave mode and an inertia–gravity wave mode. The G–G instability occurs as a sharp resonance between two inertia–gravity wave modes. The criterion for the occurrence of the ageostrophic instability is associated with the second stability condition of Ripa (1983), which requires a sufficiently large local Froude number. When $\mathit{Ro}$ and especially $\mathit{Fr}$ increase, the balanced instability is suppressed, while the ageostrophic instabilities are enhanced. The profile of the mean flow also affects the strength of the balanced and ageostrophic instabilities.

In the present study, shock tube experiments are used to study the very late-time development of the Richtmyer–Meshkov instability from a diffuse, nearly sinusoidal, initial perturbation into a fully turbulent flow. The interface is generated by two opposing gas flows and a perturbation is formed on the interface by transversely oscillating the shock tube to create a standing wave. The puncturing of a diaphragm generates a Mach $1. 2$ shock wave that then impacts a density gradient composed of air and SF6, causing the Richtmyer–Meshkov instability to develop in the 2.0 m long test section. The instability is visualized with planar Mie scattering in which smoke particles in the air are illuminated by a Nd:YLF laser sheet, and images are recorded using four high-speed video cameras operating at 6 kHz that allow the recording of the time history of the instability. In addition, particle image velocimetry (PIV) is implemented using a double-pulsed Nd:YAG laser with images recorded using a single CCD camera. Initial modal content, amplitude, and growth rates are reported from the Mie scattering experiments while vorticity and circulation measurements are made using PIV. Amplitude measurements show good early-time agreement but relatively poor late-time agreement with existing nonlinear models. The model of Goncharov (Phys. Rev. Lett., vol. 88, 2002, 134502) agrees with growth rate measurements at intermediate times but fails at late experimental times. Measured background acceleration present in the experiment suggests that the late-time growth rate may be influenced by Rayleigh–Taylor instability induced by the interfacial acceleration. Numerical simulations conducted using the LLNL codes Ares and Miranda show that this acceleration may be caused by the growth of boundary layers, and must be accounted for to produce good agreement with models and simulations. Adding acceleration to the Richtmyer–Meshkov buoyancy–drag model produces improved agreement. It is found that the growth rate and amplitude trends are also modelled well by the Likhachev–Jacobs vortex model (Likhachev & Jacobs, Phys. Fluids, vol. 17, 2005, 031704). Circulation measurements also show good agreement with the circulation value extracted by fitting the vortex model to the experimental data.

Turbidity currents derive their motion from the excess density imposed by suspended sediments. The settling tendency of sediments is countered by flow turbulence, which expends energy to keep them in suspension. This interaction leads to downward increasing concentration of suspended sediments (stable stratification) in the flow. Thus in a turbidity current sediments play the dual role of sustaining turbulence by driving the flow and damping turbulence due to stable stratification. By means of direct numerical simulations, it has been shown previously that stratification above a threshold can substantially reduce turbulence and possibly extinguish it. This study expands the simplified model by Cantero et al. (J. Geophys. Res., vol. 114, 2009a, C03008), and puts forth a proposition that explains the mechanism of complete turbulence suppression due to suspended sediments. In our simulations it is observed that suspensions of larger sediments lead to stronger stratification and, above a threshold size, induce an abrupt transition in the flow to complete turbulence suppression. It has been widely accepted that hairpin and quasi-streamwise vortices are key to sustaining turbulence in wall-bounded flows, and that only vortices of sufficiently strong intensity can spawn the next generation of vortices. This auto-generation mechanism keeps the flow populated with hairpin and quasi-streamwise vortical structures and thus sustains turbulence. From statistical analysis of Reynolds stress events and visualization of flow structures, it is observed that settling sediments damp the Reynolds stress events (Q2 events), which means a reduction in both the strength and spatial distribution of vortical structures. Beyond the threshold sediment size, the existing vortical structures in the flow are damped to an extent where they lose their ability to regenerate the subsequent generation of turbulent vortical structures, which ultimately leads to complete turbulence suppression.

Direct numerical simulations of free convection over a smooth, heated plate are used to investigate unbounded, unsteady turbulent convection. Four different boundary conditions are considered: free-slip or no-slip walls, and constant buoyancy or constant buoyancy flux. It is first shown that, after the initial transient, the vertical structure agrees with observations in the atmospheric boundary layer and predictions from classical similarity theory. A quasi-steady inner layer and a self-preserving outer layer are clearly distinguished, with an overlap region between them of constant turbulent buoyancy flux. The extension of the overlap region reached in our simulations is more than 100 wall units $ \mathop{ ({\kappa }^{3} / {B}_{s} )}\nolimits ^{1/ 4} $, where ${B}_{s} $ is the surface buoyancy flux and $\kappa $ the corresponding molecular diffusivity (the Prandtl number is one). The buoyancy fluctuation inside the overlap region already exhibits the $\ensuremath{-} 1/ 3$ power-law scaling with height for the four types of boundary conditions, as expected in the local, free-convection regime. However, the mean buoyancy gradient and the vertical velocity fluctuation are still evolving toward the corresponding power laws predicted by the similarity theory. The second major result is that the relation between the Nusselt and Rayleigh numbers agrees with that reported in Rayleigh–Bénard convection when the heated plate is interpreted as half a convection cell. The range of Rayleigh numbers covered in the simulations is then $5\ensuremath{\times} 1{0}^{7} \text{{\ndash}} 1{0}^{9} $. Further analogies between the two problems indicate that knowledge can be transferred between steady Rayleigh–Bénard and unsteady convection. Last, we find that the inner scaling based on $\{ {B}_{s} , \hspace{0.167em} \kappa \} $ reduces the effect of the boundary conditions to, mainly, the diffusive wall layer, the first 10 wall units. There, near the plate, free-slip conditions allow stronger mixing than no-slip ones, which results in 30 % less buoyancy difference between the surface and the overlap region and 30–40 % thinner diffusive sublayers. However, this local effect also entails one global, substantial effect: with an imposed buoyancy, free-slip systems develop a surface flux 60 % higher than that obtained with no-slip walls, which implies more intense turbulent fluctuations across the whole boundary layer and a faster growth.

We consider linear waves propagating over periodic topographies of arbitrary amplitude and wave form, generalizing the method in Howard & Yu (J. Fluid Mech., vol. 593, 2007, pp. 209–234). By a judicious construction of a conformal map from the flow domain to a uniform strip, exact solutions of Floquet type can be developed in the mapped plane. These Floquet solutions, in an essentially analytical form, are analogous to the complete set of flat-bottom propagating and evanescent waves. Therefore they can be used as a basis for the solutions of boundary value problems involving a wavy topography with a constant mean water depth. Various concrete examples are given and quantitative results are discussed. Comparisons with experimental data are made, and qualitative agreement is achieved.

Surface pressure fluctuations induced by turbulent boundary-layer flow at ${\mathit{Re}}_{\theta } = 4755$ over small backward- and forward-facing steps are studied with large-eddy simulation. Four step heights that are 53, 13, 3.3 and 0.83 % of the boundary-layer thickness are considered to investigate the effects of step height on surface pressure characteristics and pressure-source mechanisms. The extent to which turbulent velocity fluctuations in the boundary layer and the separated shear layer contribute to the surface pressure fluctuations is examined with scaling of various pressure statistics and two-point correlations. For larger steps, vortical structures develop in the shear layer and the associated intense velocity fluctuations are the dominant source. Downstream of slightly less than one reattachment length from the step, the root-mean-square pressure is found to scale with the local maximum cross-stream Reynolds normal stress ${ \overline{{v}^{\ensuremath{\prime} \hspace{0.167em} 2} } }_{\mathit{max}} $. The pressure frequency spectrum at the maximum ${p}_{\mathit{rms}} $ location consists of an energy-containing range that scales with the mean reattachment length ${x}_{r} $ and a higher frequency range that rolls off with a slope close to $\ensuremath{-} 7/ 3$. As the step height decreases, the boundary-layer turbulent fluctuations become the dominant source, the ${ \overline{{v}^{\ensuremath{\prime} \hspace{0.167em} 2} } }_{\mathit{max}} $ scaling of ${p}_{\mathit{rms}} $ is no longer valid and the roll-off slope of the frequency spectrum becomes steeper. The downstream recovery of a step-perturbed boundary layer towards an equilibrium boundary layer is investigated from the point of view of surface pressure fluctuations. For steps with a strong separated shear layer, pressure fluctuations are found to decay rapidly for up to three reattachment lengths downstream of the step, within which approximately 60 % of the peak ${p}_{\mathit{rms}} $ is dissipated. Farther downstream, recovery is much slower. The pressure-recovery distances estimated for the largest backward and forward steps are 175 and 295 step heights, respectively.

Hydrodynamic interactions among multiple circular cylinders translating in an otherwise undisturbed inviscid fluid are theoretically investigated. A constructive method for solving a Neumann boundary-value problem in a domain outside $N$ circles (one kind of Hilbert boundary-value problem in the complex plane) is presented in the study to derive the velocity potential of the liquid. The method employs successive offset functions combined with a ‘generalized cyclic permutation’ in turn to satisfy the impenetrable boundary condition on each circle. The complex potential is therefore expressed as $N$ isolated singularities in power series form and used to get instantaneous added masses of $N$ submerged circular cylinders. Then, based on the Hamilton variational principle, a dynamical equation of motion in vector form is derived to predict nonlinear translations of the submerged bodies under fully hydrodynamic interactions. Also, the equivalence of the energy-based Lagrangian framework and a momentum-type one in the two-dimensional body–liquid system is proved. It implies that the pressure integration around a submerged body is holographic, which provides information about velocities and accelerations of all bodies. The numerical solutions indicate some typical dynamical behaviours of more than two circular cylinders which reveal that interesting nonlinear phenomena would appear in such a system with simple physical assumptions.