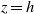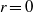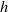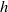The wall pressure and wall shear stress of a submerged viscous jet impinging on an infinite planar wall are derived. The whole creeping flow of semi-infinite extent is generated via distributions on a cylindrical pipe of tangentially and normally directed Stokeslets which are modified to achieve no-slip at the wall in two stages. First the pressure and vorticity jumps associated with the Poiseuille flow upstream in the pipe are readily forced, and then further distributions, of zero density far upstream but with square-root density singularity at the orifice $z= h$, are added to achieve no-slip on the pipe wall. Thus the adjustment of the interior pipe flow from its upstream parabolic profile to its exit profile is fully included in – and a major feature of – this creeping flow analysis. The maximum plane wall pressure is always located on the axis $r= 0$, and decreases as $h$ increases to alleviate the obstruction effect of the wall. The interaction of the inflow with the ambient fluid in the neighbourhood of $z= 0$ causes the wall stress to rise rapidly to a maximum and then decay with the radial position of this maximum increasing as $h$ increases. This behaviour is discussed in the context of physiological experiments on auditory sensory hair cells that motivated this study.

Ice-stream shear margins are the lateral boundaries of narrow, fast-flowing bands of ice within an ice sheet. We develop a theory for the migration of shear margins over time driven by viscous dissipation of heat within the ice, focusing on widening of the ice stream. The location of the margin is modelled as a transition from a cold to a temperate ice-sheet bed, and simultaneously as the transition from no slip to free slip at the same location. The temperature field in the ice is affected by intense shear heating as well as by the migration velocity of the margin (i.e. by the widening rate of the ice stream); if migration is too fast, there is little time for the ice to warm up and the margin remains cold, causing the bed to freeze. This suppresses widening. Conversely, if the migration speed is too slow, the ice in the margin warms up, causing the bed on the far side of the cold–temperate transition to reach the melting point, and migration to speed up. Using a Wiener–Hopf method, we show that for a given far-field shear stress, geothermal heat flux, and ice geometry, there is a single migration velocity that balances the two effects and permits widening at a steady rate. This velocity increases with the far-field lateral shear stress imposed by the ice stream, which controls shear heating in the margin. Our results also indicate that (i) a region of temperate ice must form in the margin, and that (ii) lateral advection of ice may play a significant role in controlling migration speeds.

The pressure-driven miscible displacement of a less viscous fluid by a more viscous one in a horizontal channel is studied. This is a classically stable system if the more viscous solution is the displacing one. However, we show by numerical simulations based on the finite-volume approach that, in this system, double diffusive effects can be destabilizing. Such effects can appear if the fluid consists of a solvent containing two solutes both influencing the viscosity of the solution and diffusing at different rates. The continuity and Navier–Stokes equations coupled to two convection–diffusion equations for the evolution of the solute concentrations are solved. The viscosity is assumed to depend on the concentrations of both solutes, while density contrast is neglected. The results demonstrate the development of various instability patterns of the miscible ‘interface’ separating the fluids provided the two solutes diffuse at different rates. The intensity of the instability increases when increasing the diffusivity ratio between the faster-diffusing and the slower-diffusing solutes. This brings about fluid mixing and accelerates the displacement of the fluid originally filling the channel. The effects of varying dimensionless parameters, such as the Reynolds number and Schmidt number, on the development of the ‘interfacial’ instability pattern are also studied. The double diffusive instability appears after the moment when the invading fluid penetrates inside the channel. This is attributed to the presence of inertia in the problem.

The force and power of flapping plates are studied by vortex dynamic analysis. Based on the dynamic analysis of the numerical results of viscous flow past three-dimensional flapping plates, it is found that the force and power are strongly dominated by the vortical structures close to the body. Further, the dynamics of the flapping plate is investigated in terms of viscous vortex-ring model. It is revealed that the model can reasonably reflect the essential properties of the ring-like vortical structure in the wake, and the energy of the plate transferred to the flow for the formation of each vortical structure possesses a certain relation. Moreover, simplified formulae for the thrust and efficiency are proposed and verified to be reliable by the numerical solutions and experimental measurements of animal locomotion. The results obtained in this study provide physical insight into the understanding of the dynamic mechanisms relevant to flapping locomotion.

This experimental study, performed under microgravity conditions, focuses on the evaporation dynamics of ethanol drops and the formation and behaviour of the hydrothermal waves that spontaneously develop on the drop surfaces. The aim of this study is to compare our results to a similar study performed under normal gravity conditions to confirm the purely thermocapillary origin of these instabilities. A scaling law predicts with good agreement the number of instabilities that form, regardless of the gravity level.

We present a study of bidispersed particulate gravity currents at high Reynolds numbers flowing along a V-shaped valley. The speed and width of the currents, the mass deposited by the currents and the density of the deposits were examined by both a box model and lock-exchange experiments in a 5 m long tank. Silicon carbide and glass beads were used for the bidispersed suspension models. The initial conditions of the currents were similar, except that the grain size of the glass beads was successively chosen to be 2, 2.5 and 4 times that of the silicon carbide. For all experiments a Stokes’ settling velocity model, assuming that both particles are spherical, gives a settling rate of the glass beads that is greater than that of the silicon carbide by a factor ranging from 1.6 to 16.5. When the ratio of the Stokes’ settling velocity of the glass beads to that of the silicon carbide is greater than ∼6, we find a complete agreement between the box model and the experiment. In particular, the deposit shows a substantial decline in the mass of the coarser glass beads in the first metre, so that it only contains the finer silicon carbide further downstream. By contrast, when the Stokes’ settling velocity ratio is less than ∼4, only the speed of the current and the total sedimented mass can be well described by the box model. The experimental deposit is otherwise characterized by a slightly increasing density, which the box model fails to match. There is no difference in the deposit density across the valley. For all experiments in the V-shaped valley, the width of the currents decreases with time $t$ according to ${t}^{\ensuremath{-} 2/ 7} $. Analogue experiments in a flat-bottom tank were also performed to assess the influence of the valley on the sedimentation dynamics described above. A similar behaviour with settling velocity ratios was found. This study eventually shows the need for considering particle interactions in even dilute gravity currents at high Reynolds numbers.

Dyachenko & Zakharov (J. Expl Theor. Phys. Lett., vol. 93, 2011, pp. 701–705) recently derived a compact form of the well-known Zakharov integro-differential equation for the third-order Hamiltonian dynamics of a potential flow of an incompressible, infinitely deep fluid with a free surface. Special travelling wave solutions of this compact equation are numerically constructed using the Petviashvili method. Their stability properties are also investigated. In particular, unstable travelling waves with wedge-type singularities, namely peakons, are numerically discovered. To gain insight into the properties of these singular solutions, we also consider the academic case of a perturbed version of the compact equation, for which analytical peakons with exponential shape are derived. Finally, by means of an accurate Fourier-type spectral scheme it is found that smooth solitary waves appear to collide elastically, suggesting the integrability of the Zakharov equation.

Equations are derived that relate the vertical hydrodynamic force on two- and three-dimensional structures that are floating in a fluid of infinite depth to the far-field dipole coefficient in the velocity potential. By an application of Green’s theorem to the radiation or scattering potential and a suitable test potential, the heave added mass, the heave damping and the vertical exciting force are shown to be expressible in terms of the dipole coefficient in the relevant potential. The results add to the known reciprocity relations, which relate quantities such as the damping and the exciting force to the amplitude of the far-field radiated wave. The expressions are valid at all frequencies, and their high- and low-frequency asymptotics are investigated.