We study the mechanisms of centrifugal instability and its eventual self-limitation, as well as regenerative instability on a vortex column with a circulation overshoot (potentially unstable) via direct numerical simulations of the incompressible Navier–Stokes equations. The perturbation vorticity (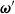 ${\boldsymbol{\omega} }^{\prime } $) dynamics are analysed in cylindrical (
${\boldsymbol{\omega} }^{\prime } $) dynamics are analysed in cylindrical (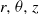 $r, \theta , z$) coordinates in the computationally accessible vortex Reynolds number,
$r, \theta , z$) coordinates in the computationally accessible vortex Reynolds number, 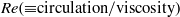 $\mathit{Re}({\equiv }\mathrm{circulation/viscosity} )$, range of 500–12 500, mostly for the axisymmetric mode (azimuthal wavenumber
$\mathit{Re}({\equiv }\mathrm{circulation/viscosity} )$, range of 500–12 500, mostly for the axisymmetric mode (azimuthal wavenumber 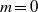 $m= 0$). Mean strain generates azimuthally oriented vorticity filaments (i.e. filaments with azimuthal vorticity,
$m= 0$). Mean strain generates azimuthally oriented vorticity filaments (i.e. filaments with azimuthal vorticity, 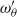 ${ \omega }_{\theta }^{\prime } $), producing positive Reynolds stress necessary for energy growth. This
${ \omega }_{\theta }^{\prime } $), producing positive Reynolds stress necessary for energy growth. This 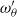 ${ \omega }_{\theta }^{\prime } $ in turn tilts negative mean axial vorticity,
${ \omega }_{\theta }^{\prime } $ in turn tilts negative mean axial vorticity, 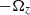 $- {\Omega }_{z} $ (associated with the overshoot), to amplify the filament, thus causing instability. (The initial energy growth rate (
$- {\Omega }_{z} $ (associated with the overshoot), to amplify the filament, thus causing instability. (The initial energy growth rate ( ${\sigma }_{r} $), peak energy (
${\sigma }_{r} $), peak energy (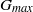 ${G}_{\mathit{max}} $) and time of peak energy (
${G}_{\mathit{max}} $) and time of peak energy (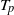 ${T}_{p} $) are found to vary algebraically with
${T}_{p} $) are found to vary algebraically with 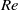 $\mathit{Re}$.) Limitation of vorticity growth, also energy production, occurs as the filament moves the overshoot outward, hence lessening and shifting
$\mathit{Re}$.) Limitation of vorticity growth, also energy production, occurs as the filament moves the overshoot outward, hence lessening and shifting 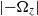 $\vert {- }{\Omega }_{z} \vert $, while also transporting the core
$\vert {- }{\Omega }_{z} \vert $, while also transporting the core 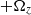 $+ {\Omega }_{z} $, to the location of the filament. We discover that a basic change in overshoot decay behaviour from viscous to inviscid occurs at
$+ {\Omega }_{z} $, to the location of the filament. We discover that a basic change in overshoot decay behaviour from viscous to inviscid occurs at 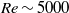 $Re\sim 5000$. We also find that the overshoot decay time has an asymptotic limit of 45 turnover times with increasing
$Re\sim 5000$. We also find that the overshoot decay time has an asymptotic limit of 45 turnover times with increasing 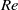 $\mathit{Re}$. After the limitation, the filament generates negative Reynolds stress, concomitant energy decay and hence self-limitation of growth; these inviscid effects are enhanced further by viscosity. In addition, the filament transports angular momentum radially inward, which can produce a new circulation overshoot and renewed instability. Energy decays at the
$\mathit{Re}$. After the limitation, the filament generates negative Reynolds stress, concomitant energy decay and hence self-limitation of growth; these inviscid effects are enhanced further by viscosity. In addition, the filament transports angular momentum radially inward, which can produce a new circulation overshoot and renewed instability. Energy decays at the 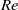 $\mathit{Re}$ studied, but, at higher
$\mathit{Re}$ studied, but, at higher  $\mathit{Re}$, regenerative growth of energy is likely due to the renewed mean shearing. New generation of overshoot and Reynolds stress is examined using a helical (
$\mathit{Re}$, regenerative growth of energy is likely due to the renewed mean shearing. New generation of overshoot and Reynolds stress is examined using a helical ( $m= 1$) perturbation. Regenerative energy growth, possibly resulting in even vortex breakup, can be triggered by this new overshoot at practical
$m= 1$) perturbation. Regenerative energy growth, possibly resulting in even vortex breakup, can be triggered by this new overshoot at practical 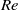 $\mathit{Re}$ (
$\mathit{Re}$ (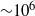 ${\sim }1{0}^{6} $ for trailing vortices), which are currently beyond the computational capability.
${\sim }1{0}^{6} $ for trailing vortices), which are currently beyond the computational capability.
 $18{0}^{\circ } $ contact angle
$18{0}^{\circ } $ contact angle