We use the method of Ash and Stevens to prove the existence of small slope $p$-adic families of cohomological modular forms for an indefinite quaternion algebra $B$. We prove that the Jacquet–Langlands correspondence relating modular forms on $\text{G}{{\text{L}}_{\text{2}}}/\mathbb{Q}$ and cohomomological modular forms for $B$ is compatible with the formation of $p$-adic families. This result is an analogue of a theorem of Chenevier concerning definite quaternion algebras.

We study unitary quotients of the free product unitary pivotal category ${{A}_{2}}\,*\,{{T}_{2}}$. We show that such quotients are parametrized by an integer $n\,\ge \,1$ and an $2n$–th root of unity $\omega $. We show that for $n\,=\,1,\,2,\,3$, there is exactly one quotient and $\omega \,=\,1$. For $4\,\le \,n\,\le \,10$, we show that there are no such quotients. Our methods also apply to quotients of ${{T}_{2}}\,*\,{{T}_{2}}$, where we have a similar result.

The essence of our method is a consistency check on jellyfish relations. While we only treat the specific cases of ${{A}_{2}}\,*\,{{T}_{2}}$ and ${{T}_{2}}\,*\,{{T}_{2}}$, we anticipate that our technique can be extended to a general method for proving the nonexistence of planar algebras with a specified principal graph.

During the preparation of this manuscript, we learnt of Liu's independent result on composites of ${{A}_{3}}$ and ${{A}_{4}}$ subfactor planar algebras (arxiv:1308.5691). In 1994, Bisch–Haagerup showed that the principal graph of a composite of ${{A}_{3}}$ and ${{A}_{4}}$ must fit into a certain family, and Liu has classified all such subfactor planar algebras. We explain the connection between the quotient categories and the corresponding composite subfactor planar algebras. As a corollary of Liu's result, there are no such quotient categories for $n\,\ge \,4$.

This is an abridged version of arxiv:1308.5723.

We formulate and prove a “winding number” index theorem for certain “Toeplitz” operators in the same spirit as Gohberg–Krein, Lesch and others. The “number” is replaced by a self-adjoint operator in a subalgebra 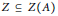$Z\,\subseteq \,Z\left( A \right)$ of a unital $C*$-algebra, 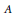$A$. We assume a faithful 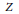$Z$-valued trace $\tau $ on 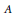$A$ left invariant under an action 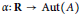$\alpha :\,\text{R}\,\to \,\text{Aut}\left( A \right)$ leaving 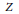$Z$ pointwise fixed. If 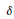$\delta $ is the infinitesimal generator of 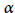$\alpha $ and 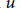$u$ is invertible in 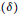$\text{dom}\left( \delta \right)$, then the “winding operator” of 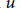$u$ is 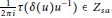$\frac{1}{2\pi i}\tau \left( \delta \left( u \right){{u}^{-1}} \right)\,\in \,{{Z}_{sa}}$. By a careful choice of representations we extend $\left( A,\,Z,\,\tau ,\,\alpha \right)$ to a von Neumann setting 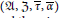$\left( \mathfrak{A},\,\mathfrak{Z},\,\bar{\tau },\,\bar{\alpha } \right)$ where 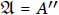$\mathfrak{A}\,=\,{A}''$ and 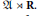$\mathfrak{Z}\,=\,{Z}''$. Then $A\,\subset \,\mathfrak{A}\,\subset \,\mathfrak{A}\,\rtimes \,\mathbf{R}$, the von Neumann crossed product, and there is a faithful, dual 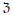$\mathfrak{Z}$-trace on $\mathfrak{A}\,\rtimes \,\mathbf{R}$. If 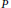$P$ is the projection in $\mathfrak{A}\,\rtimes \,\mathbf{R}$ corresponding to the non-negative spectrum of the generator of $\mathbf{R}$ inside $\mathfrak{A}\,\rtimes \,\mathbf{R}$ and 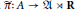$\tilde{\pi }:\,A\,\to \,\mathfrak{A}\,\rtimes \,\mathbf{R}$ is the embedding, then we define 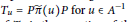${{T}_{u}}\,=\,P\tilde{\pi }\left( u \right)P\,\text{for}\,u\,\in \,{{A}^{-1}}$ and show it is Fredholm in an appropriate sense and the 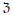$\mathfrak{Z}$-valued index of ${{T}_{u}}$ is the negative of the winding operator. In outline the proof follows that of the scalar case done previously by the authors. The main difficulty is making sense of the constructions with the scalars replaced by 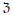$\mathfrak{Z}$ in the von Neumann setting. The construction of the dual 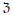$\mathfrak{Z}$-trace on $\mathfrak{A}\,\rtimes \,\mathbf{R}$ requires the nontrivial development of a 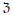$\mathfrak{Z}$-Hilbert algebra theory. We show that certain of these Fredholm operators fiber as a “section” of Fredholm operators with scalar-valued index and the centre-valued index fibers as a section of the scalar-valued indices.

The notion of positive-definite functions over locally compact quantum groups was recently introduced and studied by Daws and Salmi. Based on this work, we generalize various well-known results about positive-definite functions over groups to the quantum framework. Among these are theorems on “square roots” of positive-definite functions, comparison of various topologies, positive-definite measures and characterizations of amenability, and the separation property with respect to compact quantum subgroups.

This article provides an account of the functorial correspondence between irreducible singular $G$-monopoles on ${{S}^{1}}\,\times \,\sum $ and $\vec{t}$-stable meromorphic pairs on $\sum $. A theorem of B.Charbonneau and J. Hurtubise is thus generalized here from unitary to arbitrary compact, connected gauge groups. The required distinctions and similarities for unitary versus arbitrary gauge are clearly outlined, and many parallels are drawn for easy transition. Once the correspondence theorem is complete, the spectral decomposition is addressed.

Assuming Lang's conjectured lower bound on the heights of non-torsion points on an elliptic curve, we show that there exists an absolute constant $C$ such that for any elliptic curve $E/\mathbb{Q}$ and non-torsion point $P\,\in \,E\left( \mathbb{Q} \right)$, there is at most one integral multiple $\left[ n \right]P$ such that $n\,>\,C$. The proof is a modification of a proof of Ingram giving an unconditional, but not uniform, bound. The new ingredient is a collection of explicit formulæ for the sequence $v\left( {{\Psi }_{n}} \right)$ of valuations of the division polynomials. For $P$ of non-singular reduction, such sequences are already well described in most cases, but for $P$ of singular reduction, we are led to define a new class of sequences called elliptic troublemaker sequences, which measure the failure of the Néron local height to be quadratic. As a corollary in the spirit of a conjecture of Lang and Hall, we obtain a uniform upper bound on $\widehat{h}\left( P \right)/h\left( E \right)$ for integer points having two large integral multiples.

In this work type II Hermite-Padé approximants for a vector of Cauchy transforms of smooth Jacobi-type densities are considered. It is assumed that densities are supported on mutually disjoint intervals (an Angelesco system with complex weights). The formulae of strong asymptotics are derived for any ray sequence of multi-indices.