Let 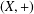 $(X,+)$ be an Abelian group and
$(X,+)$ be an Abelian group and 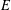 $E$ be a Banach space. Suppose that
$E$ be a Banach space. Suppose that 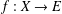 $f:X\rightarrow E$ is a surjective map satisfying the inequality
$f:X\rightarrow E$ is a surjective map satisfying the inequality 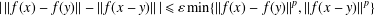 $$\begin{eqnarray}|\,\Vert f(x)-f(y)\Vert -\Vert f(x-y)\Vert \,|\leq {\it\varepsilon}\min \{\Vert f(x)-f(y)\Vert ^{p},\Vert f(x-y)\Vert ^{p}\}\end{eqnarray}$$
$$\begin{eqnarray}|\,\Vert f(x)-f(y)\Vert -\Vert f(x-y)\Vert \,|\leq {\it\varepsilon}\min \{\Vert f(x)-f(y)\Vert ^{p},\Vert f(x-y)\Vert ^{p}\}\end{eqnarray}$$ ${\it\varepsilon}>0$,
${\it\varepsilon}>0$, 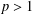 $p>1$ and for all
$p>1$ and for all 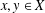 $x,y\in X$. We prove that
$x,y\in X$. We prove that  $f$ is an additive map. However, this result does not hold for
$f$ is an additive map. However, this result does not hold for 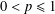 $0<p\leq 1$. As an application, we show that if
$0<p\leq 1$. As an application, we show that if 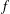 $f$ is a surjective map from a Banach space
$f$ is a surjective map from a Banach space 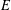 $E$ onto a Banach space
$E$ onto a Banach space 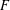 $F$ so that for some
$F$ so that for some 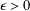 ${\it\epsilon}>0$ and
${\it\epsilon}>0$ and  $p>1$
$p>1$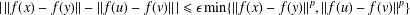 $$\begin{eqnarray}|\,\Vert f(x)-f(y)\Vert -\Vert f(u)-f(v)\Vert \,|\leq {\it\epsilon}\min \{\Vert f(x)-f(y)\Vert ^{p},\Vert f(u)-f(v)\Vert ^{p}\}\end{eqnarray}$$
$$\begin{eqnarray}|\,\Vert f(x)-f(y)\Vert -\Vert f(u)-f(v)\Vert \,|\leq {\it\epsilon}\min \{\Vert f(x)-f(y)\Vert ^{p},\Vert f(u)-f(v)\Vert ^{p}\}\end{eqnarray}$$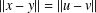 $\Vert x-y\Vert =\Vert u-v\Vert$, then
$\Vert x-y\Vert =\Vert u-v\Vert$, then  $f$ preserves equality of distance. Moreover, if
$f$ preserves equality of distance. Moreover, if  $\dim E\geq 2$, there exists a constant
$\dim E\geq 2$, there exists a constant 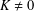 $K\neq 0$ such that
$K\neq 0$ such that 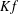 $Kf$ is an affine isometry. This improves a result of Vogt [‘Maps which preserve equality of distance’, Studia Math.45 (1973) 43–48].
$Kf$ is an affine isometry. This improves a result of Vogt [‘Maps which preserve equality of distance’, Studia Math.45 (1973) 43–48].