We perform direct numerical simulations of soluble bubbles dissolving in a Taylor–Couette (TC) flow reactor with a radius ratio of $\eta =0.5$ and Reynolds number in the range $0 \leq Re \leq 5000$, which covers the main regimes of this flow configuration, up to fully turbulent Taylor vortex flow. The numerical method is based on a geometric volume-of-fluid framework for incompressible flows coupled with a phase-change solver that ensures mass conservation of the soluble species, whilst boundary conditions on solid walls are enforced through an embedded boundary approach. The numerical framework is validated extensively against single-phase TC flows and competing mass transfer in multicomponent mixtures for an idealised infinite cylinder and for a bubble rising in a quiescent liquid. Our results show that when bubbles in a TC flow are mainly driven by buoyancy, theoretical formulae derived for spherical interfaces on a vertical trajectory still provide the right fundamental relationship between the bubble Reynolds and Sherwood numbers, which reduces to $Sh \propto \sqrt {Pe}$ for large Péclet values. For bubbles mainly transported by TC flows, the dissolution of bubbles depend on the TC Reynolds number and, for the turbulent configurations, we show that the smallest characteristic turbulent scales control mass transfer, in agreement with the small-eddy model of Lamont & Scott (AIChE J., vol. 16, 1970, pp. 513–519). Finally, the interaction between two aligned bubbles is investigated and we show that a significant increase in mass transfer can be obtained when the rotor of the apparatus is operated at larger speeds.

Embedding physical knowledge into neural network (NN) training has been a hot topic. However, when facing the complex real world, most of the existing methods still strongly rely on the quantity and quality of observation data. Furthermore, the NNs often struggle to converge when the solution to the real equation is very complex. Inspired by large eddy simulation in computational fluid dynamics, we propose an improved method based on filtering. We analysed the causes of the difficulties in physics-informed machine learning, and proposed a surrogate constraint (filtered partial differential equation, FPDE) of the original physical equations to reduce the influence of noisy and sparse observation data. In the noise and sparsity experiment, the proposed FPDE models (which are optimized by FPDE constraints) have better robustness than the conventional PDE models. Experiments demonstrate that the FPDE model can obtain the same quality solution with 100 % higher noise and 12 % quantity of observation data of the baseline. Besides, two groups of real measurement data are used to show the FPDE improvements in real cases. The final results show that the FPDE still gives more physically reasonable solutions when facing the incomplete equation problem and the extremely sparse and high-noise conditions. The proposed FPDE constraint is helpful for merging real-world experimental data into physics-informed training, and it works effectively in two real-world experiments: simulating cell movement in scratches and blood velocity in vessels.

Three-dimensional numerical simulations of hypersonic boundary layer transition delay due to porosity representative of carbon-fibre-reinforced carbon-matrix ceramics (C/C) were carried out on a 7$^{\circ {}}$ half-angle cone for unit Reynolds numbers $Re_m=2.43 \times 10^6$–$6.40\times 10^6\ \text {m}^{-1}$, at the free-stream Mach number $M_\infty =7.4$, for both sharp and 2.5 mm nose tip radii. A broadband time-domain impedance boundary condition was used to model the acoustic effects of the porous surface on the flow field. A quasi-spectral sub-filter-scale dynamic closure was adopted to stabilize the computations upon turbulent breakdown under extreme cooling conditions, with wall-to-adiabatic temperature ratio of $T_{w}/ T_{ad} \simeq 0.08 $, while accurately recovering the growth rates of the unstable modes present in the early transition stages. Good agreement is observed with the reference experimental data, both in terms of the predicted extent of the transition delay and the measured second-mode frequency spectrum. The latter is strongly modulated by the formation of near-wall low-temperature three-dimensional streaks. Pressure disturbances concentrate in corridors of locally thickened boundary layer, with frequencies lower than what predicted by linear theory. Here, trapped wavetrains are formed, which can persist long into the turbulent region. Finally, it is shown that the presence of a porous wall simply shifts the onset of turbulence downstream, without affecting its structure.

Although the interaction between microswimmers and walls during near-wall swimming has been extensively studied, the effect of microswimmer shapes and slip boundary conditions on the dynamic characteristics of near-wall microswimmers has received less attention. In this study, elliptical microswimmer models have been developed with various aspect ratios based on circular microswimmers. The lattice Boltzmann method has been used for the numerical simulation of the dynamic behaviour of microswimmers near walls. Under slip boundary conditions, the escape or capture of microswimmers by the walls is influenced by the swimming Reynolds number (Res), wall slip length (ls) and the aspect ratio (Cab) of a microswimmer. Changes in the Cab value of a microswimmer considerably affect its swimming state, especially for puller-type microswimmers. The tendency of pullers to be captured by the wall increases with increasing Cab. Moreover, changes in ls within the slip boundary condition of a puller can induce a transition in its movement state from a wall oscillation state to a stable sliding state and eventually to a wall lock-up state, a process influenced by the Cab value of the puller. Pusher-type microswimmers show a considerably increased tendency to escape from walls with increasing Cab and no wall lock-up state is observed, which is opposite to the case of pullers. Pushers and pullers show an increased tendency to be captured by the wall with increasing initial swimming angle of the microswimmer. The findings of this study enhance our understanding of the swimming patterns of natural microswimmers near walls and are of substantial importance for the design of artificial microswimmers and microfluidic devices.

We study the effect of acceleration and deceleration on the stability of channel flows. To do so, we derive an exact solution for laminar profiles of channel flows with an arbitrary, time-varying wall motion and pressure gradient. This solution then allows us to investigate the stability of any unsteady channel flow. In particular, we restrict our investigation to the non-normal growth of perturbations about time-varying base flows with exponentially decaying acceleration and deceleration, with comparisons to growth about a constant base flow (i.e. the time-invariant simple shear or parabolic profile). We apply this acceleration and deceleration through the velocity of the walls and through the flow rate. For accelerating base flows, perturbations never grow larger than perturbations about a constant base flow, while decelerating flows show massive amplification of perturbations – at a Reynolds number of $500$, properly timed perturbations about the decelerating base flow grow $ {O}(10^5)$ times larger than perturbations grow about a constant base flow. This amplification increases as we raise the rate of deceleration and the Reynolds number. We find that this amplification arises due to a transition from spanwise perturbations leading to the largest amplification to streamwise perturbations leading to the largest amplification that only occurs in the decelerating base flow. By evolving the optimal perturbations through the linearized equations of motion, we reveal that the decelerating base flow achieves this massive amplification through the Orr mechanism, or the down-gradient Reynolds stress mechanism, which accelerating and constant base flows cannot maintain.

Stratified wakes past an isolated conical seamount are simulated at a Froude number of $Fr = 0.15$ and Rossby numbers of $Ro = 0.15$, 0.75 and $\infty$. The wakes exhibit a Kármán vortex street, unlike their unstratified, non-rotating counterpart. Vortex structures are studied in terms of large-scale global modes, as well as spatially localised vortex evolution, with a focus on rotation effects. The global modes are extracted by spectral proper orthogonal decomposition (SPOD). For all three studied $Ro$ ranging from mesoscale, submesoscale and non-rotating cases, the frequency of the SPOD modes at different heights remains coupled as a global constant. However, the shape of the SPOD modes changes from slanted ‘tongues’ at zero rotation ($Ro=\infty$) to tall hill-height columns at strong rotation ($Ro=0.15$). A novel method for vortex centre tracking shows that, in all three cases, the vortices at different heights advect uniformly at approximately $0.9U_{\infty }$ beyond the near wake, consistent with the lack of variability of the global modes. Under system rotation, cyclonic vortices and anticyclonic vortices (AVs) present considerable asymmetry, especially at $Ro = 0.75$. The vorticity distribution as well as the stability of AVs are tracked downstream using statistics conditioned to the identified vortex centres. At $Ro=0.75$, intense AVs with relative vorticity up to $\omega _z/f_{c}=-2.4$ (where $\omega_z$ is the vertical vorticity and $f_c$ is the Coriolis frequency) are seen with small regions of instability and they maintain large $\omega _z/f_{c}$ magnitude in the far wake. Recent stability analysis that accounts for stratification and viscosity is found to improve on earlier criteria and show that these intense AVs are stable.

We present a simulation-based study of the effect of a passing wave packet on underlying fully developed turbulence. We propose a novel wave-phase-resolved simulation method inspired by Helmholtz decomposition to directly couple the turbulence simulation with instantaneous wave orbital motions without wave-phase averaging. We also introduce a boundary condition treatment for the turbulence at the wave surface, which allows the turbulence simulation to be conducted in a rectangular domain while retaining the wave-phase effect. The results obtained from the proposed method reveal considerable variations in turbulence statistics, including the enstrophy and Reynolds normal stresses, during wave packet passage. Most changes occur rapidly when the narrow bandwidth around the wave packet core passes. Further analyses of the energy spectra indicate that the enhancement of turbulence occurs across a wide range of scales, with the near-surface small-scale motions experiencing the most significant intensification. Meanwhile, large-scale motions with scales comparable to the boundary layer depth are also enhanced. The mechanisms underlying the Reynolds normal stress variation at different length scales are related to the energy transfer from the wave orbital straining to turbulence through production, the pressure–strain effect, the pressure diffusion and the wave advection. By assessing the turbulence statistics and dynamics impacted by a wave packet in detail, this study provides an improved understanding of the response of a developed turbulent flow to a transient wave field. The proposed simulation method also proves to be a promising phase-resolved approach for efficiently modelling the wave effect on turbulence.

Explosive dispersal of granular media widely occurs in nature across various length scales, enabling engineering applications ranging from commercial or military explosive systems to the loss prevention industry. However, the correlation between the explosive dispersal behaviour and the structure of dispersal system is far from completely understood, thereby compromising the prediction of the explosive dispersal outcome resulting from a specific dispersal system. Here, we investigate the dispersal behaviours of densely packed particle rings driven by the enclosed pressurized gases using coarse-grained computational fluid dynamics–discrete parcel method. Distinct dispersal modes emerge from the dispersal systems with vastly varying sets of the macro- and micro-scale structural parameters in terms of the dispersal completeness and the spatial uniformity of the dispersed mass. Further investigation reveals the variation in the dispersal modes arises from the collective effects of multiscale gas–particle coupling relationships. Specifically, the macroscale coupling dictates the cyclic momentum/energy transfer between gases and particle ring as an entirety. The mesoscale coupling relates to the inter-pore gas filtration through the thickness of the particle ring, leading to the mass/energy reduction of the explosive source. The microscale coupling involves the individual particle dynamics influenced by the local flow parameters. A persistent macroscale coupling results in an incomplete dispersal which takes the form of an aggregated annular band, whereas the meso- and micro-scale couplings alter the macroscale coupling to a different extent. By incorporating the effects of the variety of structural parameters on the multiscale gas–particle coupling relationships, a non-dimensional parameter referred to as the modified mass ratio is constructed, which shows an explicit correlation with the dispersal mode. We proceed to establish a dispersal ring model in the continuum frame which accounts for the macro and meso-scale coupling effects. This model proves to be capable of successfully predicting the ideal and validated failed dispersal modes.

Guided-jet waves have been shown to close resonance loops in a myriad of problems such as screech and impingement tones in jets. These discrete, upstream-travelling waves have long been identified in linear-stability models of jet flows, but in this work they are instead considered in the context of an acoustic-scattering problem. It is shown that the guided-jet mode results from total internal reflection and transmission of acoustic waves, arising from the shear layer behaving like a duct with some given wall impedance. After total reflection, only discrete streamwise wavenumbers may be supported by the flow, with these wavenumbers dictated by the fact that the standing wave formed inside of the jet must fit between the two shear layers. Close to the sonic line, the transmission of this mode to the outside is maximum, leading to a net-energy flux directed upstream, which dictates the direction of propagation of this mode, providing a clear connection to the better understood soft-duct mode (Towne et al., J. Fluid Mech., vol. 825, 2017, pp. 1113–1152). The model also indicates that these waves are generated in the core of the flow and can only be efficiently transmitted to the quiescent region under certain conditions, providing an explanation as to why screech is only observed at conditions where the discrete mode is supported by the flow. The present results explain, for the first time, the nature and characteristics of the guided-jet waves.

The Taylor–Maccoll (T–M) equations are the governing equations for steady inviscid irrotational axisymmetric conical flow, and have been widely applied to the design of waveriders and intakes. However, only four classic solutions have been reported: external conical flow (ECF), Busemann flow and internal conical flow of types A and B (ICFA and ICFB). In this work, the analysis of the T–M equations clarifies all possible solutions and reveals their relations. The domain where elementary solutions exist is divided into four domains. The classic Busemann and ICFB solutions share the same elementary solution as the template in a domain called the pre-shock domain, while the classic ECF and ICFA solutions belong to a domain named the ECF domain. Two new solutions, the inner flow of ECF (IECF) and degenerate conical flow (DCF), are found in the domains named after the corresponding solutions, namely the IECF and DCF domains. The IECF behaves as the mass injection supporting the classic ECF on an imaginary cone surface, while the DCF behaves as the conical expansion of a uniform flow. Furthermore, possible combinations of pre-shock solutions and supersonic post-shock solutions are clarified. The classic solutions are special cases where the pre-/post-shock solutions are combined with uniform flows. In general, the Busemann and ICFB solutions can be combined with any post-shock solutions in accord with the shock relations, including the ECF, ICFA, IECF and DCF solutions. In addition, numerical analyses are conducted to verify the validity of the two new solutions, DCF, IECF and one combined solution Busemann–ECF.

We conduct direct numerical simulations (DNS) to study the temporal and spatial developments of the roll waves on a laminar sheet flow of Newtonian fluid. The DNS unveil the physics of the wavefront and show the limitation of the widely used shallow-layer approximations. The most prominent wave, the front runner, is determined by the DNS for the first time in studying the spatial development of the laminar sheet flow with negligible surface tension. Depending on the Froude and Reynolds numbers, the front runner can be a multi-peaked undular bore or a single-peaked non-breaking or breaking wave. The simulation has uncovered an extended region behind the wavefront, where the bed-friction stress is much higher than the corresponding friction in the undisturbed uniform flow. It also produces an uplift velocity needed in the description of wave breaking. For comparison, we also examine the nonlinear development of the instability using two-equation and four-equation shallow-layer models. The two-equation shallow-layer model has produced the bulk of the wave profile but is deficient because it fails to predict the uplift velocity and the substantial increase in bed friction in the frontal region. The four-equation shallow-layer model correctly predicts the bed friction but cannot produce the breaking wave. The simulations also determine the celerity and amplitude of the front runner to follow a linear relationship, qualitatively similar to the roll waves in a turbulent flow.

A stream of free-falling particles from a rectangular hopper, hereafter called a ‘curtain’, was characterised systematically using a well-resolved, non-intrusive optical shadowgraphic method, to reveal both an additional axial region and an additional dilute region distributed laterally on either side of the curtain, relative to those identified previously. The effects of particle size and hopper outlet thickness on the evolution of the particle curtain were separately isolated, whilst measuring particle mass flow rate. The curtains were characterised into four distinct axial regions, namely a near-field expansion region near to the hopper exit, a neck zone where the curtain contracts, a region of intermediate-field expansion and a far field with particles reaching terminal velocity. The initial expansion half-angle, $2.3^{\circ } \le \alpha \le 4.8^{\circ }$, was found to be insensitive to particle size, but to increase with hopper outlet thickness. The ‘trough’ in the neck zone was deduced to be caused by a pressure gradient driven by particle acceleration. The curtain expansion rate at the intermediate field was found to increase with a decrease in particle size and hopper outlet thickness. The outermost dilute-particle region was deduced to be caused by collisions, induced by gradients in the velocity profile near to the hopper exit. New dimensionless analysis reveals that the dynamics of curtains can be characterised broadly into two regimes, one in which the aerodynamics is dominant and the other where it is weak. Curtain transmittance was found to scale with the Froude number, highlighting the importance of particle momentum.

Direct numerical simulations of spanwise-rotating turbulent channel flow with a parabolic bump on the bottom wall are employed to investigate the effects of rotation on flow separation. Four rotation rates, $Ro_b := 2\varOmega H/U_b = \pm 0.42$, $\pm$1.0, are compared with the non-rotating scenario. The mild adverse pressure gradient induced by the lee side of the bump allows for a variable pressure-induced separation. The separation region is reduced (increased) when the bump is on the anti-cyclonic (cyclonic) side of the channel, compared with the non-rotating separation. The total drag is reduced in all rotating cases. Through several mechanisms, rotation alters the onset of separation, reattachment and wake recovery. The mean momentum deficit is found to be the key. A physical interpretation of the ratio between the system rotation and mean shear vorticity, $S:=\varOmega /\varOmega _s$, provides the mechanisms regarding stability thresholds $S=-0.5$ and $-$1. The rotation effects are explained accordingly, with reference to the dynamics of several flow structures. For anti-cyclonic separation, particularly, the interaction between the Taylor–Görtler vortices and hairpin vortices of wall-bounded turbulence is proven to be responsible for the breakdown of the separating shear layer. A generalized argument is made regarding the essential role of near-wall deceleration and resultant ejection of enhanced hairpin vortices in destabilizing an anti-cyclonic flow. This mechanism is anticipated to have broad impacts on other applications in analogy to rotating shear flows, such as thermal convection and boundary layers over concave walls.

This study investigates the correlation between the fluctuating wall heat flux, and the distribution and transport of Reynolds shear stress and turbulent heat flux in compressible boundary layers at Mach number 5.86 and friction Reynolds number 420, with a relatively weaker and a stronger wall cooling imposed. As illustrated from the probability density functions of the wall-heat-flux perturbations, with increasing wall cooling, the extreme wall heat flux is intensified and tends to be more negatively skewed. To examine the role of the extreme events in the transport of the momentum and heat, conditional analysis of the extreme positive and negative wall-heat-flux-perturbation events is conducted. In most regions of the boundary layer, the positive events are predominantly associated with an increase in Reynolds shear stress and a decrease in turbulent heat flux. Joint probability density functions of velocity and wall-heat-flux perturbations in the near-wall region indicate that the extreme positive events tend to be more correlated with ejections, which is particularly evident in the stronger wall-cooling case. To further shed light on the underlying mechanisms of the connections between wall heat flux and transport budgets, a transport equation for turbulent heat flux is derived, in a similar manner to that for Reynolds shear stress. The energy balance is inspected, with conditional analysis applied to budget terms and mean flow properties so as to quantify the correlation between wall-heat-flux fluctuations and energy evolution.

This work introduces a formulation of resolvent analysis that uses wavelet transforms rather than Fourier transforms in time. Under this formulation, resolvent analysis may extend to turbulent flows with non-stationary mean states. The optimal resolvent modes are augmented with a temporal dimension and are able to encode the time-transient trajectories that are most amplified by the linearised Navier–Stokes equations. We first show that the wavelet- and Fourier-based resolvent analyses give equivalent results for statistically stationary flow by applying them to turbulent channel flow. We then use wavelet-based resolvent analysis to study the transient growth mechanism in the near-wall region of a turbulent channel flow by windowing the resolvent operator in time and frequency. The computed principal resolvent response mode, i.e. the velocity field optimally amplified by the linearised dynamics of the flow, exhibits characteristics of the Orr mechanism, which supports the claim that this mechanism is key to linear transient energy growth. We also apply this method to non-stationary parallel shear flows such as an oscillating boundary layer, and three-dimensional channel flow in which a sudden spanwise pressure gradient perturbs a fully developed turbulent channel flow. In both cases, wavelet-based resolvent analysis yields modes that are sensitive to the changing mean profile of the flow. For the oscillating boundary layer, wavelet-based resolvent analysis produces oscillating principal forcing and response modes that peak at times and wall-normal locations associated with high turbulent activity. For the turbulent channel flow under a sudden spanwise pressure gradient, the resolvent modes gradually realign themselves with the mean flow as the latter deviates. Wavelet-based resolvent analysis thus captures the changes in the transient linear growth mechanisms caused by a time-varying turbulent mean profile.

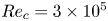The boundary-layer stability on a section of a rotating wind turbine blade with an FFA-W3 series aerofoil at a chord Reynolds number of $3 \times 10^5$, with varying rotation and radii, is studied with direct numerical simulations and linear stability analyses. Low rotation does not significantly affect transition in the outboard blade region. The relative insensitivity to rotation is due to a laminar separation bubble near the leading edge, spanwise-deformed by a primary self-excited instability, promoting the secondary absolute instability of the Kelvin–Helmholtz (KH) vortices and rapid transition. Moderate increases in rotation, or moving inboard, stabilise the flow by accelerating the attached boundary layer and possibly inducing competition between cross-flow and KH modes. This delays separation and transition. Initially, for high rotation rates or radial locations close to the hub, transition is delayed. Nevertheless, strong stationary and travelling cross-flow modes are eventually triggered, spanwise modulating the KH rolls and shifting the transition line close to the leading edge. Cross-flow velocities as high as $56\,\%$ of the free stream velocity directed towards the blade tip are reached at the transition location. For radial locations farther from the hub, the effective angle of attack is decreased, and cross-flow transition occurs at lower rotation rates. The advance or delay of the transition line compared with a non-rotating configuration depends on the competing rotation effects of stabilising the attached boundary layer and triggering cross-flow modes in the separation flow region.

We study the stability and dewetting dynamics of a thin free-surface film composed of two miscible liquids placed on a solid substrate. Our study focuses on the development of a self-consistent model such that the mixture concentration influences both free-surface and wetting energies. By assuming a simple relation between these energies and the bulk and surface concentrations, we analyse their effect on the concentration distribution and dewetting down to the equilibrium film thickness determined by the fluid–solid interaction potential. The model, developed within the gradient dynamics formulation, includes the dependence of the free-surface energy on surface concentration leading to the Marangoni effect, while a composition-dependent Hamaker constant describes the wetting energy resulting from the fluid–solid interaction. We analyse the restrictions that must be fulfilled to ensure an equilibrium state for a flat film of a binary fluid. Then, we proceed by studying its linear stability. First, we consider the Marangoni effect while assuming that wetting energy depends only on the fluid thickness. Then, we include a dependence of wetting energy on concentration and study its effects. We find that the linear stability results compare very well with those of numerical simulations of the full nonlinear problem applied to the particular case of a binary melted metal alloy, even close to breakup times. Therefore, in practice, most of the evolution can be studied by using the linear theory, simplifying the problem considerably.

Stochastically generated instantaneous velocity profiles are used to reproduce the outer region of rough-wall turbulent boundary layers in a range of Reynolds numbers extending from the wind tunnel to field conditions. Each profile consists in a sequence of steps, defined by the modal velocities and representing uniform momentum zones (UMZs), separated by velocity jumps representing the internal shear layers. Height-dependent UMZ is described by a minimal set of attributes: thickness, mid-height elevation, and streamwise (modal) and vertical velocities. These are informed by experimental observations and reproducing the statistical behaviour of rough-wall turbulence and attached eddy scaling, consistent with the corresponding experimental datasets. Sets of independently generated profiles are reorganized in the streamwise direction to form a spatially consistent modal velocity field, starting from any randomly selected profile. The operation allows one to stretch or compress the velocity field in space, increases the size of the domain and adjusts the size of the largest emerging structures to the Reynolds number of the simulated flow. By imposing the autocorrelation function of the modal velocity field to be anchored on the experimental measurements, we obtain a physically based spatial resolution, which is employed in the computation of the velocity spectrum, and second-order structure functions. The results reproduce the Kolmogorov inertial range extending from the UMZ and their attached-eddy vertical organization to the very-large-scale motions (VLSMs) introduced with the reordering process. The dynamic role of VLSM is confirmed in the $-u^{\prime }w^{\prime }$ co-spectra and in their vertical derivative, representing a scale-dependent pressure gradient contribution.

In this paper, we study the effect of lateral wall vibrations on the excitation and evolution of non-modal perturbations in hypersonic boundary layers subject to low-frequency freestream vortical disturbances (FSVDs). A novel, high-efficiency numerical approach, combining the harmonic weakly nonlinear Navier–Stokes and nonlinear parabolised stability equation approaches, is developed, which is sufficient to accommodate both the rapid distortion of the perturbation in the leading-edge vicinity and the nonlinear development of finite-amplitude high-order harmonics in the downstream region. The boundary-layer response to low-frequency FSVDs shows a longitudinal streaky structure, for which the temperature perturbation shows much greater magnitude than the streamwise velocity perturbation. The lateral vibration induces a Stokes layer solution for the spanwise velocity perturbation, which interacts with the FSVD-induced perturbations and leads to a suppression of the non-modal perturbation and an enhancement of the downstream modal perturbation. The new perturbations excited by the FSVD–vibration interaction strengthen as the vibration intensifies, and they could become comparable with the FSVD-induced perturbations in downstream locations at a high vibration intensity, indicating a remarkable modification of the streaky structure and its instability property. Secondary instability (SI) analyses based on the streaky base flow indicate that the vibration could enhance or suppress the SI modes, depending on their initial phases over the vibration period. Overall, the average effect is that the low-frequency and high-frequency SI modes are stabilised and destabilised by the vibration, respectively. Since the high-frequency SI modes undergo higher amplifications, the subsequent bypass transition is likely to be promoted by relatively strong vibrations.

A novel theoretical model for bubble dynamics is established that simultaneously accounts for the liquid compressibility, phase transition, oscillation, migration, ambient flow field, etc. The bubble dynamics equations are presented in a unified and concise mathematical form, with clear physical meanings and extensibility. The bubble oscillation equation can be simplified to the Keller–Miksis equation by neglecting the effects of phase transition and bubble migration. The present theoretical model effectively captures the experimental results for bubbles generated in free fields, near free surfaces, adjacent to rigid walls, and in the vicinity of other bubbles. Based on the present theory, we explore the effect of the bubble content by changing the vapour proportion inside the cavitation bubble for an initial high-pressure bubble. It is found that the energy loss of the bubble shows a consistent increase with increasing Mach number and initial vapour proportion. However, the radiated pressure peak by the bubble at the collapse stage increases with decreasing Mach number and increasing vapour proportion. The energy analyses of the bubble reveal that the presence of vapour inside the bubble not only directly contributes to the energy loss of the bubble through phase transition but also intensifies the bubble collapse, which leads to greater radiation of energy into the surrounding flow field due to the fluid compressibility.