Let f: T → T be a tree map with n end-points, SAP(f) the set of strongly almost periodic points of f and CR(f) the set of chain recurrent points of f. Write E(f,T) = {x: there exists a sequence {ki} with 2 ≤ ki ≤ n such that 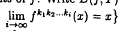and g = f\CR(f). In this paper, we show that the following three statements are equivalent:

(1) f has zero topological entropy.

(2) SAP(f) ⊂ E(f,T).

(3) Map ωg: x → ω(x,g) is continuous at p for every periodic point p of f.

This work considers the discrete subgroups of group of isometries of an Alexandrov space with a lower curvature bound. By developing the notion of Hausdorff distance in these groups, a rigidity theorem for the close discrete groups was proved.

In this paper, we introduce the concept of A-proper topological degree in Menger PN-space and study some of its properties. Utilising its properties, we obtain a new fixed point theorem.

In the paper “The topological degree of A-proper mapping in the Menger PN-space (I)”, the new concept of A-proper topological degree has been given. Now, utilising the new concept, we give the corresponding definitions of convex A-proper, P*-compact and Pγ-compact in Menger PN-space. As an application of these new concepts, we prove the existence of solution for some equations.

In this paper, we characterise the n-dimensional (n ≥ 3) complete spacelike hypersurfaces Mn in a de Sitter space with constant scalar curvature and with two distinct principal curvatures. We show that if the multiplicities of such principal curvatures are greater than 1, then Mn is isometric to Hk (sinh r) × Sn−k (cosh r), 1 < k < n − 1. In particular, when Mn is the complete spacelike hypersurfaces in with the scalar curvature and the mean curvature being linearly related, we also obtain a characteristic Theorem of such hypersurfaces.

Let p be a prime and m be a positive integer. For a positive integer n, let ep(n) be the nonnegative integer with pep(n) | n and pep(n)+1 ∤ n. As a corollary of our main result we derive an asymptotic formula for the counting function with regard to the condition ep(n!) ≡ ɛ (mod 3), where ɛ ∈ Z3. In 2001, Sander proved the result with modulus 2.

In this paper, we study the existence of positive periodic solutions to the equation x″ = f (t, x). It is proved that such a equation has more than one positive periodic solution when the nonlinearity changes sign. The proof relies on a fixed point theorem in cones.

In this paper, it is proved that, for any domain G of the complex plane, there exists an infinite-dimensional closed linear submanifold M1 and a dense linear submanifold M2 with maximal algebraic dimension in the space H(G) of holomorphic functions on G such that G is the domain of holomorphy of every nonzero member f of M1 or M2 and, in addition, the growth of f near each boundary point is as fast as prescribed.

We give some inequalities of Cauchy-Bunyakovsky-Schwarz type for sequences of bounded linear operators in Hilbert spaces with applications.

A Kuga fibre variety is a fibre bundle over a locally symmetric space whose fibre is a polarized Abelian variety. We describe a complex torus bundle associated to a 2-cocycle of a discrete group, which may be regarded as a generalized Kuga fibre variety, and prove the existence of such a bundle.

Let Γ be a group and Σ a symmetric generating set for Γ. In 1966, Stallings called Γ a unique factorisation group if each group element may be written in a unique way as a product a1…am, where ai ∈ Σ for each i and aiai+1 ∉ Σ ∪ {1} for each i < m. In this paper we give a complete combinatorial proof of a theorem, not explicitly stated by Stallings in 1966, characterising all such pairs (Γ, Σ). We also characterise the unique factorisation pairs by a certain tree-like property of their Cayley graphs.

In this paper we give a complete classification of -invariant or Hopf pseudo-Einstein real hypersurfaces in complex two-plane Grassmannians G2(ℂm+2).

In this paper, we show that if q(x) satisfies suitable conditions, then the Neumann problem -Δu+u = q(x)ⅠuⅠp−2u in Ω has at least two solutions of which one is positive and the other changes sign.

Let X be a real or complex Banach space and let U be a Banach space with an unconditional basis. We show that the projective tensor product of U and X, UX, has the complete continuity property (respectively, the analytic complete continuity property) whenever U and X have the complete continuity property (respectively, the analytic complete continuity property). More general versions of these results are also obtained. Moreover, the techniques applied here to the projective tensor product, can also be used to establish some Banach space properties of the Fremlin projective tensor product.

In the paper the asymptotic distribution of (|ζ(s)|,ζ(s)), where ζ(s) is the Riemann zeta - function, in the sense of weak convergence of probability measures is considered. For this, the continuity theorems for probability measures on ℝ × ℂ are used. Some aspects of the dependence of |ζ(s)| and ζ(s) are also discussed.

An integral representation formula in terms of the normal Gauss map for minimal surfaces in 3-dimensional solvable Lie groups with left invariant metric is obtained.

Motivated by constructions of Witt rings in the algebraic theory of quadratic forms, the authors construct new classes of finite commutative rings and explore some of their properties. These rings are constructed as quotient rings of a special class of integral group rings for which the group is an elementary 2-group. The new constructions are compared to other rings in the literature.

In 1996 Poland and Rhemtulla proved that the number v (G) of conjugacy classes of non-normal subgroups of a non-Hamiltonian nilpotent group G is at least c − 1, where c is the nilpotency class of G. In this paper we consider the map that associates to every conjugacy class of subgroups of a finite p-group the conjugacy class of the normaliser of any of its representatives. In spite of the fact that this map need not be injective, we prove that, for p odd, the number of conjugacy classes of normalisers in a finite p-group is at least c (taking into account the normaliser of the normal subgroups). In the case of p-groups of maximal class we can find a better lower bound that depends also on the prime p.

In this article using techniques which appeared in Witt's proof of Wedderburn's theorem, an arithmetical characterisation of groups acting on a set whose orbits are all singleton is given. This can then be used to obtain a new proof of Wedderburn's theorem.

We consider the symmetric inverse monoid ℐn of an n-element chain and its inverse submonoids ℐn, ℐn, ℐn and ℘ℐn of all order-preserving, order-preserving or order-reversing, orientation-preserving and orientation-preserving or orientation-reversing transformations, respectively, and give descriptions of their Abelian kernels relative to decidable pseudovarieties of Abelian groups.