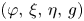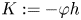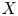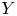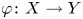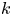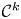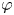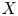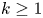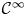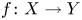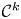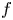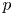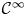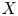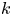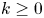This paper is concerned with the study on an open problem of classifying conformally flat minimal Legendrian submanifolds in the $(2n+1)$-dimensional unit sphere $\mathbb {S}^{2n+1}$ admitting a Sasakian structure $(\varphi,\,\xi,\,\eta,\,g)$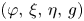 for $n\ge 3$, motivated by the classification of minimal Legendrian submanifolds with constant sectional curvature. First of all, we completely classify such Legendrian submanifolds by assuming that the tensor $K:=-\varphi h$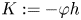 is semi-parallel, which is introduced as a natural extension of $C$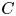-parallel second fundamental form $h$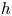. Secondly, such submanifolds have also been determined under the condition that the Ricci tensor is semi-parallel, generalizing the Einstein condition. Finally, as direct consequences, new characterizations of the Calabi torus are presented.

Let $X$, $Y$ be nonsingular real algebraic sets. A map $\varphi \colon X \to Y$ is said to be $k$-regulous, where $k$ is a nonnegative integer, if it is of class $\mathcal {C}^k$ and the restriction of $\varphi$ to some Zariski open dense subset of $X$ is a regular map. Assuming that $Y$ is uniformly rational, and $k \geq 1$, we prove that a $\mathcal {C}^{\infty }$ map $f \colon X \to Y$ can be approximated by $k$-regulous maps in the $\mathcal {C}^k$ topology if and only if $f$ is homotopic to a $k$-regulous map. The class of uniformly rational real algebraic varieties includes spheres, Grassmannians and rational nonsingular surfaces, and is stable under blowing up nonsingular centers. Furthermore, taking $Y=\mathbb {S}^p$ (the unit $p$-dimensional sphere), we obtain several new results on approximation of $\mathcal {C}^{\infty }$ maps from $X$ into $\mathbb {S}^p$ by $k$-regulous maps in the $\mathcal {C}^k$ topology, for $k \geq 0$.