Research Article
Infinitary formulas preserved under unions of models1
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 449-465
-
- Article
- Export citation
On n-quantifier induction
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 466-482
-
- Article
- Export citation
Addition in nonstandard models of arithmetic
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 483-486
-
- Article
- Export citation
Almost strongly minimal theories. I
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 487-493
-
- Article
- Export citation
Countable homogeneous relational structures and ℵ0-categorical theories
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 494-500
-
- Article
- Export citation
Decidability of the “almost all” theory of degrees
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 501-506
-
- Article
- Export citation
Characterization of recursively enumerable sets
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 507-511
-
- Article
- Export citation
Omitting quantifier-free types in generic structures
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 512-520
-
- Article
- Export citation
An elementary sentence which has ordered models
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 521-530
-
- Article
- Export citation
On power-like models for hyperinaccessible cardinals
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 531-537
-
- Article
- Export citation
The inconsistency of a certain axiom system for set theory
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 538-542
-
- Article
- Export citation
A note on frame extensions
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 543-545
-
- Article
- Export citation
The model-companion of a class of structures1
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 546-556
-
- Article
- Export citation
An algebraic difference between isols and cosimple isols
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 557-561
-
- Article
- Export citation
Theories without countable models
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 562-568
-
- Article
- Export citation
On the inadequacy of inner models
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 569-571
-
- Article
- Export citation
Some representations of Diophantine sets1
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 572-578
-
- Article
- Export citation
Decidability of some intuitionistic predicate theories
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 579-587
-
- Article
- Export citation
The Hanf number of second order logic1
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 588-594
-
- Article
- Export citation
On the undecidability of finite planar cubic graphs1
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 595-597
-
- Article
- Export citation

 , the first results were the theorems of Lopez-Escobar on sentences preserved under homomorphic images and of Malitz on formulas preserved under substructures. More recently, Feferman added a result on formulas preserved under (or persistent for) ∈-extensions. Some of these theorems will be considered in subsequent sections. A more thorough treatment may be found in Makkai [17]. The main new preservation result obtained here characterizes the
, the first results were the theorems of Lopez-Escobar on sentences preserved under homomorphic images and of Malitz on formulas preserved under substructures. More recently, Feferman added a result on formulas preserved under (or persistent for) ∈-extensions. Some of these theorems will be considered in subsequent sections. A more thorough treatment may be found in Makkai [17]. The main new preservation result obtained here characterizes the 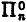 (or, equivalently,
(or, equivalently, 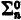 ) formulae and the rule of induction restricted to
) formulae and the rule of induction restricted to 
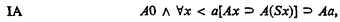
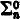 the restriction to
the restriction to 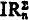 the restriction to
the restriction to 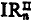 ,
, 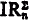 are analogous.
are analogous.
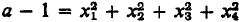 ,
, 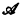 is an
is an 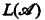 of
of 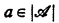 (the universe of
(the universe of 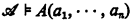 if in Shoenfield's notation
if in Shoenfield's notation 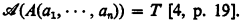 If
If 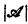 , then
, then 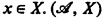 is the natural expansion of
is the natural expansion of  is an
is an 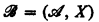 for some
for some 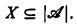 .
. of cardinality ℵ
of cardinality ℵ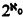 isomorphism types of such structures for the first order language
isomorphism types of such structures for the first order language 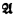 is
is 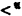 is a linear ordering of
is a linear ordering of 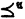 is a (reflexive) linear ordering of type
is a (reflexive) linear ordering of type 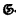 -system, an axiom system for set theory suggested for investigation by Takeuti in [2], is inconsistent. We also show that this system without the
-system, an axiom system for set theory suggested for investigation by Takeuti in [2], is inconsistent. We also show that this system without the  . The case when
. The case when 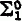 , nonrecursive was left open. We show in this note that the extension procedures in fact agree for all
, nonrecursive was left open. We show in this note that the extension procedures in fact agree for all 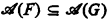 So let
So let 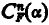 . Let
. Let 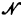 be the standard model for
be the standard model for 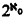 infinite subsets of
infinite subsets of 
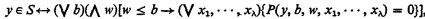
 instance of the axiom of replacement.
instance of the axiom of replacement. we will mean a nonempty set
we will mean a nonempty set