Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-19T04:21:08.685Z Has data issue: false hasContentIssue false
  • English
  • Français

The inconsistency of a certain axiom system for set theory

Published online by Cambridge University Press:  12 March 2014

James D. Davis
James D. Davis*
Affiliation:
Fairleigh Dickinson University, Madison, New Jersey 07940
Get access Rights & Permissions [Opens in a new window]

Extract

We prove in this paper that the 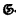-system, an axiom system for set theory suggested for investigation by Takeuti in [2], is inconsistent. We also show that this system without the ω-rule is consistent if Zermelo-Fraenkel set theory with the axiom of choice and an axiom due to Reinhardt and Silver is consistent. The -system is an effort to strengthen Bernays-Gödel set theory by adding a reflection principle.

In addition to the standard notation of set theory, we write X″{x) to mean {y∣〈x, y〉 Є X}.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 37 , Issue 3 , September 1972 , pp. 538 - 542
Copyright
Copyright © Association for Symbolic Logic 1972

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Gödel, K., The consistency of the continuum hypothesis, Princeton University Press, Princeton, N.J., 1953.Google Scholar
[2]Takeuti, G., Axioms of infinity of set theory, Journal of the Mathematical Society of Japan, vol. 13 (1961), pp. 220–233.CrossRefGoogle Scholar