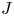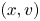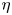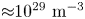Volume 91 - Issue 1 - February 2025
Research Article
Application of quadruple Beltrami state on Saturnian dusty plasma
-
- Published online by Cambridge University Press:
- 13 January 2025, E23
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Symplectic particle-in-cell methods for hybrid plasma models with Boltzmann electrons and space-charge effects
-
- Published online by Cambridge University Press:
- 15 January 2025, E22
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
The kinetic ion-temperature-gradient-driven instability and its localisation
-
- Published online by Cambridge University Press:
- 23 January 2025, E21
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Collisionless conduction in a high-beta plasma: a collision operator for whistler turbulence
-
- Published online by Cambridge University Press:
- 09 January 2025, E20
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Review Article
Multi-scale physics of magnetic reconnection in hot magnetized plasmas
- Part of:
-
- Published online by Cambridge University Press:
- 09 January 2025, E19
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Research Article
A family of quasi-axisymmetric stellarators with varied rotational transform
-
- Published online by Cambridge University Press:
- 09 January 2025, E18
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Modulational instability of geodesic-acoustic-mode packets
- Part of:
-
- Published online by Cambridge University Press:
- 10 January 2025, E17
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
A quasi-linear model of electromagnetic turbulent transport and its application to flux-driven transport predictions for STEP
-
- Published online by Cambridge University Press:
- 09 January 2025, E16
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Letter
Measurement of the properties of the dust acoustic wave in a magnetic field
- Part of:
-
- Published online by Cambridge University Press:
- 09 January 2025, E15
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Research Article
High-density positron beam generation via Breit–Wheeler and trident processes using ultra-intense lasers
-
- Published online by Cambridge University Press:
- 09 January 2025, E14
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Enhanced ion heating using a TWA antenna in DEMO-like plasmas
- Part of:
-
- Published online by Cambridge University Press:
- 09 January 2025, E13
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Energetic bounds on gyrokinetic instabilities. Part 4. Bounce-averaged electrons
-
- Published online by Cambridge University Press:
- 10 January 2025, E12
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Energy flux decomposition in magnetohydrodynamic turbulence
-
- Published online by Cambridge University Press:
- 09 January 2025, E11
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Free electron laser in the magnetically dominated regime: simulations with the ONEDFEL code
-
- Published online by Cambridge University Press:
- 09 January 2025, E10
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Ultrafast dynamics of a spin-polarized electron plasma with magnetic ions
-
- Published online by Cambridge University Press:
- 07 January 2025, E9
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Acceleration of the particle-in-cell code Osiris with graphics processing units
-
- Published online by Cambridge University Press:
- 07 January 2025, E8
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Review Article
A review on the vortex and coherent structures in dusty plasma medium
-
- Published online by Cambridge University Press:
- 03 January 2025, E7
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Research Article
Self-organization of collisionless shocks: a ‘phase transition’ from a planar stationary profile to a rippled structure
-
- Published online by Cambridge University Press:
- 03 January 2025, E6
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Turbulent magnetic decay controlled by two conserved quantities
-
- Published online by Cambridge University Press:
- 03 January 2025, E5
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service
Quasilinear drift kinetic theory of alpha particle transport by neoclassical tearing modes
-
- Published online by Cambridge University Press:
- 03 January 2025, E4
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- NASA ADS Abstract Service