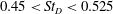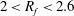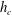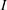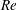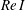The bifurcation and the blooming of jets have been numerically investigated at moderate Reynolds numbers. The study is motivated by a review article of Reynolds et al. (Annu. Rev. Fluid Mech., vol. 35, 2003, pp. 295–315) in which flow visualization images of jet blooming have been discussed, when the flow is subjected to inflow perturbations. Dual-mode perturbation, a combination of axisymmetric and helical excitations, has been used at the inflow plane to control the jet structures. In addition to the excitation frequency ratio, the effects of small-scale perturbation, excitation amplitude and initial momentum thickness have been examined. Results obtained at a Reynolds number of 2000 show that the number of branches formed in the blooming jet is strongly dependent on the excitation frequency ratio. For frequency ratios of 2, 2.5, 2.25, 2.4 and 2.22, the number of branches seen is 2, 5, 9, 12 and 20 respectively. In a blooming jet, the offset angle lies in the range 140°–180°. An equal number of branches is seen in the time-averaged flow field as well. The range of excitation frequency of the axisymmetric mode of perturbation is found to be 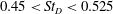 $0.45<\mathit{St}_{D}<0.525$, with an excitation frequency ratio range of
$0.45<\mathit{St}_{D}<0.525$, with an excitation frequency ratio range of 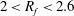 $2<R_{f}<2.6$, for which blooming jets are formed. The role of inlet shear layer thickness is less important as far as the blooming jet is concerned, while increasing excitation amplitude increases entrainment. Time-averaged data show that the blooming patterns persist in time, showing a substantial increase in spreading and entrainment.
$2<R_{f}<2.6$, for which blooming jets are formed. The role of inlet shear layer thickness is less important as far as the blooming jet is concerned, while increasing excitation amplitude increases entrainment. Time-averaged data show that the blooming patterns persist in time, showing a substantial increase in spreading and entrainment.
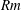 $Rm$
$Rm$