Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-23T09:11:11.809Z Has data issue: false hasContentIssue false
  • English
  • Français

Simulation of the blooming phenomenon in forced circular jets

Published online by Cambridge University Press:  26 October 2015

Trushar B. Gohil ,
Arun K. Saha  and
K. Muralidhar
Trushar B. Gohil
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Kanpur, Kanpur 208016, India
Arun K. Saha*
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Kanpur, Kanpur 208016, India
K. Muralidhar
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Kanpur, Kanpur 208016, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The bifurcation and the blooming of jets have been numerically investigated at moderate Reynolds numbers. The study is motivated by a review article of Reynolds et al. (Annu. Rev. Fluid Mech., vol. 35, 2003, pp. 295–315) in which flow visualization images of jet blooming have been discussed, when the flow is subjected to inflow perturbations. Dual-mode perturbation, a combination of axisymmetric and helical excitations, has been used at the inflow plane to control the jet structures. In addition to the excitation frequency ratio, the effects of small-scale perturbation, excitation amplitude and initial momentum thickness have been examined. Results obtained at a Reynolds number of 2000 show that the number of branches formed in the blooming jet is strongly dependent on the excitation frequency ratio. For frequency ratios of 2, 2.5, 2.25, 2.4 and 2.22, the number of branches seen is 2, 5, 9, 12 and 20 respectively. In a blooming jet, the offset angle lies in the range 140°–180°. An equal number of branches is seen in the time-averaged flow field as well. 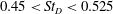The range of excitation frequency of the axisymmetric mode of perturbation is found to be $0.45<\mathit{St}_{D}<0.525$, with an excitation frequency ratio range of 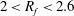$2<R_{f}<2.6$, for which blooming jets are formed. The role of inlet shear layer thickness is less important as far as the blooming jet is concerned, while increasing excitation amplitude increases entrainment. Time-averaged data show that the blooming patterns persist in time, showing a substantial increase in spreading and entrainment.

JFM classification

Flow Control: Flow Control Wakes/Jets: Jets Vortex Flows: Vortex dynamics
Type
Papers
Information
Journal of Fluid Mechanics , Volume 783 , 25 November 2015 , pp. 567 - 604
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Babu, P. C. & Mahesh, K. 2004 Upstream entrainment in numerical simulations of spatially evolving round jets. Phys. Fluids 16, 3699–3705.CrossRefGoogle Scholar
Cantwell, B. J. 1981 Organized motion in turbulent flow. Annu. Rev. Fluid Mech. 13, 457–515.Google Scholar
Cohen, J. & Wygnanski, I. 1987 The evolution of instabilities in the axisymmetric jet. Part 1 & Part 2. J. Fluid Mech. 176, 191–221.Google Scholar
Corke, T. C. & Kusek, S. M. 1993 Resonance in axisymmetric jets with controlled helical-mode input. J. Fluid Mech. 249, 307–336.Google Scholar
Crow, S. C. & Champagne, F. H. 1971 Orderly structure in jet turbulence. J. Fluid Mech. 48 (5), 547–591.Google Scholar
Danaila, I. & Boersma, J. 1998 Mode interaction in a forced homogeneous jet at low Reynolds numbers. Proceedings of the Summer Program 1998, Stanford University, USA. pp. 141–158. Center for Turbulent Research.Google Scholar
Danaila, I. & Boersma, B. J. 2000 Direct numerical simulation of bifurcating jets. Phys. Fluids 12 (5), 1255–1257.Google Scholar
Fornberg, B. 1988 Generation of finite difference formulas on arbitrarily spaced grids. Maths Comput. 51, 699–706.CrossRefGoogle Scholar
Gad-el-Hak, M. 1996 Modern developments in flow control. Appl. Mech. Rev. 49, 365–379.Google Scholar
Ginevsky, A. S., Vlasov, Ye. V. & Karavosov, R. K. 2004 Acoustic Control of Turbulent Jets. Springer.Google Scholar
Gohil, T. B.2011 Control of circular and square jets using large scale perturbations: a numerical study. Doctoral thesis, Indian Institute of Technology Kanpur, Kanpur.Google Scholar
Gohil, T. B., Saha, A. K.  & Muralidhar, K. 2010 Control of flow in forced jets: a comparison of round and square cross-sections. J. Vis. 13 (2), 141–149.Google Scholar
Gohil, T. B., Saha, A. K. & Muralidhar, K. 2011 Direct numerical simulation of a naturally evolving free circular jet. Trans. ASME J. Fluids Engng 133, 111203 (11 pages).CrossRefGoogle Scholar
Gohil, T. B., Saha, A. K. & Muralidhar, K. 2012 Numerical study of instability mechanisms in a circular jet at low Reynolds numbers. Comput. Fluids 64, 1–18.CrossRefGoogle Scholar
Gohil, T. B., Saha, A. K. & Muralidhar, K. 2013 Direct numerical simulation of forced circular jets: effect of varicose perturbation. Intl J. Heat Fluid Flow 44, 524–541.Google Scholar
Gohil, T. B., Saha, A. K. & Muralidhar, K. 2014 Large eddy simulation of a free circular jet. Trans. ASME J. Fluids Engng 136, 051205 (14 pages).Google Scholar
Gutmark, E. & Ho, C. M. 1983 Preferred modes and the spreading rates of jets. Phys. Fluids 26 (10), 2932–2938.CrossRefGoogle Scholar
Hajj, M. R., Miksad, R. W. & Powers, E. J. 1992 Sub-harmonic growth by parametric resonance. J. Fluid Mech. 236, 385–413.Google Scholar
Harlow, F. H. & Welch, J. E. 1966 Numerical study of large-amplitude free-surface motions. Phys. Fluids 9, 842–851.Google Scholar
Ho, C. M. & Huang, L. S. 1982 Sub-harmonic and vortex merging in mixing layers. J. Fluid Mech. 19, 443–473.Google Scholar
Ho, C. M. & Huerre, P. 1984 Perturbed free shear layers. Ann. Rev Fluid Mech. 16, 365–422.Google Scholar
Hunt, J. C. R., Wray, A. A. & Moin, P.1988 Eddies, stream, and convergence zones in turbulent flows. Rep. CTR-S88. Center For Turbulence Research, pp. 193–208.Google Scholar
Hussain, A. K. F. M. & Zaman, K. B. M. Q. 1980 Vortex pairing in a circular jet under controlled excitation. Part 2. Coherent structure dynamics. J. Fluid Mech. 103 (3), 493–544.CrossRefGoogle Scholar
Hussein, H. J., Capp, S. P. & George, W. K. 1994 Velocity measurements in a high-Reynolds-number, momentum-conserving, axisymmetric, turbulent jet. J. Fluid Mech. 258, 31–75.CrossRefGoogle Scholar
Kasagi, N. 1998 Progress in direct numerical simulation of turbulent transport and its control. Intl J. Heat Fluid Flow 19, 125–134.Google Scholar
Kelly, R. E. 1967 On the stability of an inviscid shear layer which is periodic in space and time. J. Fluid Mech. 27, 657–689.Google Scholar
Lee, M. & Reynolds, W. C. 1985a Bifurcating and blooming jets. Rep. TF-22. Thermosciences Division, Department of Mechanical Engineering, Stanford University, Stanford, CA.Google Scholar
Lee, M. & Reynolds, W. C. 1985b Bifurcating and blooming jets at high Reynolds number. 5th Symposium on Turbulent Shear Flows, New York. pp. 1.7–1.12.Google Scholar
Lepicovsky, J., Ahuja, K. K. & Burrin, R. H. 1985 Tone excited jets. Part 3. Flow measurements. J. Sound Vib. 102 (1), 71–91.Google Scholar
Long, T. A. & Petersen, R. A. 1990 Controlled interactions in a forced axisymmetric jet. Part 1. The distortion of the mean flow. J. Fluid Mech. 235, 37–55.Google Scholar
Longmire, E. K. & Duong, L. H. 1996 Bifurcating jets generated with stepped and sawtooth nozzles. Phys. Fluids 8, 978–992.Google Scholar
Michalke, A. & Hermann, G. 1982 On the inviscid instability of a circular jet with external flow. J. Fluid Mech. 114, 343–359.Google Scholar
Moin, P. & Bewley, T. 1994 Feedback control of turbulence. Appl. Mech Rev. 47 (6), Part 2. S3–S13.Google Scholar
Monkewitz, P. A. 1988 Sub-harmonic resonance, pairing, and shredding in the mixing layer. J. Fluid Mech. 188, 223–252.Google Scholar
Moore, C. J. 1977 The role of shear layer instability waves in jet exhaust noise. J. Fluid Mech. 80 (2), 321–367.Google Scholar
Ng, T. T. & Bradley, T. A. 1988 Effect of multi-frequency forcing on the near field development of a jet. AIAA J. 26 (10), 1201–1207.Google Scholar
Nikitopoulos, D. E. & Liu, J. T. C. 1987 Non-linear binary mode interactions in a developing mixing layer. J. Fluid Mech. 179, 345–370.Google Scholar
O’Neill, P., Soria, J. & Honnery, D. 2004 The stability of low Reynolds number round jets. Exp. Fluids 36, 473–483.Google Scholar
Orlanski, I. 1976 A simple boundary condition for unbounded flows. J. Comput. Phys. 21, 251–269.Google Scholar
Panchapakesan, N. R. & Lumley, J. L. 1993 Turbulence measurements in axisymmetric jets of air and helium. Part 1. Air jet. J. Fluid Mech. 246, 197–223.CrossRefGoogle Scholar
Parekh, D. E., Leonard, A. & Reynolds, W. C.1988 Bifurcating jets at high Reynolds numbers. Rep. TF-35. Department of Mechanical Engineering, Stanford University.Google Scholar
Pashereit, C. O., Oster, D., Long, T. A., Fiedler, H. E. & Wygnanski, I. 1992 Flow visualization of interactions among large coherent structures in axisymmetric jet. Exp. Fluids 12, 189–199.Google Scholar
Patnaik, P. C., Sherman, F. S. & Corcos, G. M. 1976 A numerical simulation of Kelvin–Helmholtz waves of finite amplitude. J. Fluid Mech. 73, 215–240.Google Scholar
Petersen, R. A. & Clough, R. C. 1992 The influence of higher harmonics on vortex pairing in an axisymmetric mixing layer. J. Fluid Mech. 239, 81–98.Google Scholar
Rai, M. M. & Moin, P. 1991 Direct simulations of turbulent flow using finite-difference schemes. J. Comput. Phys. 96, 15–53.Google Scholar
Reynolds, W. C., Parekh, D. E., Juvet, P. J. D. & Lee, M. J. D. 2003 Bifurcating and blooming jets. Annu. Rev. Fluid Mech. 35, 295–315.Google Scholar
Riley, J. J. & Metcalfe, R. W.1980 Direct numerical simulation of a perturbed, turbulent mixing layer. AIAA Paper No. 80-0274.Google Scholar
Robinson, K. S. 1991 Coherent motions in the turbulent boundary layer. Annu. Rev. Fluid Mech. 23, 601–639.Google Scholar
Rodi, W. 1975 A new method of analyzing hot-wire signals in highly turbulent flow and its evaluation in a round jet. DISA Inform. 17, 9–18.Google Scholar
da Silva, C. B. & Métais, O. 2002 Vortex control of bifurcating jets: a numerical study. Phys. Fluids 14, 3798.Google Scholar
Smith, B. L. & Glezer, A.1997 Vectoring and small-scale motions effected in free shear flows using synthetic jet actuators. AIAA Paper 97-0213.CrossRefGoogle Scholar
Suzuki, H., Kasagi, N. & Suzuki, Y.1999 Active control of an axisymmetric jet with an intelligent nozzle. 1st Symposium on Turbulent Shear Flow Phenomena, Santa Barbara, California, USA, pp. 665–670.Google Scholar
Suzuki, H., Kasagi, N. & Suzuki, Y. 2004 Active control of an axisymmetric jet with distributed electromagnetic flap actuators. Exp. Fluids 36, 498–509.Google Scholar
Urbin, G. & Métais, O. 1997 Large-eddy simulations of three-dimensional spatially-developing round jets. In Direct and Large-Eddy Simulations II (ed. Chollet, J. P., Voke, P. R. & Kleiser, L.). pp. 35–46. Kluwer.CrossRefGoogle Scholar
Wygnanski, I. & Fiedler, H. 1969 Some measurements in the self-preserving jet. J. Fluid Mech. 38 (3), 577–612.Google Scholar
Zang, Y. Q., Ho, C. M. & Monkewitz, P. A. 1985 The mixing layer forced by fundamental and subharmonic. In Laminar Turbulent Transition, IUTM Symposium, Novosibirsk (ed. Kozlov, V. V.), pp. 385–395. Springer.Google Scholar