If, in a large expanse of fluid such as air or water, an object that is heavier than the fluid displaced is released from rest, it descends in a manner that can depend in a complex way on its geometry and density (relative to that of the fluid), and on the fluid viscosity, which, as in other fluid contexts, remains important no matter how small this viscosity may be. A major numerical attack on this problem for the case in which the object is a thin circular disc is presented by Auguste, Magnaudet & Fabre (J. Fluid Mech., vol. 719, 2013, pp. 388–405).

The gravitationally driven flow of a dense fluid within a two-layered porous media is examined experimentally and theoretically. We find that in systems with two horizontal layers of differing permeability a competition between gravity driven flow and flow focusing along high-permeability routes can lead to two distinct flow regimes. When the lower layer is more permeable than the upper layer, gravity acts along high-permeability pathways and the flow is enhanced in the lower layer. Alternatively, when the upper layer is more permeable than the lower layer, we find that for a sufficiently small input flux the flow is confined to the lower layer. However, above a critical flux fluid preferentially spreads horizontally within the upper layer before ultimately draining back down into the lower layer. This later regime, in which the fluid overrides the low-permeability lower layer, is important because it enhances the mixing of the two fluids. We show that the critical flux which separates these two regimes can be characterized by a simple power law. Finally, we briefly discuss the relevance of this work to the geological sequestration of carbon dioxide and other industrial and natural flows in porous media.

The purpose of the present paper is to study the influence of wall echo on pressure fluctuations 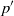${p}^{\prime } $, and on statistical correlations containing 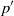${p}^{\prime } $, namely, redistribution 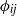${\phi }_{ij} $, pressure diffusion 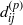${ d}_{ij}^{(p)} $ and velocity pressure-gradient ${\Pi }_{ij} $. We extend the usual analysis of turbulent correlations containing pressure fluctuations in wall-bounded direct numerical simulations (Kim, J. Fluid Mech., vol. 205, 1989, pp. 421–451), separating ${p}^{\prime } $ not only into rapid 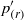${ p}_{(r)}^{\prime } $ and slow 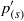${ p}_{(s)}^{\prime } $ parts (Chou, Q. Appl. Maths, vol. 3, 1945, pp. 38–54), but also further into volume (${ p}_{(r; \mathfrak{V})}^{\prime } $ and 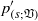${ p}_{(s; \mathfrak{V})}^{\prime } $) and surface (wall echo, 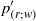${ p}_{(r; w)}^{\prime } $ and 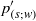${ p}_{(s; w)}^{\prime } $) terms. An algorithm, based on a Green’s function approach, is developed to compute the above splittings for various correlations containing pressure fluctuations (redistribution, pressure diffusion, velocity pressure-gradient), in fully developed turbulent plane channel flow. This exact analysis confirms previous results based on a method-of-images approximation (Manceau, Wang & Laurence, J. Fluid Mech., vol. 438, 2001, pp. 307–338) showing that, at the wall, 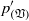${ p}_{(\mathfrak{V})}^{\prime } $ and 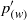${ p}_{(w)}^{\prime } $ are usually of the same sign and approximately equal. The above results are then used to study the contribution of each mechanism to the pressure correlations in low-Reynolds-number plane channel flow, and to discuss standard second-moment-closure modelling practices.

We investigate the route to dissipation in strongly stratified and rotating systems through high-resolution numerical simulations of the Boussinesq equations (BQs) and the primitive equations (PEs) in a triply periodic domain forced at large scales. By applying geostrophic scaling to the BQs and using the same horizontal length scale in defining the Rossby and the Froude numbers, $\mathit{Ro}$ and $\mathit{Fr}$, we show that the PEs can be obtained from the BQs by taking the limit ${\mathit{Fr}}^{2} / {\mathit{Ro}}^{2} \rightarrow 0$. When ${\mathit{Fr}}^{2} / {\mathit{Ro}}^{2} $ is small the difference between the results from the BQ and the PE simulations is shown to be small. For large rotation rates, quasi-geostrophic dynamics are recovered with a forward enstrophy cascade and an inverse energy cascade. As the rotation rate is reduced, a fraction of the energy starts to cascade towards smaller scales, leading to a shallowing of the horizontal spectra from ${ k}_{h}^{- 3} $ to ${ k}_{h}^{- 5/ 3} $ at the small-scale end. The vertical spectra show a similar transition as the horizontal spectra and we find that Charney isotropy is approximately valid also at larger wavenumbers than the transition wavenumber. The high resolutions employed allow us to capture both ranges within the same simulation. At the transition scale, kinetic energy in the rotational and in the horizontally divergent modes attain comparable values. The divergent energy is several orders of magnitude larger than the quasi-geostrophic divergent energy given by the $\Omega $-equation. The amount of energy cascading downscale is mainly controlled by the rotation rate, with a weaker dependence on the stratification. A larger degree of stratification favours a downscale energy cascade. For intermediate degrees of rotation and stratification, a constant energy flux and a constant enstrophy flux coexist within the same range of scales. In this range, the enstrophy flux is a result of triad interactions involving three geostrophic modes, while the energy flux is a result of triad interactions involving at least one ageostrophic mode, with a dominant contribution from interactions involving two ageostrophic and one geostrophic mode. Dividing the ageostrophic motions into two classes depending on the sign of the linear wave frequency, we show that the energy transfer is for the largest part supported by interactions within the same class, ruling out the wave–wave–vortex resonant triad interaction as a mean of the downscale energy transfer. The role of inertia-gravity waves is studied through analyses of time-frequency spectra of single Fourier modes. At large scales, distinct peaks at frequencies predicted for linear waves are observed, whereas at small scales no clear wave activity is observed. Triad interactions show a behaviour which is consistent with turbulent dynamics, with a large exchange of energy in triads with one small and two large comparable wavenumbers. The exchange of energy is mainly between the modes with two comparable wavenumbers.

The internal gravity wave (IGW) field emitted by a stably stratified, initially turbulent, wake of a towed sphere in a linearly stratified fluid is studied using fully nonlinear numerical simulations. A wide range of Reynolds numbers, $\mathit{Re}= UD/ \nu \in [5\times 1{0}^{3} , 1{0}^{5} ] $ and internal Froude numbers, $\mathit{Fr}= 2U/ (ND)\in [4, 16, 64] $ ($U$, $D$ are characteristic body velocity and length scales, and $N$ is the buoyancy frequency) is examined. At the higher $\mathit{Re}$ examined, secondary Kelvin–Helmholtz instabilities and the resulting turbulent events, directly linked to a prolonged non-equilibrium (NEQ) regime in wake evolution, are responsible for IGW emission that persists up to $Nt\approx 100$. In contrast, IGW emission at the lower $\mathit{Re}$ investigated does not continue beyond $Nt\approx 50$ for the three $\mathit{Fr}$ values considered. The horizontal wavelengths of the most energetic IGWs, obtained by continuous wavelet transforms, increase with $\mathit{Fr}$ and appear to be smaller at the higher $\mathit{Re}$, especially at late times. The initial value of these wavelengths is set by the wake height at the beginning of the NEQ regime. At the lower $\mathit{Re}$, consistent with a recently proposed model, the waves propagate over a narrow range of angles that minimize viscous decay along their path. At the higher $\mathit{Re}$, wave motion is much less affected by viscosity, at least initially, and early-time wave propagation angles extend over a broader range of values which are linked to increased efficiency in momentum extraction from the turbulent wake source.

We report an experimental study of the properties of the velocity boundary layer in turbulent Rayleigh–Bénard convection in a cylindrical cell. The measurements were made at Rayleigh numbers $\mathit{Ra}$ in the range $2. 4\times 1{0}^{8} \lt \mathit{Ra}\lt 5. 6\times 1{0}^{9} $ and were conducted with the convection cell tilted with an angle $\theta $ relative to gravity, at $\theta = 0. 5, 1. 0, 2. 0$ and $3. {4}^{\circ } $, respectively. The fluid was water with Prandtl number $\mathit{Pr}= 5. 3$. It is found that at small tilt angles ($\theta \leq {1}^{\circ } $), the measured viscous boundary layer thickness ${\delta }_{v} $ scales with the Reynolds number $\mathit{Re}$ with an exponent close to that for a Prandtl–Blasius (PB) laminar boundary layer, i.e. ${\delta }_{v} \sim {\mathit{Re}}^{- 0. 46\pm 0. 03} $. For larger tilt angles, the scaling exponent of ${\delta }_{v} $ with $\mathit{Re}$ decreases with $\theta $. The normalized mean horizontal velocity profiles measured at the same tilt angle but with different $\mathit{Ra}$ are found to have an invariant shape. However, for different tilt angles, the shape of the normalized profiles is different. It is also found that the Reynolds number $\mathit{Re}$ based on the maximum mean horizontal velocity scales with $\mathit{Ra}$ as $\mathit{Re}\sim {\mathit{Ra}}^{0. 43} $ and the Reynolds number ${\mathit{Re}}_{\sigma } $ based on the maximum root mean square velocity scales with $\mathit{Ra}$ as ${\mathit{Re}}_{\sigma } \sim {\mathit{Ra}}^{0. 55} $. Within the measurement resolution neither exponent depends on the tilt angle $\theta $. Several wall quantities are also measured directly and their dependencies on $\mathit{Re}$ are found to agree well with those predicted for a classical laminar boundary layer. These are the wall shear stress $\tau $ (${\sim }{\mathit{Re}}^{1. 46} $), the viscous sublayer ${\delta }_{w} $ (${\sim }{\mathit{Re}}^{0. 75} $), the friction velocity ${u}_{\tau } $ (${\sim }{\mathit{Re}}^{- 0. 86} $) and the skin friction coefficient ${c}_{f} $ (${\sim }{\mathit{Re}}^{- 0. 46} $). Again, all of these near-wall quantities do not exhibit a dependence on the tilt angle within the measurement resolution. We also examined the dynamical scaling method proposed by Zhou and Xia (Phys. Rev. Lett., vol. 104, 2010, p. 104301) and found that in both the laboratory and the dynamical frames the mean velocity profiles show deviations from the theoretical PB profile, with the deviations increasing with $\mathit{Ra}$. However, profiles obtained from dynamical scaling in general have better agreement with the theoretical profile. It is also found that the effectiveness of this method appears to be independent of $\mathit{Ra}$.

The dynamics of the deformation of a drop in axisymmetric compressional viscous flow is addressed through analytical and numerical analyses for a variety of capillary numbers, $\mathit{Ca}$, and viscosity ratios, $\lambda $. For low $Ca$, the drop is approximated by an oblate spheroid, and an analytical solution is obtained in terms of spheroidal harmonics; whereas, for the case of equal viscosities ($\lambda = 1$), the velocity field within and outside a drop of a given shape admits an integral representation, and steady shapes are found in the form of Chebyshev series. For arbitrary $Ca$ and $\lambda $, exact steady shapes are evaluated numerically via an integral equation. The critical $\mathit{Ca}$, below which a steady drop shape exists, is established for various $\lambda $. Remarkably, in contrast to the extensional flow case, critical steady shapes, being flat discs with rounded rims, have similar degrees of deformation ($D\sim 0. 75$) for all $\lambda $ studied. It is also shown that for almost the entire range of $\mathit{Ca}$ and $\lambda $, the steady shapes have accurate two-parameter approximations. The validity and implications of spheroidal and two-parameter shape approximations are examined in comparison to the exact steady shapes.

Clustering (or preferential concentration) of weakly inertial particles suspended in a homogeneous isotropic turbulent flow is driven primarily by the smallest eddies at the so-called Kolmogorov scale. In particle-laden large-eddy simulations (LES), these small scales are not resolved by the grid and hence their effect on both the resolved flow scales and the particle motion have to be modelled. In order to predict clustering in a particle-laden LES, it is crucial that the subgrid model for the particles captures the mechanism by which the subgrid scales affect the particle motion (Ray & Collins, J. Fluid Mech., vol. 680, 2011, pp. 488–510). In this paper, we describe novel satellite particle simulations (SPS), in which we study the clustering and relative velocity statistics of inertial particles at separation distances well below the Kolmogorov length scale. SPS is designed to isolate pairwise interactions of particles, and is therefore well suited for developing two-particle models. We show that the power-law dependence of the radial distribution function (RDF), a statistical measure of clustering, is predicted by the SPS in excellent agreement with direct numerical simulations (DNS) for Stokes numbers up to 3, implying that no explicit information from the inertial range is required to accurately describe particle clustering. This result further explains our successful prediction of the RDF power using the drift-diffusion model of Chun et al. (J. Fluid Mech., vol. 536, 2005, pp. 219–251) for $\mathit{St}\leq 0. 4$. We also consider the second-order longitudinal relative velocity structure function for the particles; we show that the SPS is able to capture its power-law exponent for $\mathit{St}\leq 0. 5$ and attribute the disagreement at larger $\mathit{St}$ to the effect of the larger scales of motion not captured by the SPS. Further, the SPS is able to capture the ‘caustic activation’ of the structure function at zero separation and predict the critical $\mathit{St}$ and rate of activation in agreement with the DNS (Salazar & Collins, J. Fluid. Mech., vol. 696, 2012, pp. 45–66). We show comparisons between filtered DNS and equivalently filtered SPS, and the findings are similar to the unfiltered case. Overall, SPS is an efficient and accurate computational tool for investigating particle pair dynamics at small separations, as well as an interesting platform for developing LES subgrid models designed to accurately reproduce particle clustering.

We investigate the problem of oscillatory flow of a homogeneous fluid with viscosity $\nu $ in a fluid-filled sphere of radius $a$ that rotates rapidly about a fixed axis with angular velocity ${\Omega }_{0} $ and that undergoes weak longitudinal libration with amplitude $\epsilon {\Omega }_{0} $ and frequency $\hat {\omega } {\Omega }_{0} $, where $\epsilon $ is the Poincaré number with $\epsilon \ll 1$ and $\hat {\omega } $ is dimensionless frequency with $0\lt \hat {\omega } \lt 2$. Three different methods are employed in this investigation: (i) asymptotic analysis at small Ekman numbers $E$ defined as $E= \nu / ({a}^{2} {\Omega }_{0} )$; (ii) linear numerical analysis using a spectral method; and (iii) nonlinear direct numerical simulation using a finite-element method. A satisfactory agreement among the three different sets of solutions is achieved when $E\leq 1{0}^{- 4} $. It is shown that the flow amplitude $\vert \boldsymbol{u}\vert $ is nearly independent of both the Ekman number $E$ and the libration frequency $\hat {\omega } $, always obeying the asymptotic scaling $\vert \boldsymbol{u}\vert = O(\epsilon )$ even though various spherical inertial modes are excited by longitudinal libration at different libration frequencies $\hat {\omega } $. Consequently, resonances do not occur in this system even when $\hat {\omega } $ is at the characteristic value of an inertial mode. It is also shown that the pressure difference along the axis of rotation is anomalous: this quantity reaches a sharp peak when $\hat {\omega } $ approaches a characteristic value. In contrast, the pressure difference measured at other places in the sphere, such as in the equatorial plane, and the volume-integrated kinetic energy are nearly independent of both the Ekman number $E$ and the libration frequency $\hat {\omega } $. Absence of resonances in a fluid-filled sphere forced by longitudinal libration is explained through the special properties of the analytical solution that satisfies the no-slip boundary condition and is valid for $E\ll 1$ and $\epsilon \ll 1$.

The physical structures of velocity are examined from a recent direct numerical simulation of fully developed incompressible turbulent pipe flow (Wu, Baltzer & Adrian, J. Fluid Mech., vol. 698, 2012, pp. 235–281) at a Reynolds number of ${\mathit{Re}}_{D} = 24\hspace{0.167em} 580$ (based on bulk velocity) and a Kármán number of ${R}^{+ } = 685$. In that work, the periodic domain length of $30$ pipe radii $R$ was found to be sufficient to examine long motions of negative streamwise velocity fluctuation that are commonly observed in wall-bounded turbulent flows and correspond to the large fractions of energy present at very long streamwise wavelengths (${\geq }3R$). In this paper we study how long motions are composed of smaller motions. We characterize the spatial arrangements of very large-scale motions (VLSMs) extending through the logarithmic layer and above, and we find that they possess dominant helix angles (azimuthal inclinations relative to streamwise) that are revealed by two- and three-dimensional two-point spatial correlations of velocity. The correlations also reveal that the shorter, large-scale motions (LSMs) that concatenate to comprise the VLSMs are themselves more streamwise aligned. We show that the largest VLSMs possess a form similar to roll cells centred above the logarithmic layer and that they appear to play an important role in organizing the flow, while themselves contributing only a minor fraction of the flow turbulent kinetic energy. The roll cell motions play an important role with the smaller scales of motion that are necessary to create the strong streamwise streaks of low-velocity fluctuation that characterize the flow.

Flapping wings often feature a leading-edge vortex (LEV) that is thought to enhance the lift generated by the wing. Here the lift on a wing featuring a leading-edge vortex is considered by performing experiments on a translating flat-plate aerofoil that is accelerated from rest in a water towing tank at a fixed angle of attack of 15°. The unsteady flow is investigated with dye flow visualization, particle image velocimetry (PIV) and force measurements. Leading- and trailing-edge vortex circulation and position are calculated directly from the velocity vectors obtained using PIV. In order to determine the most appropriate value of bound circulation, a two-dimensional potential flow model is employed and flow fields are calculated for a range of values of bound circulation. In this way, the value of bound circulation is selected to give the best fit between the experimental velocity field and the potential flow field. Early in the trajectory, the value of bound circulation calculated using this potential flow method is in accordance with Kelvin’s circulation theorem, but differs from the values predicted by Wagner’s growth of bound circulation and the Kutta condition. Later the Kutta condition is established but the bound circulation remains small; most of the circulation is contained instead in the LEVs. The growth of wake circulation can be approximated by Wagner’s circulation curve. Superimposing the non-circulatory lift, approximated from the potential flow model, and Wagner’s lift curve gives a first-order approximation of the measured lift. Lift is generated by inertial effects and the slow buildup of circulation, which is contained in shed vortices rather than bound circulation.

Snow avalanches and other hazardous geophysical granular flows, such as debris flows, lahars and pyroclastic flows, often impact on obstacles as they flow down a slope, generating rapid changes in the flow height and velocity in their vicinity. It is important to understand how a granular material flows around such obstacles to improve the design of deflecting and catching dams, and to correctly interpret field observations. In this paper small-scale experiments and numerical simulations are used to investigate the supercritical gravity-driven free-surface flow of a granular avalanche around a circular cylinder. Our experiments show that a very sharp bow shock wave and a stagnation point are generated in front of the cylinder. The shock standoff distance is accurately reproduced by shock-capturing numerical simulations and is approximately equal to the reciprocal of the Froude number, consistent with previous approximate results for shallow-water flows. As the grains move around the cylinder the flow expands and the pressure gradients rapidly accelerate the particles up to supercritical speeds again. The internal pressure is not strong enough to immediately push the grains into the space behind the cylinder and instead a grain-free region, or granular vacuum, forms on the lee side. For moderate upstream Froude numbers and slope inclinations, the granular vacuum closes up rapidly to form a triangular region, but on steeper slopes both experiments and numerical simulations show that the pinch-off distance moves far downstream.

We consider the flow of a viscous liquid along an unknown topography. A new strategy is presented to reconstruct the topography and the free surface shape from one component of the free surface velocity only. In contrast to the classical approach in inverse problems based on optimization theory we derive an ordinary differential equation which can be solved directly to obtain the inverse solution. This is achieved by averaging the Navier–Stokes equation and coupling the function parameterizing the flow domain with the free surface velocity. Even though we consider nonlinear systems including inertia and surface tension, the inverse problem can be solved analytically with a Fourier series approach. We test our method on a variety of benchmark problems and show that the analytical solution can be applied to reconstruct the flow domain from noisy input data. It is also demonstrated that the asymptotic approach agrees very well with numerical results of the Navier–Stokes equation. The results are finally confirmed with an experimental study where we measure the free surface velocity for the film flow over a trench and compare the reconstructed topography with the measured one.

We study the occurrence and dynamics of rogue waves in three-dimensional deep water using phase-resolved numerical simulations based on a high-order spectral (HOS) method. We obtain a large ensemble of nonlinear wave-field simulations ($M= 3$ in HOS method), initialized by spectral parameters over a broad range, from which nonlinear wave statistics and rogue wave occurrence are investigated. The HOS results are compared to those from the broad-band modified nonlinear Schrödinger (BMNLS) equations. Our results show that for (initially) narrow-band and narrow directional spreading wave fields, modulational instability develops, resulting in non-Gaussian statistics and a probability of rogue wave occurrence that is an order of magnitude higher than linear theory prediction. For longer times, the evolution becomes quasi-stationary with non-Gaussian statistics, a result not predicted by the BMNLS equations (without consideration of dissipation). When waves spread broadly in frequency and direction, the modulational instability effect is reduced, and the statistics and rogue wave probability are qualitatively similar to those from linear theory. To account for the effects of directional spreading on modulational instability, we propose a new modified Benjamin–Feir index for effectively predicting rogue wave occurrence in directional seas. For short-crested seas, the probability of rogue waves based on number frequency is imprecise and problematic. We introduce an area-based probability, which is well defined and convergent for all directional spreading. Based on a large catalogue of simulated rogue wave events, we analyse their geometry using proper orthogonal decomposition (POD). We find that rogue wave profiles containing a single wave can generally be described by a small number of POD modes.

We assess the extent to which local isotropy (LI) holds in a wake flow for different initial conditions, which may be geometrical (the shape of the bluff body which creates the wake) and hydrodynamical (the Reynolds number), as a function of the dynamical effects of the large-scale forcing (the mean strain, $ \overline{S} $, combined with the strain induced by the coherent motion, $\tilde {S} $). LI is appraised through either classical kinematic tests or phenomenological approaches. In this respect, we reanalyse existing LI criteria and formulate a new isotropy criterion based on the ratio between the turbulence strain intensity and the total strain ($ \overline{S} + \tilde {S} $). These criteria involve either time-averaged or phase-averaged quantities, thus providing a deeper insight into the dynamical aspect of these flows. They are tested using hot wire data in the intermediate wake of five types of obstacles (a circular cylinder, a square cylinder, a screen cylinder, a normal plate and a screen strip). We show that in the presence of an organized motion, isotropy is not an adequate assumption for the large scales but may be satisfied over a range of scales extending from the smallest dissipative scale up to a scale which depends on the total strain rate that characterizes the flow. The local value of this scale depends on the particular nature of the wake and the phase of the coherent motion. The square cylinder wake is the closest to isotropy whereas the least locally isotropic flow is the screen strip wake. For locations away from the axis, the study is restricted to the circular cylinder only and reveals that LI holds at scales smaller than those that apply at the wake centreline. Arguments based on self-similarity show that in the far wake, the strength of the coherent motion decays at the same rate as that of the turbulent motion. This implies the persistence of the same degree of anisotropy far downstream, independently of the scale at which anisotropy is tested.

The autoignition of an axisymmetric nitrogen-diluted hydrogen plume in a turbulent coflowing stream of high-temperature air was investigated in a laboratory-scale set-up using three-dimensional numerical simulations with detailed chemistry and transport. The plume was formed by releasing the fuel from an injector with bulk velocity equal to that of the surrounding air coflow. In the ‘random spots’ regime, autoignition appeared randomly in space and time in the form of scattered localized spots from which post-ignition flamelets propagated outwards in the presence of strong advection. Autoignition spots were found to occur at a favourable mixture fraction close to the most reactive mixture fraction calculated a priori from considerations of homogeneous mixtures based on inert mixing of the fuel and oxidizer streams. The value of the favourable mixture fraction evolved in the domain subject to the effect of the scalar dissipation rate. The hydroperoxyl radical appeared as a precursor to the build-up of the radical pool and the ensuing thermal runaway at the autoignition spots. Subsequently, flamelets propagated in all directions with complex dynamics, without anchoring or forming a continuous flame sheet. These observations, as well as the frequency of and scatter in appearance of the spots, are in good agreement with experiments in a similar set-up. In agreement with experimental observations, an increase in turbulence intensity resulted in a downstream shift of autoignition. An attempt is made to understand the key processes that control the mean axial and radial locations of the spots, and are responsible for the observed scatter. The advection of the most reactive mixture through the domain, and hence the history of evolution of the developing radical pools were considered to this effect.

The flow over a flat plate at a Reynolds number of 750 is numerically investigated via fine large-eddy simulation (LES), first at normal ($90\textdegree $) and then at oblique ($45\textdegree $) incidence flow direction with a uniform steady inlet. The results are in complete agreement with the direct numerical simulation (DNS) and experimental data, thereby serving as a validation for the present simulations. For the normal ($90\textdegree $) uniform inflow case, coherent vortices are alternately shed from both leading edges of the plate, whereas for the oblique ($45\textdegree $) uniform inflow case the vortices shed from the two sides of the plate interact strongly resulting in a quasi-periodic force response. The normal flat plate is then analysed with an incident gust signal with varying amplitude and time period. For these incident coherent gust cases, a reference test case with variable coherent inlet is first studied and the results are compared to a steady inlet simulation, with a detailed analysis of the flow behaviour and the wake response under the incident gust. Finally, the flat plate response to 16 different gust profiles is studied. A transient drag reconstruction for these incident coherent gust cases is then presented based on a frequency-dependent transfer function and phase spectrum analysis.

Linear stability analysis and direct numerical simulations are conducted to analyse mixed convection in a liquid metal flow in a horizontal pipe with imposed transverse magnetic field. The pipe walls are electrically insulated and subject to constant flux heating in the lower half. The results reveal the nature of anomalous temperature fluctuations detected in earlier experiments. It is found that, at the magnetic field strength far exceeding the laminarization threshold, the natural convection develops in the form of coherent quasi-two-dimensional rolls aligned with the magnetic field. Transport of the rolls by the mean flow causes high-amplitude, low-frequency fluctuations of temperature.

We present a mathematical model and corresponding series of microfluidic experiments examining the flow of a viscous fluid past an elastic fibre in a three-dimensional channel. The fibre’s axis lies perpendicular to the direction of flow and its base is clamped to one wall of the channel; the sidewalls of the channel are close to the fibre, confining the flow. Experiments show that there is a linear relationship between deflection and flow rate for highly confined fibres at low flow rates, which inspires an asymptotic treatment of the problem in this regime. The three-dimensional problem is reduced to a two-dimensional model, consisting of Hele-Shaw flow past a barrier, with boundary conditions at the barrier that allow for the effects of flexibility and three-dimensional leakage. The analysis yields insight into the competing effects of flexion and leakage, and an analytical solution is derived for the leading-order pressure field corresponding to a slit that partially blocks a two-dimensional channel. The predictions of our model show favourable agreement with experimental results, allowing measurement of the fibre’s elasticity and the flow rate in the channel.

As one of the most important force production mechanisms of swimming and flying animals, the fluid dynamics of flapping has been intensively studied. However, these efforts have been mainly directed toward animals in forward motion or locomotive appendages undergoing linear translation. Here we seek to complement the existing knowledge of the flapping mechanism by studying angularly reciprocating flat plates without a free stream velocity, under a so-called ‘bollard pull’ condition. We visualize the flow field around the flat plate to find that two independent vortical structures are formed per half-cycle, resulting in the separation of two distinct vortex pairs at sharp edges rather than a single vortex loop which is typical of a starting–stopping vortex paradigm in flows with free streams. Based on our observations, we derive a scaling law to predict the thrust of the flapping plate; this is the first experimentally validated theoretical model for the thrust of angularly reciprocating plates without a prescribed background flow.