Let 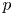p$p$ be a rational prime, let 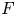F$F$ denote a finite, unramified extension of 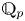{{\mathbb {Q}}}_p${{\mathbb {Q}}}_p$, let 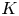K$K$ be the maximal unramified extension of 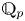{{\mathbb {Q}}}_p${{\mathbb {Q}}}_p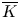$, {{\overline {K}}}${{\overline {K}}}$ some fixed algebraic closure of 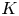K$K$, and 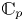{{\mathbb {C}}}_p${{\mathbb {C}}}_p$ be the completion of 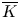{{\overline {K}}}${{\overline {K}}}$. Let 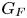G_F$G_F$ be the absolute Galois group of 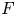F$F$. Let 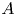A$A$ be an abelian variety defined over 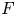F$F$, with good reduction. Classically, the Fontaine integral was seen as a Hodge–Tate comparison morphism, i.e. as a map 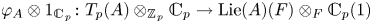\varphi _{A} \otimes 1_{{{\mathbb {C}}}_p}\colon T_p(A)\otimes _{{{\mathbb {Z}}}_p}{{\mathbb {C}}}_p\to \operatorname {Lie}(A)(F)\otimes _F{{\mathbb {C}}}_p(1)$\varphi _{A} \otimes 1_{{{\mathbb {C}}}_p}\colon T_p(A)\otimes _{{{\mathbb {Z}}}_p}{{\mathbb {C}}}_p\to \operatorname {Lie}(A)(F)\otimes _F{{\mathbb {C}}}_p(1)$, and as such it is surjective and has a large kernel. This paper starts with the observation that if we do not tensor T_p(A)$T_p(A)$ with 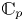{{\mathbb {C}}}_p${{\mathbb {C}}}_p$, then the Fontaine integral is often injective. In particular, it is proved that if T_p(A)^{G_K} = 0$T_p(A)^{G_K} = 0$, then 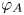\varphi _A$\varphi _A$ is injective. As an application, we extend the Fontaine integral to a perfectoid like universal cover of A$A$ and show that if 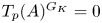T_p(A)^{G_K} = 0$T_p(A)^{G_K} = 0$, then 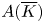A(\overline {K})$A(\overline {K})$ has a type of 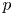p$p$-adic uniformization, which resembles the classical complex uniformization.

The purpose of this paper is to extend the explicit geometric evaluation of semisimple orbital integrals for smooth kernels for the Casimir operator obtained by the first author to the case of kernels for arbitrary elements in the center of the enveloping algebra.

We present an \ell$\ell$-adic trace formula for saturated and admissible dg-categories over a base monoidal differential graded (dg)-category. Moreover, we prove Künneth formulas for dg-category of singularities and for inertia-invariant vanishing cycles. As an application, we prove a categorical version of Bloch's conductor conjecture (originally stated by Spencer Bloch in 1985), under the additional hypothesis that the monodromy action of the inertia group is unipotent.

We show that the completed Hecke algebra of p$p$-adic modular forms is isomorphic to the completed Hecke algebra of continuous p$p$-adic automorphic forms for the units of the quaternion algebra ramified at p$p$ and \infty$\infty$. This gives an affirmative answer to a question posed by Serre in a 1987 letter to Tate. The proof is geometric, and lifts a mod p$p$ argument due to Serre: we evaluate modular forms by identifying a quaternionic double-coset with a fiber of the Hodge–Tate period map, and extend functions off of the double-coset using fake Hasse invariants. In particular, this gives a new proof, independent of the classical Jacquet–Langlands correspondence, that Galois representations can be attached to classical and p$p$-adic quaternionic eigenforms.

We study horizontal semistable and horizontal de Rham representations of the absolute Galois group of a certain smooth affinoid over a p$p$-adic field. In particular, we prove that a horizontal de Rham representation becomes horizontal semistable after a finite extension of the base field. As an application, we show that every de Rham local system on a smooth rigid analytic variety becomes horizontal semistable étale locally around every classical point. We also discuss potentially crystalline loci of de Rham local systems and cohomologically potentially good reduction loci of smooth proper morphisms.

We propose a variation of the classical Hilbert scheme of points, the double nested Hilbert scheme of points, which parametrizes flags of zero-dimensional subschemes whose nesting is dictated by a Young diagram. Over a smooth quasi-projective curve, we compute the generating series of topological Euler characteristic of these spaces, by exploiting the combinatorics of reversed plane partitions. Moreover, we realize this moduli space as the zero locus of a section of a vector bundle over a smooth ambient space, which therefore admits a virtual fundamental class. We apply this construction to the stable pair theory of a local curve, that is the total space of the direct sum of two line bundles over a curve. We show that the invariants localize to virtual intersection numbers on double nested Hilbert scheme of points on the curve, and that the localized contributions to the invariants are controlled by three universal series for every Young diagram, which can be explicitly determined after the anti-diagonal restriction of the equivariant parameters. Under the anti-diagonal restriction, the invariants are matched with the Gromov–Witten invariants of local curves of Bryan–Pandharipande, as predicted by the Maulik–Nekrasov–Okounkov–Pandharipande (MNOP) correspondence. Finally, we discuss K$K$-theoretic refinements à la Nekrasov–Okounkov.

We determine the local deformation rings of sufficiently generic mod l$l$ representations of the Galois group of a p$p$-adic field, when l \neq p$l \neq p$, relating them to the space of q$q$-power-stable semisimple conjugacy classes in the dual group. As a consequence, we give a local proof of the l \neq p$l \neq p$ Breuil–Mézard conjecture of the author, in the tame case.

In this paper we compute the topological Hochschild homology of quotients of discrete valuation rings (DVRs). Along the way we give a short argument for Bökstedt periodicity and generalizations over various other bases. Our strategy also gives a very efficient way to redo the computations of \operatorname {THH}$\operatorname {THH}$ (respectively, logarithmic \operatorname {THH}$\operatorname {THH}$) of complete DVRs originally due to Lindenstrauss and Madsen (respectively, Hesselholt and Madsen).

We prove a formula, which, given a principally polarized abelian variety (A,\lambda )$(A,\lambda )$ over the field of algebraic numbers, relates the stable Faltings height of A$A$ with the Néron–Tate height of a symmetric theta divisor on A$A$. Our formula completes earlier results due to Bost, Hindry, Autissier and Wagener. The local non-archimedean terms in our formula can be expressed as the tropical moments of the tropicalizations of (A,\lambda )$(A,\lambda )$.

A famous problem in birational geometry is to determine when the birational automorphism group of a Fano variety is finite. The Noether–Fano method has been the main approach to this problem. The purpose of this paper is to give a new approach to the problem by showing that in every positive characteristic, there are Fano varieties of arbitrarily large index with finite (or even trivial) birational automorphism group. To do this, we prove that these varieties admit ample and birationally equivariant line bundles. Our result applies the differential forms that Kollár produces on p$p$-cyclic covers in characteristic p > 0$p > 0$.

Crofton formulas on simply connected Riemannian space forms allow the volumes, or more generally the Lipschitz–Killing curvature integrals of a submanifold with corners, to be computed by integrating the Euler characteristic of its intersection with all geodesic submanifolds. We develop a framework of Crofton formulas with distributions replacing measures, which has in its core Alesker's Radon transform on valuations. We then apply this framework, and our recent Hadwiger-type classification, to compute explicit Crofton formulas for all isometry-invariant valuations on all pseudospheres, pseudo-Euclidean and pseudohyperbolic spaces. We find that, in essence, a single measure which depends analytically on the metric, gives rise to all those Crofton formulas through its distributional boundary values at parts of the boundary corresponding to the different indefinite signatures. In particular, the Crofton formulas we obtain are formally independent of signature.

The Hanna Neumann conjecture is a statement about the rank of the intersection of two finitely generated subgroups of a free group. The conjecture was posed by Hanna Neumann in 1957. In 2011, a strengthened version of the conjecture was proved independently by Joel Friedman and by Igor Mineyev. In this paper we show that the strengthened Hanna Neumann conjecture holds not only in free groups but also in non-solvable surface groups. In addition, we show that a retract in a free group and in a surface group is inert. This implies the Dicks–Ventura inertia conjecture for free and surface groups.

We construct the logarithmic and tropical Picard groups of a family of logarithmic curves and realize the latter as the quotient of the former by the algebraic Jacobian. We show that the logarithmic Jacobian is a proper family of logarithmic abelian varieties over the moduli space of Deligne–Mumford stable curves, but does not possess an underlying algebraic stack. However, the logarithmic Picard group does have logarithmic modifications that are representable by logarithmic schemes, all of which are obtained by pullback from subdivisions of the tropical Picard group.

We formulate a version of the integral Hodge conjecture for categories, prove the conjecture for two-dimensional Calabi–Yau categories which are suitably deformation equivalent to the derived category of a K3 or abelian surface, and use this to deduce cases of the usual integral Hodge conjecture for varieties. Along the way, we prove a version of the variational integral Hodge conjecture for families of two-dimensional Calabi–Yau categories, as well as a general smoothness result for relative moduli spaces of objects in such families. Our machinery also has applications to the structure of intermediate Jacobians, such as a criterion in terms of derived categories for when they split as a sum of Jacobians of curves.

A celebrated result by Orlov states that any fully faithful exact functor between the bounded derived categories of coherent sheaves on smooth projective varieties is of geometric origin, i.e. it is a Fourier–Mukai functor. In this paper we prove that any smooth projective variety of dimension \ge 3$\ge 3$ equipped with a tilting bundle can serve as the source variety of a non-Fourier–Mukai functor between smooth projective schemes.

We establish central limit theorems for an action of a group G$G$ on a hyperbolic space X$X$ with respect to the counting measure on a Cayley graph of G$G$. Our techniques allow us to remove the usual assumptions of properness and smoothness of the space, or cocompactness of the action. We provide several applications which require our general framework, including to lengths of geodesics in geometrically finite manifolds and to intersection numbers with submanifolds.

We prove the flow tree formula conjectured by Alexandrov and Pioline, which computes Donaldson–Thomas invariants of quivers with potentials in terms of a smaller set of attractor invariants. This result is obtained as a particular case of a more general flow tree formula reconstructing a consistent scattering diagram from its initial walls.

We show that every orbispace satisfying certain mild hypotheses has ‘enough’ vector bundles. It follows that the K$K$-theory of finite rank vector bundles on such orbispaces is a cohomology theory. Global presentation results for smooth orbifolds and derived smooth orbifolds also follow.

In this paper we construct an action of the affine Hecke category (in its ‘Soergel bimodules’ incarnation) on the principal block of representations of a simply connected semisimple algebraic group over an algebraically closed field of characteristic bigger than the Coxeter number. This confirms a conjecture of G. Williamson and the second author, and provides a new proof of the tilting character formula in terms of antispherical p$p$-Kazhdan–Lusztig polynomials.