Let α1, …, αm be distinct complex numbers and τ(1), …, τ(m) be non-negative integers. We obtain conditions under which the functions
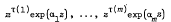
form a perfect system, that is, for every set ρ(1), …, ρ(m) of non-negative integers, there are polynomials a1 (z), …, am (z) with respective degrees exactly ρ(1)−1, …, ρ(m)−1, such that the function
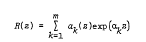
has a zero of order at least ρ(1) + … + ρ(m)−1 at the origin. Moreover, subject to the evaluation of certain determinants, we give explicit formulae for the approximating polynomials a1 (z), …, am (z).