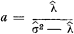Most cited
This page lists all time most cited articles for this title. Please use the publication date filters on the left if you would like to restrict this list to recently published content, for example to articles published in the last three years. The number of times each article was cited is displayed to the right of its title and can be clicked to access a list of all titles this article has been cited by.
- Cited by 3
The propensity to cause accidents*
-
- Published online by Cambridge University Press:
- 29 August 2014, pp. 154-164
-
- Article
-
- You have access
- Export citation
- Cited by 3
A Loglinear Lagrangian Poisson Model
-
- Published online by Cambridge University Press:
- 29 August 2014, pp. 123-129
-
- Article
-
- You have access
- Export citation
- Cited by 3
Premium Calculation for Fat-tailed Risk
-
- Published online by Cambridge University Press:
- 17 April 2015, pp. 163-188
-
- Article
-
- You have access
- Export citation
- Cited by 3
What is a Sports Car?
-
- Published online by Cambridge University Press:
- 29 August 2014, pp. 175-187
-
- Article
-
- You have access
- Export citation
- Cited by 3
Telematics combined actuarial neural networks for cross-sectional and longitudinal claim count data
-
- Published online by Cambridge University Press:
- 14 February 2024, pp. 239-262
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 3
Integration of traditional and telematics data for efficient insurance claims prediction
-
- Published online by Cambridge University Press:
- 15 February 2024, pp. 263-279
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
- Cited by 3
Balancing International Insurance Portfolios and Exchange Risks*
-
- Published online by Cambridge University Press:
- 29 August 2014, pp. 283-294
-
- Article
-
- You have access
- Export citation
- Cited by 3
GEOGRAPHICAL DIVERSIFICATION AND LONGEVITY RISK MITIGATION IN ANNUITY PORTFOLIOS
-
- Published online by Cambridge University Press:
- 28 April 2021, pp. 375-410
-
- Article
-
- You have access
- Open access
- Export citation
- Cited by 2
CHAIN-LADDER METHOD AND MIDYEAR LOSS RESERVING
-
- Published online by Cambridge University Press:
- 28 March 2017, pp. 3-24
-
- Article
- Export citation
- Cited by 2
Continuous Monitoring: Does Credit Risk Vanish?1
-
- Published online by Cambridge University Press:
- 09 August 2013, pp. 577-589
-
- Article
- Export citation
- Cited by 2
Extension of the Capital Asset Pricing Model to Non-normal Dependence Structures
-
- Published online by Cambridge University Press:
- 17 April 2015, pp. 35-52
-
- Article
-
- You have access
- Export citation
- Cited by 2
R. E. Beard: 11th January 1911–7th November 1983
-
- Published online by Cambridge University Press:
- 29 August 2014, pp. 103-104
-
- Article
-
- You have access
- Export citation
- Cited by 2
A NEW INFERENCE STRATEGY FOR GENERAL POPULATION MORTALITY TABLES
- Part of:
-
- Published online by Cambridge University Press:
- 17 April 2020, pp. 325-356
-
- Article
- Export citation
- Cited by 2
Testing Goodness-of-Fit of an Estimated Run-Off Triangle
-
- Published online by Cambridge University Press:
- 29 August 2014, pp. 78-86
-
- Article
-
- You have access
- Export citation
- Cited by 2
The ruin probability in a special case
-
- Published online by Cambridge University Press:
- 29 August 2014, pp. 66-68
-
- Article
-
- You have access
- Export citation
- Cited by 2
M. J. Goovaerts and W. J. Hoogstad (1987). Credibility Theory. Surveys of Actuarial Studies 4. Nationale-Nederlanden N. V., Rotterdam. 114 pages + 1 diskette.
-
- Published online by Cambridge University Press:
- 29 August 2014, pp. 197-199
-
- Article
-
- You have access
- Export citation
- Cited by 2
ON THE EVALUATION OF MULTIVARIATE COMPOUND DISTRIBUTIONS WITH CONTINUOUS SEVERITY DISTRIBUTIONS AND SARMANOV'S COUNTING DISTRIBUTION
-
- Published online by Cambridge University Press:
- 17 January 2018, pp. 841-870
-
- Article
- Export citation
- Cited by 2
Utilisation pratique de la methode de simulation dans l'assurance „non life”
-
- Published online by Cambridge University Press:
- 29 August 2014, pp. 87-117
-
- Article
-
- You have access
- Export citation
- Cited by 2
OPTIMAL REINSURANCE DESIGN WITH DISTORTION RISK MEASURES AND ASYMMETRIC INFORMATION
-
- Published online by Cambridge University Press:
- 29 March 2021, pp. 607-629
-
- Article
- Export citation
- Cited by 2
Optimal Premium Plans for Reinsurance with Reinstatements
-
- Published online by Cambridge University Press:
- 17 April 2015, pp. 299-313
-
- Article
-
- You have access
- Export citation

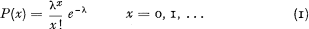
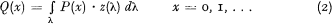
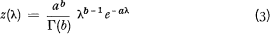


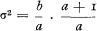
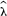 and the observed variance
and the observed variance  it is possible to determine
it is possible to determine