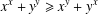Skip to main content Accessibility help

We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.

Close cookie message
×
  1. Home
  2. Search

Refine search

Refine search

Access:
Content type:
Publication date:
Apply   From and To filters
Subject: Show more
Journals Show more
Publishers: Show more
Societies Show more
Series: Show more
Collections: Show more

Actions for selected content:

Select all | Deselect all
  • View selected items
  • Export citations
  • Download PDF (zip)
  • Save to Kindle
  • Save to Dropbox
  • Save to Google Drive

Save Search

You can save your searches here and later view and run them again in "My saved searches".

Please provide a title, maximum of 40 characters.
Cancel
×

2 results

  • NEW ALGEBRAIC-TRIGONOMETRIC INEQUALITIES OF LAUB–ILANI TYPE

  • Part of
  • AHMET YAŞAR ÖZBAN
  • Journal:
    Bulletin of the Australian Mathematical Society / Volume 96 / Issue 1 / August 2017
    Published online by Cambridge University Press:
    02 March 2017, pp. 87-97
    Print publication:
    August 2017
    • Article
      • You have access
    • PDF
    • Export citation

  • The Laub–Ilani inequality [‘A subtle inequality’, Amer. Math. Monthly97 (1990), 65–67] states that $x^{x}+y^{y}\geqslant x^{y}+y^{x}$ for nonnegative real numbers $x,y$. We introduce and prove new trigonometric and algebraic-trigonometric inequalities of Laub–Ilani type and propose some conjectural algebraic-trigonometric inequalities of similar forms.

  • TRACE INEQUALITIES AND A QUESTION OF BOURIN

  • Part of
  • SAJA HAYAJNEH, FUAD KITTANEH
  • Journal:
    Bulletin of the Australian Mathematical Society / Volume 88 / Issue 3 / December 2013
    Published online by Cambridge University Press:
    25 January 2013, pp. 384-389
    Print publication:
    December 2013
    • Article
      • You have access
    • PDF
    • Export citation

  • We give an affirmative answer to one of the questions posed by Bourin regarding a special type of inequality referred to as subadditivity inequalities in the case of the Hilbert–Schmidt and the trace norms. We formulate the solution for arbitrary commuting positive operators, and we conjecture that it is true for all unitarily invariant norms and all commuting positive operators. New related trace inequalities are also presented.