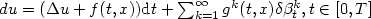Research Article
Estimating a discrete distribution via histogram selection
-
- Published online by Cambridge University Press:
- 22 February 2011, pp. 1-29
-
- Article
- Export citation
A new stochastic restricted biased estimator under heteroscedastic or correlated error
-
- Published online by Cambridge University Press:
- 22 February 2011, pp. 30-40
-
- Article
- Export citation
A non asymptotic penalized criterion for Gaussian mixture model selection
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 41-68
-
- Article
- Export citation
Local Asymptotic Normality Property for Lacunar Wavelet Series multifractal model*
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 69-82
-
- Article
- Export citation
On the large deviations of a class of modulated additiveprocesses
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 83-109
-
- Article
- Export citation
Lp-theory for the stochastic heat equationwith infinite-dimensional fractional noise*
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 110-138
-
- Article
- Export citation
Expansions for the distribution of M-estimates with applications to the Multi-Tone problem
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 139-167
-
- Article
- Export citation
Cramér type moderate deviations for Studentized U-statistics******
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 168-179
-
- Article
- Export citation
SURE shrinkage of Gaussian paths and signal identification*
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 180-196
-
- Article
- Export citation
Penalized nonparametric drift estimationfor a continuously observed one-dimensional diffusion process
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 197-216
-
- Article
- Export citation
Large deviations for directed percolationon a thin rectangle
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 217-232
-
- Article
- Export citation
Simulation and approximationof Lévy-driven stochastic differential equations
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 233-248
-
- Article
- Export citation
Random fractals generated by a local Gaussian process indexed by a class of functions
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 249-269
-
- Article
- Export citation
Estimation for misspecified ergodic diffusion processesfrom discrete observations
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 270-290
-
- Article
- Export citation
Limit theorems for measure-valued processesof the level-exceedance type
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 291-319
-
- Article
- Export citation
Data-driven penalty calibration: A case studyfor Gaussian mixture model selection
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 320-339
-
- Article
- Export citation
Expansions for Repeated Integrals of Productswith Applications to the Multivariate Normal
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 340-357
-
- Article
- Export citation
KPZ formula for log-infinitely divisible multifractal random measures
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 358-371
-
- Article
- Export citation
Dislocation measure of the fragmentationof a general Lévy tree
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 372-389
-
- Article
- Export citation
A note on spider walks
-
- Published online by Cambridge University Press:
- 05 January 2012, pp. 390-401
-
- Article
- Export citation