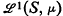Most work in genetic algebras has been concerned with inheritance which is symmetric with respect to sex, in that the characters studied are determined by genes located at autosomal loci, and it is assumed that the segregation pattern is the same in males and females. When asymmetric situations are studied, the development of the theory is complicated by the higher dimensions of the algebras, and by a feature to which Etherington (3, p. 40) drew attention, namely the fact that the passage from the gametic to the zygotic algebra no longer quite corresponds to the process of duplication, as it does in the symmetric case. Etherington gave some results for the gametic and zygotic algebras of a single sex linked diallelic locus, and its properties were discussed further by Gonshor (4, p. 44). In a second paper (5, p. 334) Gonshor studied sex linkage in the case of multiple alleles, choosing a canonical basis which exhibited very clearly the multiplication table and ideal structure of the algebra. His treatment from the statement of the multiplication table in terms of the natural basis to its expression in terms of a canonical basis, is repeated in the displayed relations (4)–(8) below, for completeness and to establish the present notation.

Consider uniform spaces X and Y and a separately uniformly continuous real-valued function f on X × Y. The following question arises in the theory of games: under what conditions can f be extended to a separately continuous function on × Ŷ, where , Ŷ are the completions of X and Y respectively? Firstly observe that such an extension is not always possible. If X = Y = (0, 1] with the usual uniform structure and f(x, y) = xy then f is separately uniformly continuous but has no separately continuous extension to × Ŷ = [0, 1]2 since such an extension would satisfy f(0, .) = 0 on Y and f(., 0) = 1 on X and so would necessarily have a discontinuity in one argument at the origin.

A problem of considerable interest in combinatorial analysis is that of determining the number of ways in which a connected figure can be constructed in the plane by assembling n regular hexagons in such a way that two hexagons abut on each other, if at all, along the whole of a common edge. Examples of these constructions can be seen in the various figures in this paper.

Etherington introduced certain algebraic methods into the study of population genetics (6). It was noted that algebras arising in genetic systems tend to have certain abstract properties and that these can be used to give elegant proofs of some classical stability theorems in population genetics (4, 5, 9, 10).

Dirac (2) and Plummer (5) independently investigated the structure of minimally 2-connected graphs G, which are characterized by the property that for any line x of G, G–x is not 2-connected. In this paper we investigate an analogous class of strongly connected digraphs D such that for any arc x, D–x is not strong. Not surprisingly, these digraphs have much in common with the minimally 2-connected graphs, and a number of theorems similar to those in (2) and (5) are proved, notably our Theorems 9 and 12.

We are concerned with the stability properties of uniformly closed wedges in C(E) (resp. C+(E)), the real-valued (resp. non-negative) continuous functionson a compact space E, and solve the following problems in this area:

(a) Let A be a closed semi-algebra in C+(E) such that

If X is a Tychonoff topological space, and if βX is the Stone-Cech compactification of X, then βX\X will denote the complement of X in βX. If A is a subset of X, then cl [A: X] will denote the closure of A in X, and int [A: X] will denote the interior of A in X. In Isbell ((3), p. 119) a property of βX\X is called a property which X has at infinity, and it is the aim of this paper to give necessary and sufficient conditions for X to be finite at infinity. Since βX is T1 we can say that if X is finite at infinity, then βX\X is closed in βX. So we lose nothing by restricting our attention to locally compact, Tychonoff spaces, and for the remainder of the paper X will denote such a space.

It is known (13, p. 92) that each closed normal cone in a weakly sequentially complete locally convex space is regular and fully regular. Part of the main theorem of this paper shows that a certain amount of weak sequential completeness is necessary in order that each closed normal cone be regular. Specifically, it is shown that each closed normal cone in a Fréchet space is regular if and only if each closed subspace with an unconditional basis is weakly sequentially complete. If E is a strongly separable conjugate of a Banach space it is shown that each closed normal cone in E is fully regular. If E is a Banach space with an unconditional basis it is shown that each closed normal cone in E is fully regular if and only if E is the conjugate of a Banach space.

We have previously studied in some detail the multiplicative properties of a given arithmetic function f with respect to a fixed basic sequence (see, for example, (1), (2)). We investigate here the structure of M(f), the collection of all basic sequences such that f is multiplicative with respect to , and in particular we focus our attention on the maximal members of M(f). Our principal result will be a proof that each maximal member of M(f) contains the same set of type II primitive pairs. Moreover, we will give a simple criterion for determining, in terms of the behaviour of f, whether or not a particular primitive pair (p, p) is in any (and therefore every) maximal member of M(f).

The study of periodic, irrotational waves of finite amplitude in an incompressible fluid of infinite depth was reduced by Levi-Civita (1) to the determination of a function

regular analytic in the interior of the unit circle ρ = 1 and which satisfies the condition

The concept of a pseudo-ring was introduced by Patterson (1). Briefly, a pseudo-ring is an algebraic system consisting of an additive abelian group A, a distinguished subgroup A*, and a multiplication operation A* × A→A under which A* is a ring and A a left A*-module. For convenience, we denote the pseudo-ring by = (A*, A). For the definitions of the various types of ideal, we refer the reader to (1).

If we consider a semigroup, its algebraic structure may be such that it is isomorphic to a subsemigroup of a group, or is algebraically embeddable in a group. This problem was investigated in 1931 by Ore who obtained in (4) a set of necessary conditions for this embedding. A necessary condition is that the semigroup should be cancellative: for any a, x, y in the semigroup either xa = ya or ax = ay implies that x = y. Malcev in (3) showed that this was not sufficient. It is enough to note that his example was a non-commutative semigroup: a commutative cancellative semigroup is embeddable algebraically in a group.

In this paper we introduce a generalised Hankel operator and generalised Erdélyi-Kober operators and deduce some relations between them. The operators are then applied to obtain solutions to some dual integral equations which have applications in diffraction theory.

In (4) J. F. C. Kingman and A. P. Robertson introduced the idea of thin sets in certain ℒ1 spaces. Thin sets are extreme cases of sets which are not total, and so the problem naturally arises of partitioning a measure space relative to a given set of integrable functions in such a way that on each element of the partition, the set of functions is either thin or total in a sense which is made precise below. In the present note, such partitions are obtained in §2 for finite or totally σ-finite measure spaces. In §3 the basic ideas are reformulated in terms of Radon measures on locally compact spaces, leading to an extension of the results of §2 in this context.

The resolution of a small initial discontinuity in a gas is examined using the linearised Navier-Stokes equations. The smoothing of the resultant contact surface and sound waves due to dissipation results in small flows which interact. The problem is solved for arbitrary Prandtl number by using a Fourier transform in space and a Laplace transform in time. The Fourier transform is inverted exactly and the density perturbation is found as two asymptotic series valid for small dissipation near the contact surface and the sound waves respectively. The modifications to the structures of the contact surface and the sound waves are exhibited.

In an earlier paper (5) a description was given in set-theoretic terms of the semigroup generated by the idempotents of a full transformation semigroup , one of the results being that if X is finite then every element of that is not bijective is expressible as a product of idempotents. In view of this it was natural to ask whether by analogy every singular square matrix is expressibleas a product of idempotent matrices. This is indeed the case, as was shown by J. A. Erdos (2). Magill (6) has considered products of idempotents in thesemigroup of all continuous self-maps of a topological space X, but a comparable characterization of products of idempotents in this case appears to be extremelydifficult, and no solution is available yet.

A smooth map of a differentiable n-manifold into Euclidean (n+k)-space is called an immersion if its Jacobian has rank n at each point of M. If f is also 1-1, it is called an embedding.

In this paper expressions are derived for the unknown coefficients in the dual cosine series:

where α is a given constant, and f1(x) and f2(x) are prescribed functions.

Results of Arscott (1) and Jayne (3) on real matrices are generalized to obtain bounds for the real parts of the eigenvalues of certain complex tridiagonal matrices, and bounds for the imaginary parts of the eigenvalues of other tridiagonal matrices are given. It is shown that analogous results hold for zeros of the permanent of certain characteristic matrices.

Let X be a complex normed space, with dual space X′. Let T be a bounded linear operator on X. The numerical range V(T) of T is defined as {f(Tx): x ∈ X, f ∈ X′, ‖ x ‖ = ‖ f ‖ = f(x) = 1}, and the numerical radius v(T) of T is defined as sup {|z|: z ∈ V(T)}. For a unital Banach algebra A, the numerical range V(a) of a ∈ A is defined as V(Ta), where Ta is the operator on A defined by Tab = ab. It is shown in (2, Chapter 1.2, Lemma 2) that V(a) = {f(a): f ∈ D(1)}, where D(1) = {f ∈ A′: ‖f‖ = f(1) = 1}.