Research Article
Examples of 3-knots with no minimal Seifert manifolds
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 417-420
-
- Article
- Export citation
Some free groups of matrices and the Burau representation of B4
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 225-228
-
- Article
- Export citation
Square-full numbers in short intervals
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 1-3
-
- Article
- Export citation
Some self-dual local rings of integers not free over their associated orders
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 5-10
-
- Article
- Export citation
Cofiniteness and vanishing of local cohomology modules
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 421-429
-
- Article
- Export citation
Groups of Wielandt length two
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 229-244
-
- Article
- Export citation
Nonsingular systems of two length three equations over a group
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 11-24
-
- Article
- Export citation
Constructive complete distributivity II
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 245-249
-
- Article
- Export citation
Products of idempotent integer matrices
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 431-441
-
- Article
- Export citation
Paracompact locales and metric spaces
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 251-256
-
- Article
- Export citation
The integral cohomology rings of some p-groups
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 25-32
-
- Article
- Export citation
Hall–Littlewood polynomials at roots of 1 and modular representations of the symmetric group
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 443-453
-
- Article
- Export citation
A characterization of pie limits
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 33-47
-
- Article
- Export citation
Continuity of derivations on Mal'cev H*-algebras
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 455-459
-
- Article
- Export citation
Characteristic classes invariant under stable fibre homotopy type
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 257-260
-
- Article
- Export citation
On cocycle bitorsors and gerbes over a Grothendieck topos
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 49-55
-
- Article
- Export citation
On complete curves in moduli space I
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 461-466
-
- Article
- Export citation
Bass number characterization of surjective Buchsbaum modules
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 261-279
-
- Article
- Export citation
On complete curves in moduli space II
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 467-472
-
- Article
- Export citation
The Lindelöf Tychonoff Theorem and choice principles
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 57-65
-
- Article
- Export citation


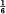 would imply a ‘quasi-Riemann Hypothesis’ of the type
would imply a ‘quasi-Riemann Hypothesis’ of the type 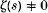 for
for 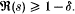 . Thus (1) is essentially as sharp as one can hope for at present. From (1) it follows that, for the number of square-full integers in a short interval, we have
. Thus (1) is essentially as sharp as one can hope for at present. From (1) it follows that, for the number of square-full integers in a short interval, we have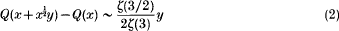
 and
and 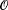
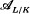 of the extension
of the extension 
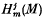 are known to be Artinian. Grothendieck [3], exposé 13, 1·2 made the following conjecture:
are known to be Artinian. Grothendieck [3], exposé 13, 1·2 made the following conjecture: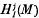 ) is finitely generated.
) is finitely generated. . From the definition it follows that
. From the definition it follows that 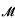
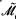
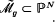 with hypersurfaces in sufficiently general position one obtains a complete curve in
with hypersurfaces in sufficiently general position one obtains a complete curve in 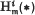 stands for the
stands for the