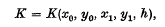Research Article
Sur les familles indenombrables de suites de nombres naturels et les problemes concernant la propriete C
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 109-126
-
- Article
- Export citation
On sets of fractional dimensions (II)
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 127-133
-
- Article
- Export citation
The reflexion of sound pulses by convex parabolic reflectors
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 134-149
-
- Article
- Export citation
The motion of a solid through an infinite liquid under no forces
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 150-167
-
- Article
- Export citation
The action in a uniform field
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 168-176
-
- Article
- Export citation
On the stability of crystal lattices: VI. The properties of matter under high pressure and the lattice theory of crystals
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 177-185
-
- Article
- Export citation
Deuteron bombardment of gold
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 186-193
-
- Article
- Export citation
Research Note
On colouring the nodes of a network
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 194-197
-
- Article
- Export citation
Front matter
PSP volume 37 issue 2 Cover and Front matter
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back matter
PSP volume 37 issue 2 Cover and Back matter
-
- Published online by Cambridge University Press:
- 24 October 2008, p. b1
-
- Article
-
- You have access
- Export citation

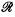 et
et  respectivement l'ensemble des nombres rationnels et l'ensemble des nombres irrationnels de cet intervalle.
respectivement l'ensemble des nombres rationnels et l'ensemble des nombres irrationnels de cet intervalle.
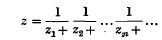
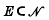 correspond à une famille Φ de suites de nombres naturels si l'on a
correspond à une famille Φ de suites de nombres naturels si l'on a