No CrossRef data available.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Published online by Cambridge University Press: 24 October 2008
1. Any number x between 0 and 1 may be expressed uniquely in the form
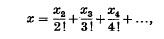
where xr is a non-negative integer less than r (r = 2,3,…). We consider the set E of numbers x for which
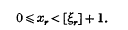
We establish an inequality connecting the dimensional number of the set E with certain constants of the series
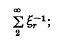
in particular we show that, when ξτ = rθ, the dimensional number of E is θ. We are concerned with the measure of Hausdorff.
† Hausdorff, F., “Dimension und äusseres Mass”, Math. Annalen, 79 (1919), 157–79.CrossRefGoogle Scholar
‡ Besicovitch, A. S., “Linear sets of points of fractional dimensions”, Math. Annalen, 101 (1929), 161–93.CrossRefGoogle Scholar
§ We write s − m*E in place of t s − m*E where there can be no ambiguity.
† Best, , “A closed dimensionless linear set”, Proc. Edinburgh Math. Soc. (2), 6 (1939), 105–8.CrossRefGoogle Scholar
† Gillis, J., “Note on a theorem of Myrberg”, Proc. Cambridge Phil. Soc. 33 (1937), 419–24CrossRefGoogle Scholar
† For if 0 <f−mE<∞ and 
then g−mE = ∞.
† I am indebted to the referee here for a considerable simplification of the argument.