Research Article
Isomorphism of structures in S-toposes
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 449-459
-
- Article
- Export citation
Busy beaver sets and the degrees of unsolvability1
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 460-474
-
- Article
- Export citation
First-order topological axioms
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 475-489
-
- Article
- Export citation
The metamathematics of model theory: Discovering language in action
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 490-498
-
- Article
- Export citation
Preservation of saturation and stability in a variety of nilpotent groups
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 499-512
-
- Article
- Export citation
Théories instables
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 513-522
-
- Article
- Export citation
An algebraic result about soft model theoretical equivalence relations with an application to H. Friedman's fourth problem
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 523-530
-
- Article
- Export citation
On the proof theory of the modal logic for arithmetic provability
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 531-538
-
- Article
- Export citation
Twilight graphs
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 539-571
-
- Article
- Export citation
Recursive isomorphism types of recursive Boolean algebras
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 572-594
-
- Article
- Export citation
Recursive Boolean algebras with recursive atoms
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 595-616
-
- Article
- Export citation
Recursively presented Abelian groups: Effective p-Group theory. I
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 617-624
-
- Article
- Export citation
Analysis without actual infinity
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 625-633
-
- Article
- Export citation
A result on implications of Σ1-sentences and its application to normal form theorems
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 634-642
-
- Article
- Export citation
Producing measurable cardinals beyond κ
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 643-648
-
- Article
- Export citation
Predicate functors revisited
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 649-652
-
- Article
- Export citation
Some facts about Kurt Gödel
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 653-659
-
- Article
- Export citation
Reviews
M.D. Krol′. The topological models of intuitionistic analysis. One counterexample. Mathematical notes, vol. 19 (1976), pp. 503–504. (English translation of K topologičéskim modélám intuicionistskogo analiza, Odin kontrprimér, Matématičéskié zamétki, vol. 19 (1976), pp. 859–862.) - M. D. Krol. A topological model for intuitionistic analysis with Kripke's scheme. Zeitschrift für mathematische Logik und Grundlagen der Mathematik, vol. 24 (1978), pp. 427–436. - M. D. Krol′. Distinct variants of Kripke's schema in intuitionistic analysis. Soviet mathematics, vol. 19 (1978), pp. 474–477. (English translation by B. F. Wells of Različnyé varianty shémy Kripké v intuicionistskom analizé, Doklady Akadémii Nauk SSSR, vol. 239 (1978), pp. 1048–1051.)
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 660-661
-
- Article
- Export citation
Robert M. Solovay. Provability interpretations of modal logic. Israel journal of mathematics, vol. 25 (1976), pp. 287–304.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 661-662
-
- Article
- Export citation
Matatyahu Rubin. Theories of linear order. Israel journal of mathematics, vol. 17 (1974), pp. 392–443.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 662-663
-
- Article
- Export citation

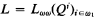 is an (
is an ( is
is 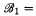
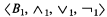 and
and  , we write
, we write 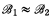 if
if 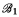 is isomorphic to
is isomorphic to 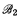 and
and  if
if 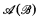 will denote the set of atoms of
will denote the set of atoms of 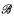 and
and 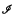 (
(
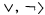 is said to be
is said to be 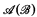 denote the set of atoms of
denote the set of atoms of 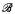 and
and 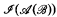 denote the ideal generated by the atoms of
denote the ideal generated by the atoms of  and
and 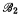 , we write
, we write 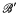 such that
such that 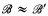 and both
and both  and
and 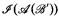 are recursive and (ii) for any nonzero r.e. degree
are recursive and (ii) for any nonzero r.e. degree  ,
, 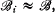
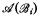 is of degree
is of degree 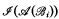 is recursive,
is recursive,