This is an analytical study of the effect of level-shift and temporary-change components, when present but neglected, on the trace test for cointegration. The contribution is threefold. First, we discuss in a multivariate framework, and jointly, effects that in the previous literature have been discussed in a univariate setting and in isolation. Second, we consider a rather general specification of shifts and outliers with random size, number, and timing and with flexible dynamics. It nests the classical cases of additive shifts, innovational outliers, and additive outliers. Third, as an instrument for this analysis we develop an asymptotic theory for product moment matrices of linear processes with stochastic level-shift components, generalizing results of Leipus and Viano (2003, Statistics and Probability Letters 61, 177–190).

By pointing out the spurious regression problem, Granger and Newbold (1974) have shown the importance of stochastic trends in time series data in the context of linear regression models. At the time, removing trends by differencing was already common practice in univariate time series modeling as part of the Box–Jenkins approach (Box and Jenkins, 1976). These new developments, however, emphasized the importance of autoregressive (AR) unit roots and motivated the development of statistical procedures for their detection. Dickey and Fuller (1979) and Fuller (1976) were pioneers in developing tests for unit roots that became widely used. The foundation of asymptotic theory for regressions involving stochastic trends was led by Phillips (1986, 1987) with the introduction of the functional limit theory, weak convergence methods, convergence to stochastic integrals, nonparametric unit root testing, and continuous record asymptotics. Phillips and Durlauf (1986) extended some of these results to the multivariate setting by presenting the multivariate invariance principles and the asymptotic theory of multivariate nonstationary and cointegrating regressions. These contributions provided the asymptotic tools that have served as the basis for most of the limit results derived in the context of unit root nonstationarity, and they have stimulated extensive subsequent research.We are grateful to Peter Phillips for proposing a special issue of Econometric Theory for papers from our conference Unit Root and Cointegration Testing. We thank all participants of the conference who contributed their papers to this special issue. We are also very grateful to those colleagues who agreed to serve as referees for the papers. They not only provided generous help through comments but also respected our rather tight deadlines. We are grateful to all those who helped us to complete the special issue unusually fast. To protect their identities, we do not list them here. They will be included in the general list of referees for Econometric Theory. Our conference would not have been possible without the generous financial support of a number of sponsors. We are grateful to the Bank of Portugal, to the Portuguese Science Foundation (FCT), to the Luso-American Foundation for Development (FLAD), and to the Journal of Applied Econometrics for their financial support.

We propose a nonparametric test of conditional independence based on the weighted Hellinger distance between the two conditional densities, f(y|x,z) and f(y|x), which is identically zero under the null. We use the functional delta method to expand the test statistic around the population value and establish asymptotic normality under β-mixing conditions. We show that the test is consistent and has power against alternatives at distance n−1/2h−d/4. The cases for which not all random variables of interest are continuously valued or observable are also discussed. Monte Carlo simulation results indicate that the test behaves reasonably well in finite samples and significantly outperforms some earlier tests for a variety of data generating processes. We apply our procedure to test for Granger noncausality in exchange rates.

We propose an estimation procedure for a semiparametric panel data censored regression model in which the error terms may be subject to general forms of nonstationarity. Specifically, we allow for heteroskedasticity over time and a time varying factor load on the individual specific effect. Empirically, estimation of this model would be of interest to explore how returns to unobserved skills change over time—see, e.g., Chay (1995, manuscript, Princeton University) and Chay and Honoré (1998, Journal of Human Resources 33, 4–38). We adopt a two-stage procedure based on nonparametric median regression, and the proposed estimator is shown to be 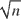-consistent and asymptotically normal. The estimation procedure is also useful in the group effect setting, where estimation of the factor load would be empirically relevant in the study of the intergenerational correlation in income, explored in Solon (1992, American Economic Review 82, 393–408; 1999, Handbook of Labor Economics, vol. 3, 1761–1800) and Zimmerman (1992, American Economic Review 82, 409–429).

This paper considers a series estimator of E[α(Y)|λ(X) = λ̄], (α,λ) ∈ 𝛢 × Λ, indexed by function spaces, and establishes the estimator's uniform convergence rate over λ̄ ∈ R, α ∈ 𝛢, and λ ∈ Λ, when 𝛢 and Λ have a finite integral bracketing entropy. The rate of convergence depends on the bracketing entropies of 𝛢 and Λ in general. In particular, we demonstrate that when each λ ∈ Λ is locally uniformly ℒ2-continuous in a parameter from a space of polynomial discrimination and the basis function vector pK in the series estimator keeps the smallest eigenvalue of E[pK(λ(X))pK(λ(X))‼] above zero uniformly over λ ∈ Λ, we can obtain the same convergence rate as that established by de Jong (2002, Journal of Econometrics 111, 1–9).

Model selection procedures have become an integral part of almost any statistical or econometric analysis of data. Prominent examples include the selection of explanatory variables in (non)linear regression or lag-length selection in time series models. It is safe to say that these methods have become an integral part of the toolbox of modern data analysis. Moreover, research on model selection (or related procedures such as model averaging and shrinkage procedures) and its implications for statistical analysis has intensified in recent years. This is witnessed by the fact that it is difficult to pick up any recent issue of an econometrics or statistics journal and avoid encountering a paper where some form of model selection, or model averaging, or general shrinkage estimation is considered.

Conference program.

In this paper, new fully nonparametric estimators of the diffusion coefficient of continuous time models are introduced. The estimators are based on Fourier analysis of the state variable trajectory observed and on the estimation of quadratic variation between observations by means of realized volatility. The estimators proposed are shown to be consistent and asymptotically normally distributed. Moreover, the Fourier estimator can be iterated to get a fully nonparametric estimate of the diffusion coefficient in a bivariate model in which one state variable is the volatility of the other. The estimators are shown to be unbiased in small samples using Monte Carlo simulations and are used to estimate univariate and bivariate models for interest rates.

High-breakdown-point regression estimators protect against large errors and data contamination. We generalize the concept of trimming used by many of these robust estimators, such as the least trimmed squares and maximum trimmed likelihood, and propose a general trimmed estimator, which renders robust estimators applicable far beyond the standard (non)linear regression models. We derive here the consistency and asymptotic distribution of the proposed general trimmed estimator under mild β-mixing conditions and demonstrate its applicability in nonlinear regression and limited dependent variable models.

A limit theory is developed for multivariate regression in an explosive cointegrated system. The asymptotic behavior of the least squares estimator of the cointegrating coefficients is found to depend upon the precise relationship between the explosive regressors. When the eigenvalues of the autoregressive matrix Θ are distinct, the centered least squares estimator has an exponential Θn rate of convergence and a mixed normal limit distribution. No central limit theory is applicable here, and Gaussian innovations are assumed. On the other hand, when some regressors exhibit common explosive behavior, a different mixed normal limiting distribution is derived with rate of convergence reduced to . In the latter case, mixed normality applies without any distributional assumptions on the innovation errors by virtue of a Lindeberg type central limit theorem. Conventional statistical inference procedures are valid in this case, the stationary convergence rate dominating the behavior of the least squares estimator.

An I(0) process is commonly defined as a process that satisfies a functional central limit theorem, i.e., whose scaled partial sums converge weakly to a Wiener process, and an I(1) process as a process whose first differences are I(0). This paper establishes that with this definition, it is impossible to consistently discriminate between I(0) and I(1) processes. At the same time, on a more constructive note, there exist consistent unit root tests and also nontrivial inconsistent stationarity tests with correct asymptotic size.

Shrinkage estimation procedures such as ridge regression and the lasso have been proposed for stabilizing estimation in linear models when high collinearity exists in the design. In this paper, we consider asymptotic properties of shrinkage estimators in the case of “nearly singular” designs.I thank Hannes Leeb and Benedikt Pötscher and also the referees for their valuable comments. This research was supported by a grant from the Natural Sciences and Engineering Research Council of Canada.

This paper investigates the asymptotic behavior of tests in situations where the likelihood is locally asymptotically quadratic. Necessary and sufficient conditions are given for a test to be admissible. Even without these restrictive parametric assumptions, it is shown that certain common procedures—such as the augmented Dickey–Fuller test in cases where no deterministic trend is present or standard tests for restrictions on cointegrating relationships—are asymptotically inadmissible. These results confirm the existence of tests that dominate these classical tests for all parameters.I express my gratitude to the editors, H. Lütkepohl and especially Peter C.B. Phillips, for their help, which enormously exceeded the usual amount of support. Also I thank the referees for their helpful comments. Their contribution greatly improved the paper. All remaining errors are mine.

Asset returns are frequently assumed to be determined by one or more common factors. We consider a bivariate factor model where the unobservable common factor and idiosyncratic errors are stationary and serially uncorrelated but have strong dependence in higher moments. Stochastic volatility models for the latent variables are employed, in view of their direct application to asset pricing models. Assuming that the underlying persistence is higher in the factor than in the errors, a fractional cointegrating relationship can be recovered by suitable transformation of the data. We propose a narrow band semiparametric estimate of the factor loadings, which is shown to be consistent with a rate of convergence, and its finite-sample properties are investigated in a Monte Carlo experiment.

This paper derives asymptotic normality of a class of M-estimators in the generalized autoregressive conditional heteroskedastic (GARCH) model. The class of estimators includes least absolute deviation and Huber's estimator in addition to the well-known quasi maximum likelihood estimator. For some estimators, the asymptotic normality results are obtained only under the existence of fractional unconditional moment assumption on the error distribution and some mild smoothness and moment assumptions on the score function.

We consider the problem of estimating the unconditional distribution of a post-model-selection estimator. The notion of a post-model-selection estimator here refers to the combined procedure resulting from first selecting a model (e.g., by a model-selection criterion such as the Akaike information criterion [AIC] or by a hypothesis testing procedure) and then estimating the parameters in the selected model (e.g., by least squares or maximum likelihood), all based on the same data set. We show that it is impossible to estimate the unconditional distribution with reasonable accuracy even asymptotically. In particular, we show that no estimator for this distribution can be uniformly consistent (not even locally). This follows as a corollary to (local) minimax lower bounds on the performance of estimators for the distribution; performance is here measured by the probability that the estimation error exceeds a given threshold. These lower bounds are shown to approach ½ or even 1 in large samples, depending on the situation considered. Similar impossibility results are also obtained for the distribution of linear functions (e.g., predictors) of the post-model-selection estimator.The research of the first author was supported by the Max Kade Foundation and by the Austrian National Science Foundation (FWF), Grant no. P13868-MAT. A preliminary draft of the material in this paper was written in 1999.

This paper introduces a simple first-difference-based approach to estimation and inference for the AR(1) model. The estimates have virtually no finite-sample bias and are not sensitive to initial conditions, and the approach has the unusual advantage that a Gaussian central limit theory applies and is continuous as the autoregressive coefficient passes through unity with a uniform rate of convergence. En route, a useful central limit theorem (CLT) for sample covariances of linear processes is given, following Phillips and Solo (1992, Annals of Statistics, 20, 971–1001). The approach also has useful extensions to dynamic panels.

Weak convergence of partial sums and multilinear forms in independent random variables and linear processes and their nonlinear analogues to stochastic integrals now plays a major role in nonstationary time series and has been central to the development of unit root econometrics. The present paper develops a new and conceptually simple method for obtaining such forms of convergence. The method relies on the fact that the econometric quantities of interest involve discrete time martingales or semimartingales and shows how in the limit these quantities become continuous martingales and semimartingales. The limit theory itself uses very general convergence results for semimartingales that were obtained in the work of Jacod and Shiryaev (2003, Limit Theorems for Stochastic Processes). The theory that is developed here is applicable in a wide range of econometric models, and many examples are given. %One notable outcome of the new approach is that it provides a unified treatment of the asymptotics for stationary, explosive, unit root, and local to unity autoregression, and also some general nonlinear time series regressions. All of these cases are subsumed within the martingale convergence approach, and different rates of convergence are accommodated in a natural way. Moreover, the results on multivariate extensions developed in the paper deliver a unification of the asymptotics for, among many others, models with cointegration and also for regressions with regressors that are nonlinear transforms of integrated time series driven by shocks correlated with the equation errors. Because this is the first time the methods have been used in econometrics, the exposition is presented in some detail with illustrations of new derivations of some well-known existing results, in addition to the provision of new results and the unification of the limit theory for autoregression.

This paper presents a method for estimating a class of panel data duration models, under which an unknown transformation of the duration variable is linearly related to the observed explanatory variables and the unobserved heterogeneity (or frailty) with completely known error distributions. This class of duration models includes a panel data proportional hazards model with fixed effects. The proposed estimator is shown to be n1/2-consistent and asymptotically normal with dependent right censoring. The paper provides some discussions on extending the estimator to the cases of longer panels and multiple states. Some Monte Carlo studies are carried out to illustrate the finite-sample performance of the new estimator.

Here, we derive optimal rank-based tests for noncausality in the sense of Granger between two multivariate time series. Assuming that the global process admits a joint stationary vector autoregressive (VAR) representation with an elliptically symmetric innovation density, both no feedback and one direction causality hypotheses are tested. Using the characterization of noncausality in the VAR context, the local asymptotic normality (LAN) theory described in Le Cam (1986, Asymptotic Methods in Statistical Decision Theory) allows for constructing locally and asymptotically optimal tests for the null hypothesis of noncausality in one or both directions. These tests are based on multivariate residual ranks and signs (Hallin and Paindaveine, 2004a, Annals of Statistics 32, 2642–2678) and are shown to be asymptotically distribution free under elliptically symmetric innovation densities and invariant with respect to some affine transformations. Local powers and asymptotic relative efficiencies are also derived. The level, power, and robustness (to outliers) of the resulting tests are studied by simulation and are compared to those of the Wald test. Finally, the new tests are applied to Canadian money and income data.