Let (ωi, σi, μi.) be two positive finite measure spaces, V a non-zero Hilbert space, and 1 ≤ p < ∞, p # 2. In this article it is shown that each surjective linear isometry between the Bochner spaces 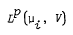 induces a Boolean isomorphism between the measure algebras
induces a Boolean isomorphism between the measure algebras 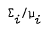 , thus generalizing a result of Cambern's for separable Hilbert spaces.
, thus generalizing a result of Cambern's for separable Hilbert spaces.
This Banach–Stone type theorem is achieved via a description of the Lp-structure of 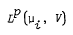 .
.