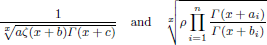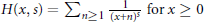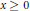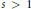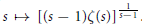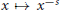The aim of this paper is to develop analytic techniques to deal with the monotonicity of certain combinatorial sequences. On the one hand, a criterion for the monotonicity of the function 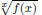 is given, which is a continuous analogue of a result of Wang and Zhu. On the other hand, the log-behaviour of the functions
is given, which is a continuous analogue of a result of Wang and Zhu. On the other hand, the log-behaviour of the functions
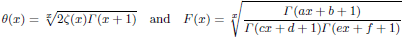
is considered, where ζ(x) and Γ(x) are the Riemann zeta function and the Euler Gamma function, respectively. Consequently, the strict log-concavities of the function θ(x) (a conjecture of Chen et al.) and 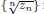 for some combinatorial sequences (including the Bernoulli numbers, the tangent numbers, the Catalan numbers, the Fuss–Catalan numbers, and the binomial coefficients
for some combinatorial sequences (including the Bernoulli numbers, the tangent numbers, the Catalan numbers, the Fuss–Catalan numbers, and the binomial coefficients 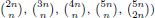 are demonstrated. In particular, this contains some results of Chen et al., and Luca and Stănică. Finally, by researching the logarithmically complete monotonicity of some functions, the infinite log-monotonicity of the sequence
are demonstrated. In particular, this contains some results of Chen et al., and Luca and Stănică. Finally, by researching the logarithmically complete monotonicity of some functions, the infinite log-monotonicity of the sequence
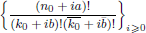
is proved. This generalizes two results of Chen et al. that both the Catalan numbers  and the central binomial coefficients
and the central binomial coefficients 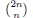 are infinitely log-monotonic, and strengthens one result of Su and Wang that
are infinitely log-monotonic, and strengthens one result of Su and Wang that 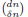 is log-convex in n for positive integers d > δ. In addition, the asymptotically infinite log-monotonicity of derangement numbers is showed. In order to research the stronger properties of the above functions θ(x) and F(x), the logarithmically complete monotonicity of functions
is log-convex in n for positive integers d > δ. In addition, the asymptotically infinite log-monotonicity of derangement numbers is showed. In order to research the stronger properties of the above functions θ(x) and F(x), the logarithmically complete monotonicity of functions
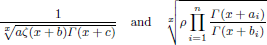
is also obtained, which generalizes the results of Lee and Tepedelenlioǧlu, and Qi and Li.

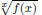 is given, which is a continuous analogue of a result of Wang and Zhu. On the other hand, the log-behaviour of the functions
is given, which is a continuous analogue of a result of Wang and Zhu. On the other hand, the log-behaviour of the functions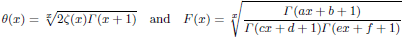
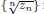 for some combinatorial sequences (including the Bernoulli numbers, the tangent numbers, the Catalan numbers, the Fuss–Catalan numbers, and the binomial coefficients
for some combinatorial sequences (including the Bernoulli numbers, the tangent numbers, the Catalan numbers, the Fuss–Catalan numbers, and the binomial coefficients 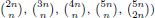 are demonstrated. In particular, this contains some results of Chen
are demonstrated. In particular, this contains some results of Chen 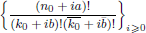
 and the central binomial coefficients
and the central binomial coefficients 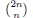 are infinitely log-monotonic, and strengthens one result of Su and Wang that
are infinitely log-monotonic, and strengthens one result of Su and Wang that 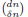 is log-convex in
is log-convex in