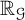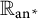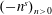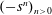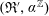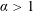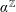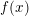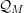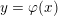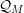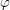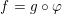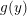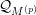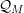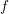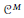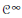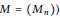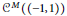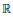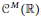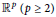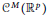5 results
Multisummability for generalized power series
- Part of
-
- Journal:
- Canadian Journal of Mathematics / Volume 76 / Issue 2 / April 2024
- Published online by Cambridge University Press:
- 27 February 2023, pp. 458-494
- Print publication:
- April 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Expansions of the Real Field by Canonical Products
- Part of
-
- Journal:
- Canadian Mathematical Bulletin / Volume 63 / Issue 3 / September 2020
- Published online by Cambridge University Press:
- 04 October 2019, pp. 506-521
- Print publication:
- September 2020
-
- Article
- Export citation
Composite quasianalytic functions
- Part of
-
- Journal:
- Compositio Mathematica / Volume 154 / Issue 9 / September 2018
- Published online by Cambridge University Press:
- 17 August 2018, pp. 1960-1973
- Print publication:
- September 2018
-
- Article
- Export citation
Pathological Phenomena in Denjoy–Carleman Classes
-
- Journal:
- Canadian Journal of Mathematics / Volume 68 / Issue 1 / 01 February 2016
- Published online by Cambridge University Press:
- 20 November 2018, pp. 88-108
- Print publication:
- 01 February 2016
-
- Article
-
- You have access
- Export citation
LINEAR CONTINUOUS EXTENSION OPERATORS FOR GEVREY CLASSES ON POLYSECTORS
-
- Journal:
- Glasgow Mathematical Journal / Volume 45 / Issue 2 / May 2003
- Published online by Cambridge University Press:
- 31 July 2003, pp. 199-216
- Print publication:
- May 2003
-
- Article
-
- You have access
- Export citation