Article contents
Development of the ambipolar electric field in a compressed current sheet and the impact on magnetic reconnection
Published online by Cambridge University Press: 19 January 2024
Abstract
Satellite data analysis of a compressed gyro-scale current sheet prior to magnetic reconnection in the magnetotail shows that electrostatic lower hybrid waves localized to the region of a transverse ambipolar electric field at the centre of the current sheet are driven by $\boldsymbol{E} \times \boldsymbol{B}$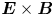 velocity shear and result from compression. The presence and location of shear-driven waves around the centre of the current sheet, where the magnetic field reverses and the density gradient is minimal, is consistent with our model. This is notable because the free energy source is the curvature of the electron $\boldsymbol{E} \times \boldsymbol{B}$
velocity shear and result from compression. The presence and location of shear-driven waves around the centre of the current sheet, where the magnetic field reverses and the density gradient is minimal, is consistent with our model. This is notable because the free energy source is the curvature of the electron $\boldsymbol{E} \times \boldsymbol{B}$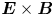 flow and not the density gradient. Laboratory experiments and particle-in-cell (PIC) simulations have shown that shear-driven lower hybrid fluctuations are capable of producing anomalous cross-field transport (viscosity) and resistivity, which can trigger magnetic reconnection. We estimate the terms in the generalized Ohm's Law directly from MMS data as the spacecraft cross a gyro-scale current sheet. Our analysis shows that the wave effects (resistivity, diffusion and viscosity) and pressure anisotropy effects are comparable. We also find that the quasi-static electric field gradient is correlated with a non-gyrotropic electron distribution function, which is consistent with our model. Furthermore, theoretical arguments suggest agyrotropy is an indicator of the possibility for magnetic reconnection to occur.
flow and not the density gradient. Laboratory experiments and particle-in-cell (PIC) simulations have shown that shear-driven lower hybrid fluctuations are capable of producing anomalous cross-field transport (viscosity) and resistivity, which can trigger magnetic reconnection. We estimate the terms in the generalized Ohm's Law directly from MMS data as the spacecraft cross a gyro-scale current sheet. Our analysis shows that the wave effects (resistivity, diffusion and viscosity) and pressure anisotropy effects are comparable. We also find that the quasi-static electric field gradient is correlated with a non-gyrotropic electron distribution function, which is consistent with our model. Furthermore, theoretical arguments suggest agyrotropy is an indicator of the possibility for magnetic reconnection to occur.
- Type
- Research Article
- Information
- Copyright
- Copyright © The Author(s), 2024. Published by Cambridge University Press
References
- 3
- Cited by



