Research Article
Fibred semantics and the weaving of logics. Part 1: Modal and intuitionistic logics
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1057-1120
-
- Article
- Export citation
Some model theory for almost real closed fields
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1121-1152
-
- Article
- Export citation
Cauchy completeness in elementary logic
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1153-1157
-
- Article
- Export citation
Minimal complementation below uniform upper bounds for the arithmetical degrees
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1158-1192
-
- Article
- Export citation
Strong termination for the epsilon substitution method
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1193-1205
-
- Article
- Export citation
Uniformization, choice functions and well orders in the class of trees
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1206-1227
-
- Article
- Export citation
A recursive nonstandard model of normal open induction
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1228-1241
-
- Article
- Export citation
Some characterization theorems for infinitary universal Horn logic without equality
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1242-1260
-
- Article
- Export citation
If there is an exactly λ-free abelian group then there is an exactly λ-separable one in λ
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1261-1278
-
- Article
- Export citation
Countable models of trivial theories which admit finite coding
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1279-1286
-
- Article
- Export citation
Boolean operations, Borel sets, and Hausdorff's question
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1287-1304
-
- Article
- Export citation
Forcing isomorphism II
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1305-1320
-
- Article
- Export citation
Simple Gentzenizations for the normal formulae of contraction-less logics
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1321-1346
-
- Article
- Export citation
Noncappable enumeration degrees below 0e′
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1347-1363
-
- Article
- Export citation
The Π20 enumeration degrees are not dense
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1364-1379
-
- Article
- Export citation
On countably closed complete Boolean algebras
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1380-1386
-
- Article
- Export citation
Reviews
Kolaitis Phokion G. and Väänänen Jouko A.. Generalized quantifiers and pebble games on finite structures. Annals of pure and applied logic, vol. 74 (1995) pp. 23–75.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1387-1388
-
- Article
- Export citation
Richard Sommer. Transfinite induction within Peano arithmetic. Annals of pure and applied logic, vol. 76 (1995), pp. 231–289.
-
- Published online by Cambridge University Press:
- 12 March 2014, p. 1388
-
- Article
- Export citation
Lawrence S. Moss. Power set recursion. Annals of pure and applied logic, vol. 71 (1995), pp. 247–306.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1388-1389
-
- Article
- Export citation
Michael Dummett. Frege. Philosophy of mathematics. Duckworth, London, and Harvard University Press, Cambridge, Mass., 1991, xiii + 331 pp.
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1389-1391
-
- Article
- Export citation


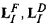 etc. Once the logics are ‘weaved’, further ‘interaction’ axioms can be geometrically motivated and added, and then systematically studied. The methodology is general and is applied to modal and intuitionistic logics as well as to general algebraic logics. We obtain general results on bulk, in the sense that we develop standard combining techniques and refinements which can be applied to any family of initial logics to obtain further combined logics.
etc. Once the logics are ‘weaved’, further ‘interaction’ axioms can be geometrically motivated and added, and then systematically studied. The methodology is general and is applied to modal and intuitionistic logics as well as to general algebraic logics. We obtain general results on bulk, in the sense that we develop standard combining techniques and refinements which can be applied to any family of initial logics to obtain further combined logics.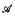 ≢
≢ 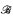 of finite type
of finite type 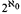
 operation. The first attempt to lay down their theory in a systematic fashion was the work of Kantorovich and Livenson [5], where they call these the
operation. The first attempt to lay down their theory in a systematic fashion was the work of Kantorovich and Livenson [5], where they call these the  ), the difference hierarchies of Hausdorff (
), the difference hierarchies of Hausdorff ( ): for each of these hierarchies, every level can be expressed as the range of an
): for each of these hierarchies, every level can be expressed as the range of an 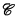 and
and 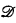 are collections of subsets of
are collections of subsets of 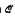 such that, in any
such that, in any