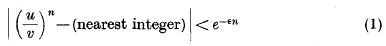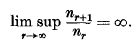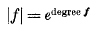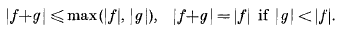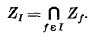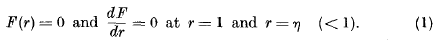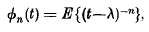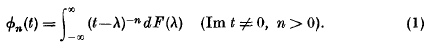Research Article
Cubic forms over algebraic number fields
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 97-101
-
- Article
- Export citation
Homogeneous forms of odd degree in a large number of variables
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 102-105
-
- Article
- Export citation
The distribution of quadratic residues and non-residues
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 106-112
-
- Article
- Export citation
On a method for the determination of class number factors in number fields
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 113-121
-
- Article
- Export citation
On the fractional parts of the powers of a rational number (II)
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 122-124
-
- Article
- Export citation
Rational approximations to algebraic numbers
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 125-131
-
- Article
- Export citation
The product of n linear forms in a field of series
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 132-137
-
- Article
- Export citation
Note on two theorems about function algebras
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 138-139
-
- Article
- Export citation
On the expansion of functions satisfying four boundary conditions
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 140-145
-
- Article
- Export citation
An inversion formula for a generalized transform
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 146-155
-
- Article
- Export citation
The relation between load and penetration for a spherical punch
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 156-161
-
- Article
- Export citation
The twisted sphere
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 162-165
-
- Article
- Export citation
On the solution of problems of dynamic plane elasticity
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 166-168
-
- Article
- Export citation
Index
Index to volume 4
-
- Published online by Cambridge University Press:
- 26 February 2010, p. 169
-
- Article
- Export citation
Front matter
MTK volume 4 issue 2 Front matter
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. f1-f2
-
- Article
-
- You have access
- Export citation

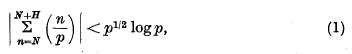
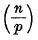 being Legendre's symbol of quadratic character.
being Legendre's symbol of quadratic character.