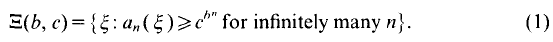Let (Sn)n = 1,2,… be the strictly increasing sequence of those natural numbers that can be represented as the sum of three cubes of positive integers. The estimate

is easily proved as follows: Let x1 be the largest natural number with Then This procedure is iterated by choosing x2 and then x3 as the largest natural numbers satisfying and Thus Since this implies (1).

In this paper we investigate the solutions in integers x, y, z, X, Y, Z of the system

where P is a real number that may be taken to be arbitrarily large, and the (fixed) integer exponent h satisfies h≥4. The system has 6P3 + O(P2) “trivial” solutions in which x, y, z are a permutation of X, Y, Z. Our result implies that the number of non-trivial solutions is at most o(P3), so that the total number of solutions is asymptotic to 6P3.

Let ν be a valuation of any rank of a field K with value group Gν and f(X)= Xm + alXm−1 + … + am be a polynomial over K. In this paper, it is shown that if (ν(ai)/i)≥(ν(am)/m)>0 for l≤i≤m, and there does not exist any integer r>1 dividing m such that ν(am)/r∈Gν, then f(X) is irreducible over K. It is derived as a special case of a more general result proved here. It generalizes the usual Eisenstein Irreducibility Criterion and an Irreducibility Criterion due to Popescu and Zaharescu for discrete, rank-1 valued fields, (cf. [Journal of Number Theory, 52 (1995), 98–118]).

Recently, it has been shown that tight or almost tight upper bounds for the discrepancy of many geometrically denned set systems can be derived from simple combinatorial parameters of these set systems. Namely, if the primal shatter function of a set system ℛ on an n-point set X is bounded by const. md, then the discrepancy disc (ℛ) = O(n(d−1)/2d) (which is known to be tight), and if the dual shatter function is bounded by const. md, then disc We prove that for d = 2, 3, the latter bound also cannot be improved in general. We also show that bounds on the shatter functions alone do not imply the average (L1)-discrepancy to be much smaller than the maximum discrepancy: this contrasts results of Beck and Chen for certain geometric cases. In the proof we give a construction of a certain asymptotically extremal bipartite graph, which may be of independent interest.

Let [0;a1(ξ), a2(ξ),…] denote the continued fraction expansion of ξ∈[0, 1]. The problem of estimating the fractional dimension of sets of continued fractions emerged in late twenties in papers by Jarnik [6, 7] and Besicovitch [1] and since then has been addressed by a number of authors (see [2, 4, 5, 8, 9]). In particular, Good [4] proved that the set of all ξ, for which an(ξ)→∞ as n→∞ has the Hausdorff dimension ½ For the set of continued fractions whose expansion terms tend to infinity doubly exponentially the dimension decreases even further. More precisely, let

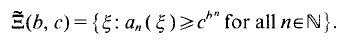
Hirst [5] showed that dim On the other hand, Moorthy [8] showed that dim 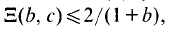where

In this note we point out that a simple proof of the lower bound of the sets (b, c), and so also of Ξ(b, c), defined in the previous paper [1] can be obtained as a simple application of a general method. By Example 4.6 from [2], if [0, 1] = E0⊃E1⊃ … are sets each of which is a finite union of disjoint closed intervals such that each interval of Ek−1, contains at least mk intervals of Ek which are separated by gaps of lengths at least εk, and if mk≥2 and εk≥εk+1>0, then the dimension of the intersection of Ek is at least

Call a set of natural numbers subset-sum-distinct (or SSD) if all pairwise distinct subsets have unequal sums. One wants to construct SSD sets in which the largest element is as small as possible. Given any SSD set, it is easy to construct an SSD set with one more element in which the biggest element is exactly double the biggest element in the original set. For any SSD set, we construct another SSD set with k more elements whose largest element is less than 2k times the largest element in the original set. This claim has been made previously for a different construction, but we show that that claim is false.

We provide a unified and simplified proof that for any partition of (0, 1] into sets that are measurable or have the property of Baire, one cell will contain an infinite sequence together with all of its sums (finite or infinite) without repetition. In fact any set which is large around 0 in the sense of measure or category will contain such a sequence. We show that sets with 0 as a density point have very rich structure. Call a sequence and its resulting all-sums set structured provided for each We show further that structured all-sums sets with positive measure are not partition regular even if one allows shifted all-sums sets. That is, we produce a two cell measurable partition of (0, 1 ] such that neither set contains a translate of any structured all-sums set with positive measure.

In what follows, R will denote a commutative domain with 1, and Q(≠R) its field of quotients, which is viewed here as an R-module. By RP we denote the localization of R at the maximal ideal P, and more generally, by MP = Rp⊗RM the localization of the R-module M at P, which we define to be the P-component of M. The symbol R* will mean the multiplicative monoid of nonzero elements of R. For a submonoid S of R*, Rs will denote the localization of R at S.

Decompositions of simply connected 4-manifolds into three closed 4-balls are studied from the view-point of abstract regular polytopes of Schläfli type {p, q, 2, 3}. The three balls correspond to three ditopes, their common intersection corresponds to a regular map of type {p, q} as an equilibrium surface whose genus equals the “genus” of the 4-manifold.

Let M be a compact flat Riemannian manifold of dimension n, and Γ its fundamental group. Then we have the following exact sequence (see [1])

where Zn is a maximal abelian subgroup of Γ and G is a finite group isomorphic to the holonomy group of M. We shall call Γ a Bieberbach group. Let T be a flat torus, and let Ggr act via isometries on T; then ┌ acts isometrically on × T where is the universal covering of M and yields a flat Riemannian structure on ( × T)/Γ. A flat-toral extension (see [9, p. 371]) of the Riemannian manifold M is any Riemannian manifold isometric to ( × T)/Γ where T is a flat torus on which Γ acts via isometries. It is convenient to adopt the convention that a single point is a 0-dimensional flat torus. If this is done, M is itself among the flat toral extensions of M. Roughly speaking, this is a way of putting together a compact flat manifold and a flat torus to make a new flat manifold the dimension of which is the sum of the dimensions of its constituents. It is, more precisely, a fibre bundle over the flat manifold with a flat torus as fibre.

Let X be a Banach space and Y its closed subspace having property U in X. We use a net (Aα) of continuous linear operators on X such that ‖ Aα ‖ ≤ 1, Aα (X) ⊂ Y for all α, and limαg(Aαy) = g(y), y ∈ Y, g∈Y* to obtain equivalent conditions for Y to be an HB-subspace, u-ideal or h-ideal of X. Some equivalent renormings of c0 and l2 are shown to provide examples of spaces X for which K(X) has property U in L(X) without being an HB-subspace. Considering a generalization of the Godun set [3], we establish some relations between Godun sets of Banach spaces and related operator spaces. This enables us to prove e.g., that if K(X) is an HB-subspace of L(X), then X is an HB-subspace of X**—the result conjectured to be true by Å. Lima [9].

We introduce a class of “differential operators” on graphs and we prove an energy estimate and a Liouville type theorem depending on some structural properties of the operators considered.

We consider the spectral function, ρα(μ), for –∞<μ<∞ associated with the Sturm-Liouville equation

and the boundary condition

We suppose that q is a real-valued member of L1[0, ∞) and λ is a real parameter.

Recently Edelman and Reiner suggested two poset structures, (n, d) and (n, d) on the set of all triangulations of the cyclic d-polytope C(n, d) with n vertices. Both posets are generalizations of the well-studied Tamari lattice. While (n, d) is bounded by definition, the same is not obvious for (n, d). In the paper by Edelman and Reiner the bounds of (n, d) were also confirmed for (n, d) whenever d≤5, leaving the general case as a conjecture.

Given a convex function u, defined in an open bounded convex subset Ω of ℝn, we consider the set

where η is a Borel subset of Ω,ρ is nonnegative, and ∂u(x) denotes the subgradient (or subdifferential) of u at x. We prove that Pp(u; η) is a Borel set and its n-dimensional measure is a polynomial of degree n with respect to ρ. The coefficients of this polynomial are nonnegative measures defined on the Borel subsets of Ω. We find an upper bound for the values attained by these measures on the sublevel sets of u. Such a bound depends on the quermassintegrals of the sublevel set and on the Lipschitz constant of u. Finally we prove that one of these measures coincides with the Lebesgue measure of the image under the subgradient map of u.

A number of known estimates of the number of translates, or lattice translates, of a convex body H required to cover a convex body K are obtained as consequences of two simple results.