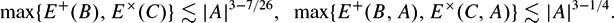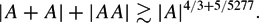Research Article
Cherlin's conjecture for almost simple groups of Lie rank 1
-
- Published online by Cambridge University Press:
- 05 July 2018, pp. 417-435
-
- Article
- Export citation
Two-cocycle twists and Atiyah–Patodi–Singer index theory
-
- Published online by Cambridge University Press:
- 22 August 2018, pp. 437-487
-
- Article
- Export citation
On the uniqueness of cellular injectives
-
- Published online by Cambridge University Press:
- 18 June 2018, pp. 489-504
-
- Article
- Export citation
Growth of quasiconvex subgroups
-
- Published online by Cambridge University Press:
- 28 June 2018, pp. 505-530
-
- Article
- Export citation
An explicit dense universal Hilbert set
-
- Published online by Cambridge University Press:
- 20 June 2018, pp. 531-547
-
- Article
- Export citation
On derived functors of graded local cohomology modules
-
- Published online by Cambridge University Press:
- 10 July 2018, pp. 549-565
-
- Article
- Export citation
An analogue of Khintchine's theorem for self-conformal sets
-
- Published online by Cambridge University Press:
- 03 August 2018, pp. 567-597
-
- Article
- Export citation
On higher energy decompositions and the sum–product phenomenon
-
- Published online by Cambridge University Press:
- 03 July 2018, pp. 599-617
-
- Article
- Export citation
Front Cover (OFC, IFC) and matter
PSP volume 167 issue 3 Cover and Front matter
-
- Published online by Cambridge University Press:
- 04 October 2019, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back Cover (IBC, OBC) and matter
PSP volume 167 issue 3 Cover and Back matter
-
- Published online by Cambridge University Press:
- 04 October 2019, pp. b1-b5
-
- Article
-
- You have access
- Export citation