Article contents
On higher energy decompositions and the sum–product phenomenon
Published online by Cambridge University Press: 03 July 2018
Abstract
Let A ⊂ ℝ be finite. We quantitatively improve the Balog–Wooley decomposition, that is A can be partitioned into sets B and C such that
We use similar decompositions to improve upon various sum–product estimates. For instance, we show
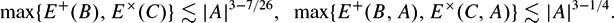 $
\begin{equation*}
\max\{E^+(B) , E^{\times}(C)\} \lesssim |A|^{3 - 7/26}, \ \ \max \{E^+(B,A) , E^{\times}(C, A) \}\lesssim |A|^{3 - 1/4}.
\end{equation*}
$
$
\begin{equation*}
\max\{E^+(B) , E^{\times}(C)\} \lesssim |A|^{3 - 7/26}, \ \ \max \{E^+(B,A) , E^{\times}(C, A) \}\lesssim |A|^{3 - 1/4}.
\end{equation*}
$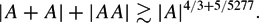 $
\begin{equation*}
|A+A| + |A A| \gtrsim |A|^{4/3 + 5/5277}.
\end{equation*}
$
$
\begin{equation*}
|A+A| + |A A| \gtrsim |A|^{4/3 + 5/5277}.
\end{equation*}
$
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 167 , Issue 3 , November 2019 , pp. 599 - 617
- Copyright
- Copyright © Cambridge Philosophical Society 2018
References
REFERENCES
[BaWo] Balog, A. and Wooley, T.. A low–energy decomposition theorem. Q. J. Math. 68.1 (2017), 207–226.Google Scholar
[BaRo] Balog, A. and Roche–Newton, O.. New sum–product estimates for real and complex numbers, Comput. Geom. 53 (2015), no. 4, 825–846.Google Scholar
[BoCh] Bourgain, J. and Chang, M–C.. On the size of k–fold sum and product sets of integers. J. Amer. Math. Soc. 17 (2) (2004), 473–497.Google Scholar
[Ch] Chang, M–C.. The Erdős–Szemerédi problem on sum set and product set. Ann. of Math. (2) 157 (3) (2003), 939–957.Google Scholar
[CrHa] Croot, E. and Hart, D.. h–fold sums from a set with few products. SIAM J. Discrete Math. 24 (2010), 505–519.Google Scholar
[ElRu] Elekes, G. and Ruzsa, I.. Few sums, many products. Studia Sci. Math. Hungar. 40 (3) (2003), 301–308.Google Scholar
[ErSz] Erdős, P. and Szemerédi, E.. On sums and products of integers. Studies in Pure Math. (Birkhauser, Basel, 1983), 213–218.Google Scholar
[Fo] Ford, K.. Sums and products from a finite set of real numbers. Ramanujan J. 2 (1998), 59–66.Google Scholar
[Ha] Hanson, B.. Estimates for character sums with various convolutions. Preprint (2015) arXiv:1509.04354.Google Scholar
[KaKo] Katz, N. H. and Koester, P.. On additive doubling and energy. SIAM Journal on Discrete Mathematics 24 (2010), 1684–1693.Google Scholar
[IRR] Iosevich, A., Roche–Newton, O. and Rudnev, M.. On discrete values of bilinear forms. arXiv: 1512.02670 (2015).Google Scholar
[KoRu] Konyagin, S. and Rudnev, M.. On new sum–product type estimates. SIAM J. Discrete Math. 27 (2) (2013), 973–990.Google Scholar
[KoSh1] Konyagin, S. and Shkredov, I.. On sum sets of sets, having small product set. Proc. Steklov Inst. Math. 290 (2015), 288–299.Google Scholar
[KoSh2] Konyagin, S. and Shkredov, I.. New results on sum–products in R. Trans. Steklov Math. Inst. 294 (2016), 87–98.Google Scholar
[LiRo] Li, L. and Roche–Newton, O.. Convexity and a sum–product estimate. Acta Arith. 156 (2012), 247–255.Google Scholar
[LiSh] Li, L. and Shen, J.. A sum–division estimate of reals. Proc. Amer. Math. Soc. 138 (1) (2010), 101–104.Google Scholar
[MRS1] Murphy, B., Roche–Newton, O. and Shkredov, I.. Variations of the sum–product problem, arXiv: 1312.6438 (2014).Google Scholar
[MRS2] Murphy, B., Roche–Newton, O. and Shkredov, I.. Variations of the sum–product problem II, arXiv: 1703.09549 (2017).Google Scholar
[MRSS] Murphy, B., Rudnev, M., Shkredov, I. and Shteinikov, Y.. On the few products, many sums problem. arXiv: 1712.0041v1 (2017).Google Scholar
[Na] Nathanson, M.. On sums and products of integers. Proc. Amer. Math. Soc. 125 (1997), 9–16.Google Scholar
[RRSS] Roche–Newton, O., Ruzsa, I., Shen, C. and Shkredov, I.. On the size of the set $AA+A$. arXiv: 1801.1043v1 (2018).Google Scholar
[RSS] Rudnev, M., Shkredov, I. and Stevens, S.. On an energy variant of the sum–product conjecture. arXiv: 1607.05053 (2016).Google Scholar
[ScSh] Schoen, T. and Shkredov, I.. On sumsets of convex sets. Comb. Probab. Comput. 20 (2011), 793–798.Google Scholar
[Sha] Shakan, G.. Konyagin–Shkredov Clustering. Blog Post. Mar. 10 (2018). https://gshakan.wordpress.com/konyagin-shkredov-clustering/.Google Scholar
[She] Sheffer, A.. Konyagain–Shkredov sum–product bound. Blog Post. July 9 (2016). https://adamsheffer.files.wordpress.com/2016/07/ks-sp.pdf.Google Scholar
[Sh1] Shkredov, I.. On sums of Szemerédi–Trotter sets. Proc. Steklov Inst. Math. 289 (1) (2015), 300–309.Google Scholar
[Sh2] Shkredov, I. D.. Some remarks on the Balog–Wooley decomposition theorem and quantities D +, D ×. Proc. Steklov Inst. Math. Accepted, arXiv:1605.00266v1 (2016).Google Scholar
[Sh3] Shkredov, I.. Some new results on higher energies. Trans. MMS 74 (1) (2013), 35–73.Google Scholar
[So1] Solymosi, J.. On the number of sums and products. Bull. Lond. Math. Soc. 37 (4) (2005), 491–494.Google Scholar
[So2] Solymosi, J.. Bounding multiplicative energy by the sumset. Adv. Math. 222 (2) (2009), 402–408.Google Scholar
- 12
- Cited by


