No CrossRef data available.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Published online by Cambridge University Press: 24 October 2008
The object of this paper is to obtain the general solution to the self-adjoint partial differential equation in n dimensions
where pij, q and ρ and bounded, continuous functions of (x1,…, xn) in a domain D and on its boundary, and where ∑pijXiXj≥0 for all (x1,…, xn) of D and all X1,…, Xn. The domain D is an n-dimensional domain and may be either the whole or part of a Riemann surface space of n dimensions. Its boundary is to consist of any number, zero, finite or enumerable, of continuous continua of n − 1 dimensions. These terms will be explained in paragraph II. The solution u = u(x1,…, xn; t) will be valid for (x1,…, xn) in D and t ≥ 0, and will satisfy boundary conditions of the type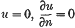 
or similar, these conditions becoming identical at any part of the boundary of D that lies at infinity.
* Hellinger, E., Journ. f. Math. 136 (1909), 210–271Google Scholar; Hahn, H., Monats. Math. Phys. 23 (1912), 161–224CrossRefGoogle Scholar; Hyslop, J., Proc. London Math. Soc. (2), 24 (1926), 264–304.CrossRefGoogle Scholar
* Carathéodory, , Math. Ann. 73 (1913), 323–370.CrossRefGoogle Scholar
* The conditions of continuity as used in a former paper are not bounded (Steen, , Proc. Camb. Phil. Soc. 28 (1932), 31–32)CrossRefGoogle Scholar, they should therefore be altered to the above form.
* See Steen, , Proc. Camb. Phil. Soc. 28 (1932), 27–29.CrossRefGoogle Scholar
* I am indebted to Mr Cornock of the General Electric Company for bringing to my notice systems of equations.