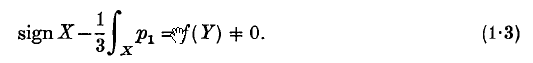Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-23T16:34:56.621Z Has data issue: false hasContentIssue false
  • English
  • Français

Spectral asymmetry and Riemannian Geometry. I

Published online by Cambridge University Press:  24 October 2008

M. F. Atiyah ,
V. K. Patodi  and
I. M. Singer
M. F. Atiyah
Affiliation:
Oxford University Tata Institute for Fundamental Research, Bombay Massachusetts Institute of Technology, Cambridge, Massachusetts
V. K. Patodi
Affiliation:
Oxford University Tata Institute for Fundamental Research, Bombay Massachusetts Institute of Technology, Cambridge, Massachusetts
I. M. Singer
Affiliation:
Oxford University Tata Institute for Fundamental Research, Bombay Massachusetts Institute of Technology, Cambridge, Massachusetts
Get access Rights & Permissions [Opens in a new window]

Extract

1. Introduction. The main purpose of this paper is to present a generalization of Hirzebruch's signature theorem for the case of manifolds with boundary. Our result is in the framework of Riemannian geometry and can be viewed as analogous to the Gauss–Bonnet theorem for manifolds with boundary, although there is a very significant difference between the two cases which is, in a sense, the central topic of the paper. To explain this difference let us begin by recalling that the classical Gauss–Bonnet theorem for a surface X with boundary Y asserts that the Euler characteristic E(X) is given by a formula:

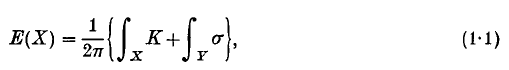
where K is the Gauss curvature of X and σ is the geodesic curvature of Y in X. In particular if, near the boundary, X is isometric to the product Y x R+, the boundary integral in (1.1) vanishes and the formula is the same as for closed surfaces. Similar remarks hold in higher dimensions. Now if X is a closed oriented Riemannian manifold of dimension 4, there is another formula relating cohomological invariants with curvature in addition to the Gauss–Bonnet formula. This expresses the signature of the quadratic form on H2(X, R) by an integral formula

where p1 is the differential 4-form representing the first Pontrjagin class and is given in terms of the curvature matrix R by p1 = (2π)−2Tr R2. It is natural to ask if (1.2) continues to hold for manifolds with boundary, provided the metric is a product near the boundary. Simple examples show that this is false, so that in general

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 77 , Issue 1 , January 1975 , pp. 43 - 69
Copyright
Copyright © Cambridge Philosophical Society 1975

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Atiyah, M. F. and Bott, R. The index theorem for manifolds with boundary. Differential Analysis (Bombay Colloquium), (Oxford 1964).Google Scholar
(2)Atiyah, M. F., Bott, R. and Patodi, V. K.On the heat equation and the index theorem. Invent. Math. 19 (1973), 279–330.CrossRefGoogle Scholar
(3)Atiyah, M. F., Bott, R. and Shapiro, A.Clifford modules. Topology 3 (Suppl. 1) 1964, 3–38.CrossRefGoogle Scholar
(4)Atiyah, M. F. and Singer, I. M.The index of elliptic operators V. Ann. of Math. 93 (1971), 139–149.Google Scholar
(5)Atiyah, M. F., Patodi, V. K. and Singer, I. M.Spectral asymmetry and Riemannian geometry. Bull. London Math. Soc. 5 (1973), 229–234.CrossRefGoogle Scholar
(6)Carslaw, H. S. and Jaeger, J. C.Conduction of Heat in Solids, second edition (Oxford 1959).Google Scholar
(7)Chern, S. S. and Simons, J.Some cohomology classes in principal fibre bundles and their application to Riemannian geometry. Proc. Nat. Acad. Sci. U.S.A. 68 (1971), 791–794.CrossRefGoogle ScholarPubMed
(8)Connor, P. E.The Neumann problem for differential forms on Riemannian manifolds. Mem. Amer. Math. Soc. 20 (1956).Google Scholar
(9)De Rham, G.Variétés Différentiables. Hermann (Paris, 1960.)Google Scholar
(10)Hirzebruch, F.Hilbert modular surfaces. Enseignement Math. 19 (1973), 183–281.Google Scholar
(11)Hörmander, L.Pseudo-differential operators and non-elliptic boundary problems. Ann of Math. 83 (1966), 129–209.CrossRefGoogle Scholar
(12)Palais, R.Seminar on the Atiyah–Singer index theorem. Ann. of Math. Study 57 (Princeton, 1965).Google Scholar
(13)Patodi, V. K.Curvature and the eigenforms of the Laplace operator. J. Diff. Geometry 5 (1971), 233–249.Google Scholar
(14)Ray, D. and Singer, I. M.R-Torsion and the Laplacian on Riemannian manifolds. Advances in Math. 7 (1971), 145–210.CrossRefGoogle Scholar
(15)Ray, D. and Singer, I. M.Analytic torsion for complex manifolds. Ann. of Math. 98 (1973), 154–177.CrossRefGoogle Scholar
(16)Seeley, R.Complex powers of an elliptic operator. Proc. Sympos. Pure Math. 10, Amer. Math. Soc. (1971), 288–307.CrossRefGoogle Scholar
(17)Stong, R.Notes on Cobordism Theory (Princeton, 1968).Google Scholar